Answer
413.4k+ views
Hint:
Proceed with the basic definition of given trigonometric ratios and from that we are able to get the idea about the sides of the triangle as if two sides are known then the third side can be calculated using Pythagoras theorem. Hence, knowing the length of all three sides we can obtain various other trigonometric ratios. Also, considering the sign conventions that in the first quadrant all are positive, in the second quadrant sine, cosecant are positive, in third quadrant tangent and cotangent are positive and in the fourth quadrant cosine and secant are positive. And hence considering all these we can obtain our required answer.
Complete step by step solution:
As the given trigonometric ratio is \[\sin x = \dfrac{3}{5}\]
And so in second quadrant we know that only sine and cosecant are positive while all other trigonometric ratios are negative.
Hence, now making triangle in order to get lengths of all sides as,
Diagram:
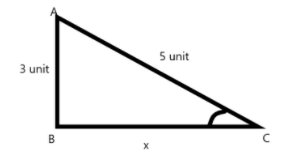
Using Pythagoras theorem calculating the value of x,
Using \[AC = \sqrt {A{B^2} + B{C^2}} \]
On squaring both sides \[A{C^2} = A{B^2} + B{C^2}\]
Now putting the length of side in above equation we can calculate x as,
\[ \Rightarrow \]\[{(5)^2} = {x^2} + {(3)^2}\]
On solving above equation we get,
\[ \Rightarrow \]\[{(x)^2} = 25 - 9\]
On simplifying we get,
\[ \Rightarrow \]\[{(x)^2} = 16\]
Hence, on taking square root,
\[ \Rightarrow \]\[x = 4\,unit\]
Now, as all the side lengths are known we can calculate all trigonometric ratios using proper convention as,
\[ \Rightarrow \]\[\cos x = \dfrac{{adj.}}{{hypo.}} = - \dfrac{4}{5}\]
On calculating other ratio as,
\[ \Rightarrow \]\[\tan x = \dfrac{{opp.}}{{adj.}} = - \dfrac{3}{4}\]
As we know that cosecant, secant and cotangent are inverse of sine, cosine and tangent hence on taking their ratios we get,
\[ \Rightarrow \]\[\cos ecx = \dfrac{{hypo.}}{{opp.}} = \dfrac{5}{3}\]
On calculating another ratio we get,
\[ \Rightarrow \]\[secx = \dfrac{{hypo.}}{{adj.}} = - \dfrac{5}{4}\]
And calculating another ratio we get,
\[ \Rightarrow \]\[\cot x = \dfrac{{adj.}}{{opp.}} = - \dfrac{4}{3}\]
Hence, all the required ratios are calculated.
Note:
There are six trigonometric ratios, sine, cosine, tangent, cosecant, secant and cotangent. These six trigonometric ratios are abbreviated as sin, cos, tan, csc, sec, cot. These are referred to as ratios since they can be expressed in terms of the sides of a right-angled triangle for a specific angle x.
Apply Pythagoras theorem carefully and also draw and calculate the triangle and sides of the triangle without any mistake.
In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others.
Proceed with the basic definition of given trigonometric ratios and from that we are able to get the idea about the sides of the triangle as if two sides are known then the third side can be calculated using Pythagoras theorem. Hence, knowing the length of all three sides we can obtain various other trigonometric ratios. Also, considering the sign conventions that in the first quadrant all are positive, in the second quadrant sine, cosecant are positive, in third quadrant tangent and cotangent are positive and in the fourth quadrant cosine and secant are positive. And hence considering all these we can obtain our required answer.
Complete step by step solution:
As the given trigonometric ratio is \[\sin x = \dfrac{3}{5}\]
And so in second quadrant we know that only sine and cosecant are positive while all other trigonometric ratios are negative.
Hence, now making triangle in order to get lengths of all sides as,
Diagram:

Using Pythagoras theorem calculating the value of x,
Using \[AC = \sqrt {A{B^2} + B{C^2}} \]
On squaring both sides \[A{C^2} = A{B^2} + B{C^2}\]
Now putting the length of side in above equation we can calculate x as,
\[ \Rightarrow \]\[{(5)^2} = {x^2} + {(3)^2}\]
On solving above equation we get,
\[ \Rightarrow \]\[{(x)^2} = 25 - 9\]
On simplifying we get,
\[ \Rightarrow \]\[{(x)^2} = 16\]
Hence, on taking square root,
\[ \Rightarrow \]\[x = 4\,unit\]
Now, as all the side lengths are known we can calculate all trigonometric ratios using proper convention as,
\[ \Rightarrow \]\[\cos x = \dfrac{{adj.}}{{hypo.}} = - \dfrac{4}{5}\]
On calculating other ratio as,
\[ \Rightarrow \]\[\tan x = \dfrac{{opp.}}{{adj.}} = - \dfrac{3}{4}\]
As we know that cosecant, secant and cotangent are inverse of sine, cosine and tangent hence on taking their ratios we get,
\[ \Rightarrow \]\[\cos ecx = \dfrac{{hypo.}}{{opp.}} = \dfrac{5}{3}\]
On calculating another ratio we get,
\[ \Rightarrow \]\[secx = \dfrac{{hypo.}}{{adj.}} = - \dfrac{5}{4}\]
And calculating another ratio we get,
\[ \Rightarrow \]\[\cot x = \dfrac{{adj.}}{{opp.}} = - \dfrac{4}{3}\]
Hence, all the required ratios are calculated.
Note:
There are six trigonometric ratios, sine, cosine, tangent, cosecant, secant and cotangent. These six trigonometric ratios are abbreviated as sin, cos, tan, csc, sec, cot. These are referred to as ratios since they can be expressed in terms of the sides of a right-angled triangle for a specific angle x.
Apply Pythagoras theorem carefully and also draw and calculate the triangle and sides of the triangle without any mistake.
In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others.
Watch videos on
Find the values of other five trigonometric ratios;
\[\sin x = \dfrac{3}{5}\], x lies in the second quadrant.
\[\sin x = \dfrac{3}{5}\], x lies in the second quadrant.

Trigonometric Functions NCERT EXERCISE 3.2 (Q.2) | Class 11 Maths | Abhishek Sir
Subscribe
 Share
Share likes
21 Views
9 months ago
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Why Are Noble Gases NonReactive class 11 chemistry CBSE

Let X and Y be the sets of all positive divisors of class 11 maths CBSE

Let x and y be 2 real numbers which satisfy the equations class 11 maths CBSE

Let x 4log 2sqrt 9k 1 + 7 and y dfrac132log 2sqrt5 class 11 maths CBSE

Let x22ax+b20 and x22bx+a20 be two equations Then the class 11 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

At which age domestication of animals started A Neolithic class 11 social science CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Write a letter to the principal requesting him to grant class 10 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Fill in the blanks A 1 lakh ten thousand B 1 million class 9 maths CBSE



 Watch Video
Watch Video