Question

Find the angle subtended at the center of a circle of radius ‘a’ by an arc of length \[\left( \dfrac{a\pi }{4} \right)\]cm.

Answer 1

Hint:.The arc is any portion of the circumference of a circle. Arc length is the distance from one endpoint of the arc to the other point. Use an equation to find arc length \[=2\pi r\left( \dfrac{\theta }{360} \right)\].

Complete step-by-step answer:
Given that the radius of the circle = a
We need to find the angle subtended by arc length \[\left( \dfrac{a\pi }{4} \right)\]

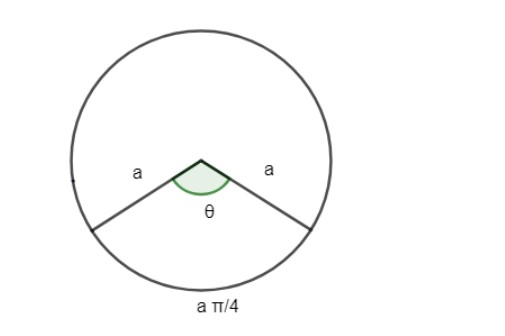
From the figure it is clear that the length of arc is\[\Rightarrow \dfrac{a\pi }{4}\]
We need to find \[\theta \].
The formula for finding the arc length is given by
\[\Rightarrow \]arc length\[=2\pi r\left( \dfrac{\theta }{360} \right)\]
We can find the arc length or portion of the arc in the circumference, if we know at what portion of 360 degrees the arc’s central angle is.
Arc length\[=2\pi r\left( \dfrac{\theta }{360} \right)\], where r is the radius of the circle.
\[\therefore 2\pi r\left( \dfrac{\theta }{360} \right)=\dfrac{a\pi }{4}\]
We have been given arc length\[=\dfrac{a\pi }{4}\]
Put radius, r = a
\[\Rightarrow 2\pi a\left( \dfrac{\theta }{360} \right)=\dfrac{a\pi }{4}\]
Simplifying the above equation,
\[\begin{align}
  & 2\pi a\left( \dfrac{\theta }{360} \right)=\dfrac{a}{4}\pi \\
 & \Rightarrow \dfrac{\theta }{360}=\dfrac{1}{8}\Rightarrow \theta =\dfrac{360}{8}={{45}^{\circ }} \\
\end{align}\]
\[\therefore \]We get the angle subtended at the center of the circle\[={{45}^{\circ }}\]

Note: Here, arc length \[=\dfrac{a\pi }{4}\]
If we assume value of \[\theta ={{45}^{\circ }}\]and applying we get
\[\begin{align}
  & =2\pi a\left( \dfrac{45}{360} \right) \\
 & =2\pi a\left( \dfrac{1}{8} \right)=\dfrac{a\pi }{4} \\
\end{align}\]