Answer
416.1k+ views
Hint: In this question, we need to start with the diagram of Young’s double-slit experiment. Then by using the coordinate geometry and approximation we get the path difference as $\vartriangle x = d\dfrac{y}{D}$ but we already know the equation of the path difference as \[\vartriangle x = n\lambda \] to get a bright fringe and $\Delta x = \left( {n + \dfrac{1}{2}} \right) \times \lambda $ to get a dark fringe pattern. So now equating these equations we get we will get the position of dark and bright fringe, then we subtract the bright fringe from the dark fringe for the same value of n, and we get $width = \dfrac{{D\lambda }}{d}$
Complete Step-by-Step solution:
First, we draw the diagram of interference using the double-slit experiment as shown in figure 1.
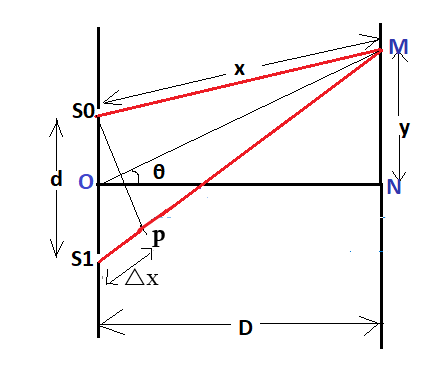
Here \[{S_1}\] and \[{S_0}\] are the slits which are $d$ distance apart.
$D$ is the distance between the screen and the slit.
Also $D \gg d$
Now we know the expression for the path difference is
\[\vartriangle x = n\lambda \] To get bright fringe ------------------ (1)
And $\Delta x = \left( {n + \dfrac{1}{2}} \right) \times \lambda $ to get a dark fringe---------- (2)
Now we assume that \[{S_1}M\] and \[{S_0}M\] are approximately parallel and hence \[{S_0}P\] is approximately perpendicular to \[{S_1}M\],\[{S_0}M\], and \[OM\].
Also as \[{S_0}P \bot OM\] and \[{S_1}{S_0} \bot ON\] therefore
\[\angle {S_0}{S_1}P = \angle MON = \theta \]
Now we can write the path difference as
$\vartriangle x = {S_1}M - {S_0}M \approx {S_1}M - PM$
$ \Rightarrow \vartriangle x = {S_1}P = d\sin \theta \approx d\tan \theta $
$ \Rightarrow \vartriangle x = d\dfrac{y}{D}$------------------------ (3)
We can see from the diagram that at a distance y from the center of the screen the center of the bright fringe is obtained. Now equating equation (1) and (3) we get
$ \Rightarrow \vartriangle x = n\lambda = d\dfrac{y}{D}$
$ \Rightarrow y = n\dfrac{{D\lambda }}{d}$
By putting $n = 1,2,3....$ we will get different positions of bright fringe that is
$ \Rightarrow y = 0, \pm 1\dfrac{{D\lambda }}{d}, \pm 2\dfrac{{D\lambda }}{d}, \pm 3\dfrac{{D\lambda }}{d}...$
And similarly, for dark fringe we will get
$ \Rightarrow y = \left( {n + \dfrac{1}{2}} \right)\dfrac{{D\lambda }}{d} = \pm 1\dfrac{{D\lambda }}{d}, \pm 3\dfrac{{D\lambda }}{d}, \pm 5\dfrac{{D\lambda }}{d}...$
Now to calculate the width we subtract the bright fringe from the dark fringe for the same value of n, and we get
$width = \dfrac{{D\lambda }}{d}$
B. It can be seen from the above equation that as the distance between the two slits is increasing and that d is increasing, the fringe width is reducing. If d becomes much higher than x, the fringe width will become quite small. Then the maxima and minima will become such closely spaced that it seems like a uniform intensity pattern on the screen. This result is an outcome of a simple theory that the effect of a wave is hard to observe if the wavelength is small in comparison to the dimensions of the obstacles or slits in the path of the incident wavefront.
Note: For these types of questions, we first need to remember Young’s double-slit experiment and the equations for path difference and positions of occurrence of the bright and dark fringes. After that, we need to have a clear understanding of how the interference gets affected when the distance between the two slits is increased or distance between the screen and the slits increases.
Complete Step-by-Step solution:
First, we draw the diagram of interference using the double-slit experiment as shown in figure 1.

Figure 1
Here \[{S_1}\] and \[{S_0}\] are the slits which are $d$ distance apart.
$D$ is the distance between the screen and the slit.
Also $D \gg d$
Now we know the expression for the path difference is
\[\vartriangle x = n\lambda \] To get bright fringe ------------------ (1)
And $\Delta x = \left( {n + \dfrac{1}{2}} \right) \times \lambda $ to get a dark fringe---------- (2)
Now we assume that \[{S_1}M\] and \[{S_0}M\] are approximately parallel and hence \[{S_0}P\] is approximately perpendicular to \[{S_1}M\],\[{S_0}M\], and \[OM\].
Also as \[{S_0}P \bot OM\] and \[{S_1}{S_0} \bot ON\] therefore
\[\angle {S_0}{S_1}P = \angle MON = \theta \]
Now we can write the path difference as
$\vartriangle x = {S_1}M - {S_0}M \approx {S_1}M - PM$
$ \Rightarrow \vartriangle x = {S_1}P = d\sin \theta \approx d\tan \theta $
$ \Rightarrow \vartriangle x = d\dfrac{y}{D}$------------------------ (3)
We can see from the diagram that at a distance y from the center of the screen the center of the bright fringe is obtained. Now equating equation (1) and (3) we get
$ \Rightarrow \vartriangle x = n\lambda = d\dfrac{y}{D}$
$ \Rightarrow y = n\dfrac{{D\lambda }}{d}$
By putting $n = 1,2,3....$ we will get different positions of bright fringe that is
$ \Rightarrow y = 0, \pm 1\dfrac{{D\lambda }}{d}, \pm 2\dfrac{{D\lambda }}{d}, \pm 3\dfrac{{D\lambda }}{d}...$
And similarly, for dark fringe we will get
$ \Rightarrow y = \left( {n + \dfrac{1}{2}} \right)\dfrac{{D\lambda }}{d} = \pm 1\dfrac{{D\lambda }}{d}, \pm 3\dfrac{{D\lambda }}{d}, \pm 5\dfrac{{D\lambda }}{d}...$
Now to calculate the width we subtract the bright fringe from the dark fringe for the same value of n, and we get
$width = \dfrac{{D\lambda }}{d}$
B. It can be seen from the above equation that as the distance between the two slits is increasing and that d is increasing, the fringe width is reducing. If d becomes much higher than x, the fringe width will become quite small. Then the maxima and minima will become such closely spaced that it seems like a uniform intensity pattern on the screen. This result is an outcome of a simple theory that the effect of a wave is hard to observe if the wavelength is small in comparison to the dimensions of the obstacles or slits in the path of the incident wavefront.
Note: For these types of questions, we first need to remember Young’s double-slit experiment and the equations for path difference and positions of occurrence of the bright and dark fringes. After that, we need to have a clear understanding of how the interference gets affected when the distance between the two slits is increased or distance between the screen and the slits increases.
Recently Updated Pages
Assertion The resistivity of a semiconductor increases class 13 physics CBSE

Three beakers labelled as A B and C each containing 25 mL of water were taken A small amount of NaOH anhydrous CuSO4 and NaCl were added to the beakers A B and C respectively It was observed that there was an increase in the temperature of the solutions contained in beakers A and B whereas in case of beaker C the temperature of the solution falls Which one of the following statements isarecorrect i In beakers A and B exothermic process has occurred ii In beakers A and B endothermic process has occurred iii In beaker C exothermic process has occurred iv In beaker C endothermic process has occurred

The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the stopping potential when the metal with class 12 physics JEE_Main

The momentum of a photon is 2 times 10 16gm cmsec Its class 12 physics JEE_Main

Trending doubts
Draw a diagram showing the external features of fish class 11 biology CBSE

Select the word that is correctly spelled a Twelveth class 10 english CBSE

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

What is BLO What is the full form of BLO class 8 social science CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Change the following sentences into negative and interrogative class 10 english CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE



