




What is Zero Polynomial?
A polynomial is an algebraic phrase with one or more terms, as we are previously aware. The real values of the variable for which the value of the polynomial becomes 0 are known as polynomial zeroes. Therefore, if $p(m) = 0$ and $p(n) = 0$, then the real numbers 'm' and 'n' are zeroes of the polynomial p(x). A polynomial with the value zero (0) is referred to as a zero polynomial. The greatest power of the variable x in the polynomial ${ax}^2+bx+c=0$ is a polynomial's degree. A degree 1 polynomial is referred to as a linear polynomial.
What are Zero Polynomials?
Any real value of x for which the polynomial's value becomes 0 is defined as the polynomial's zero. If p(k) = 0, then a real integer k is the zero of the polynomial p(x).
Geometrical Meaning of the Zero Polynomial
The x-coordinate of the place where the graph intersects the x-axis serves as the polynomial zero. When a polynomial $p(x$) meets the x-axis at the coordinates $(k, 0), k$ is the polynomial's zero.
At most one point, the graph of a linear polynomial crosses the x-axis.
A quadratic polynomial graph can intersect the x-axis up to two times. The graph in this instance has a parabola-like form.
A quadratic polynomial might contain two separate zeros, two equal zeroes, or no zero geometrically.
A cubic polynomial graph can cross the x-axis a maximum of three times. There can be a maximum of three zeros in a cubic polynomial.
An nth-degree polynomial typically crosses the x-axis a maximum of $n$ times. A polynomial of the nth degree can only have n zeroes at most.
Degree of a Polynomial
The degree of a polynomial is determined by the variable term's highest exponential power. Let’s discuss some types of polynomials based on degree:
A linear polynomial is a polynomial with a degree of $1$. $ax + b$, where $a$ and $b$ are real numbers and are not equal $0$. A linear polynomial is $2x + 3$.
A degree two polynomial is referred to as a quadratic polynomial. A quadratic polynomial has the standard form of $ax^2 + bx + c$, where $a, b$, and $c$ are All real numbers, and a not equal zero, $x^2+ 3x + 4$ is an example.
A cubic polynomial is a three-degree polynomial. The formula for standard form is $ax^3+ bx^2+ cx+ d$, where $a, b, c$, and $d$ are all real integers and not equal to zero. An illustration $x^3+ x^2$.
Representing Zero Polynomial on Graph
A graph spanning the coordinate axis can show a polynomial expression of the form $y = f(x)$. On the x-axis is displayed the value of $x$, and on the y-axis is displayed the value of $f(x)$ or $y$. Depending on the degree of the polynomials, the polynomial expression may take the form of a linear expression, quadratic expression, or cubic expression.
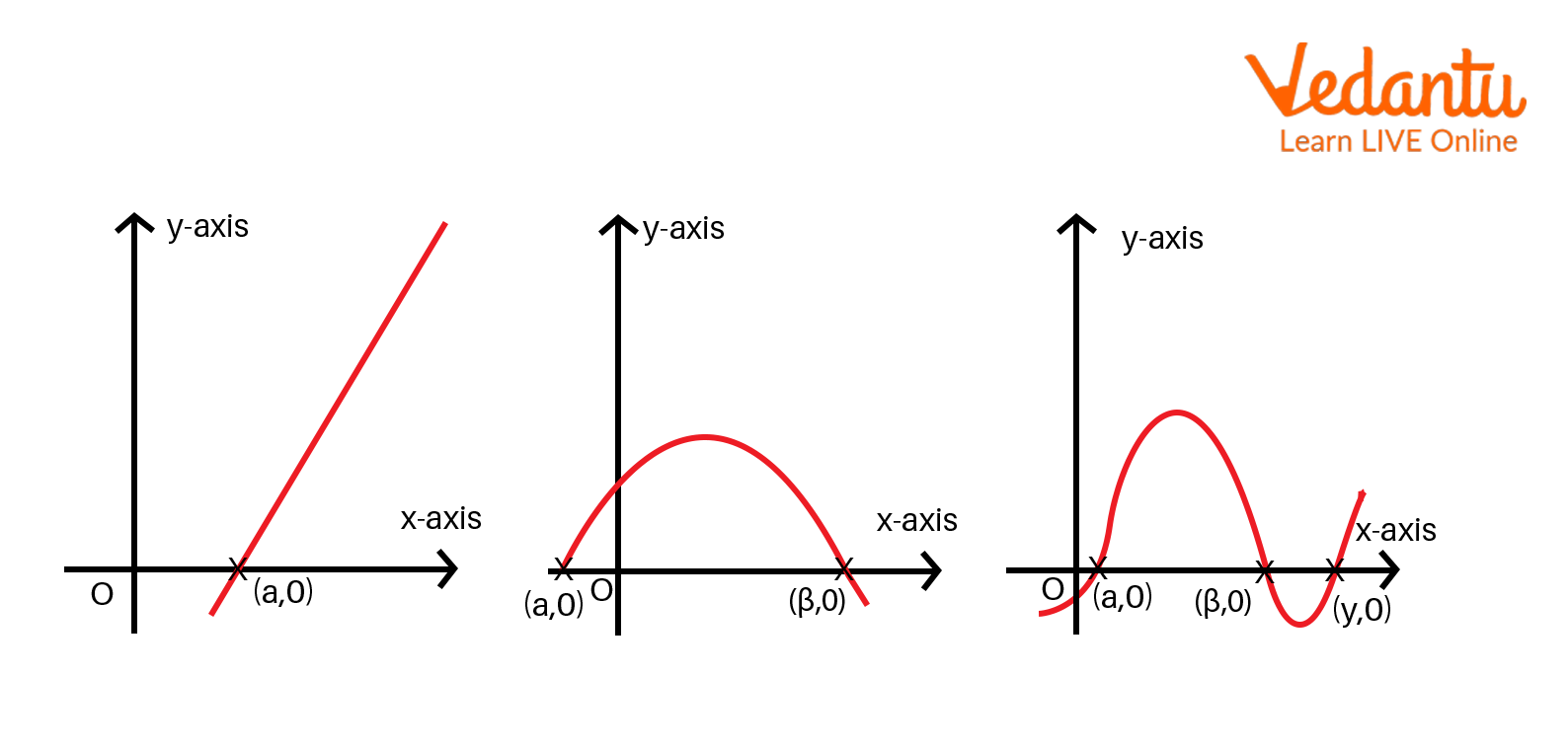
Graph of Zero Polynomial
By looking at the places on the graph where the graph line intersects the x-axis, one can determine a polynomial's zeros.
Solved Examples
Example 1: What is the value of ‘a’ when the degree of the polynomial, $x^3 + x^{a-4} + x^2 + 1$, is $4$?
Solution: The highest power of $x$ in a polynomial $P(x)$ is called the degree $(x)$.
therefore , $x^{a-4} = x^4$
$a-4 = 4, a = 4+4 =8$
Hence, the value of a comes out to be $8$.
Example 2: Sam is aware that a quadratic polynomial has zeros of -3 and 5. How can we assist in deriving the polynomial equation?
Solution: The given zeros of the quadratic polynomial are $-3$ and $5$.
Consider $\alpha = -3$, and $\beta = 5$
Then, calculate the sum of the roots $= α + \beta = 2$
Product of the roots $= \alpha.\beta = -15$
Since, the required quadratic equation is $x^2 - (\alpha + \beta)x + \alpha.\beta = 0$
Put the values of the zeros in the equation above
$ - 2(x) + (-15) = 0$
Hence, $x^2 - 2x - 15 = 0$ is the required equation.
Practice Questions
1. Find the polynomial with the values -2 and -3 for the zeros.
$x^2-5x-6=0$
$x^2+6x+5=0$
$x^2+5x+6=0$
$x^2-5x+6=0$
Answer: C
2. A polynomial's zeros are also known as the equation's____
Variables
Roots
Constants
Answer: D
Summary
Let's review what we learnt from this article. All x-values that reduce a polynomial, p(x), to zero are considered zeros. They are intriguing to us for a variety of reasons, one of which is because they show the graph's x-intercepts for the polynomial. Their relationship to the polynomial factors is direct. This article discussed the geometric meaning of a polynomial's zeros and how to find them. For you to better comprehend this idea, we have included practice problems and examples with answers that have been solved.
FAQs on Zero Polynomial
1. What connection exists between a quadratic polynomial's coefficients and its sum of zeros?
The negative of the coefficient of $x$ by the coefficient of $x^2$ is equal to the sum of the zeroes in a quadratic polynomial. The constant term divided by the coefficient of $x^2$ is equal to the product of the zeroes. The sum and Product of Zeros of a quadratic equation are as follows:
Sum of zeroes, $\alpha+\beta$ is $\dfrac{-b}{a}$
Product of zeroes, $\alpha \beta$ is $\dfrac{c}{a}$.
2. How can we find the complex zeros of a polynomial function?
The complex number formula, $i^2 = -1$, can be used to compute the complex zeros of polynomials. Using the value of I from complex numbers, the negative roots can also be made simpler. Finding the square root of a negative number is impossible for the equation ${(x + 3)}^2$ = -25. Here, we write ${(x + 3)}^2$ = 25$i^2$ using the formula $i^2$ = -1. After simplifying, we get (x + 3) = + 5i, and the polynomial's zeros are -3 + 5i and -3 -5i.
3. Do all polynomials include a zero?
There could be zero, one, or several zeros in a polynomial function. All positive, odd-order polynomial functions have at least one zero, whereas positive, even-order polynomial functions might not. Any polynomial of positive order, regardless of odd or even, can have a maximum number of zeros equal to its order.


























