Value of Tan 180

Sine, cosine, tangent, cosecant, secant, and cotangent are the six trigonometric functions. There are numerous identities involving these six trigonometric functions that are used to solve many mathematical problems, and they are all related to one another in some way.

Sine, cosine, and tangent are the three fundamental among them because we can derive the other three using these fundamental functions. Secant is the reciprocal of cosine, cotangent is the reciprocal of a tangent, and cosecant is the reciprocal of sine. While tangent and cotangent have a periodicity of $\pi$, sine, cosine, secant, and cosecant have a periodicity of $2\pi$.

What is the Value of tan 180?

In Trigonometry, the measurement of tan 180 degrees equals 0. The \[\tan{180}^{\circ}\] can also be represented by 3.14159, which is the spherical geometry equivalent to the specific angle of 180 degrees.

How to Calculate Tan Value at 180 Degrees?

In this section, we will learn methods to find the value \[\tan{180}^{\circ}\].

The method for determining $\tan 180$ degree is as follows:

1. Making Use of Trigonometric Functions

2. Using Unit Circle

Value of Tan 180 Degrees Using Unit Circle

The \[\tan{180}^{\circ}\] can be expressed as $\tan \pi$ in a circular notation.

We already know that the tan can be written as \[\dfrac{\text{sin}}{\text{cos}}\]. The tan 180 value can be obtained from the unit circle using this relationship.

We can calculate the tangent values for each degree for a unit circle with a radius of 1. We can determine all the trigonometric ratios and values by using a unit circle drawn on the XY plane.

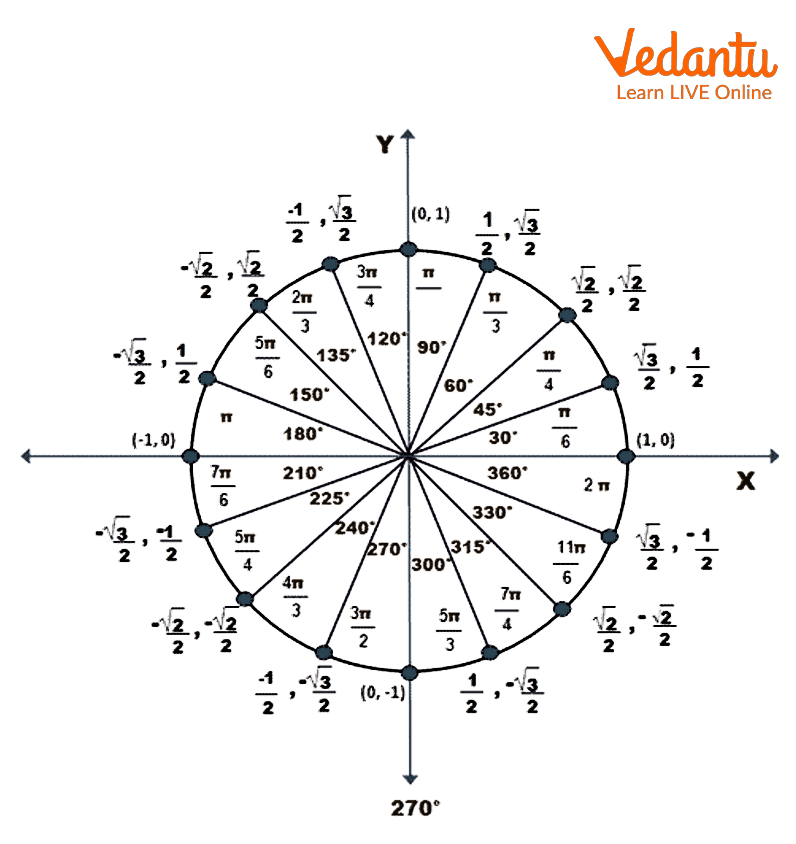
Value of Tan 180 Degrees on the Unit Circle

We know,

\[\sin 180^0=0\] and \[\cos 180^0 = -1\]

Therefore, \[\tan 180^0 = \dfrac{\sin 180^0}{\cos 180^0}\]

$=\dfrac{0}{-1}=0$

Value of Tan 180 Degrees Using Trigonometric Functions

In trigonometric ratios of angles \[(180\cos 180^{\circ}- \theta)\], we will find the relation between all six trigonometric ratios.

We know that

\[\sin (90^0 + \theta) = \cos \theta\]

\[\cos (90^0 + \theta) = - \sin \theta\]

\[\tan (90^0 + \theta) = - \cot \theta\]

\[\csc (90^0 + \theta) = \sec \theta\]

\[\sec ( 90^0 + \theta) = - \csc \theta\]

\[\cot ( 90^0 + \theta) = - \tan \theta\]

and

\[\sin (90^0 - \theta) = \cos \theta\]

\[\cos (90^0 - \theta) = \sin \theta\]

\[\tan (90^0 - \theta) = \cot \theta\]

\[\csc (90^0 - \theta) = \sec \theta\]

\[\sec (90^0 - \theta) = \csc \theta\]

\[\cot (90^0 - \theta) = \tan \theta\]

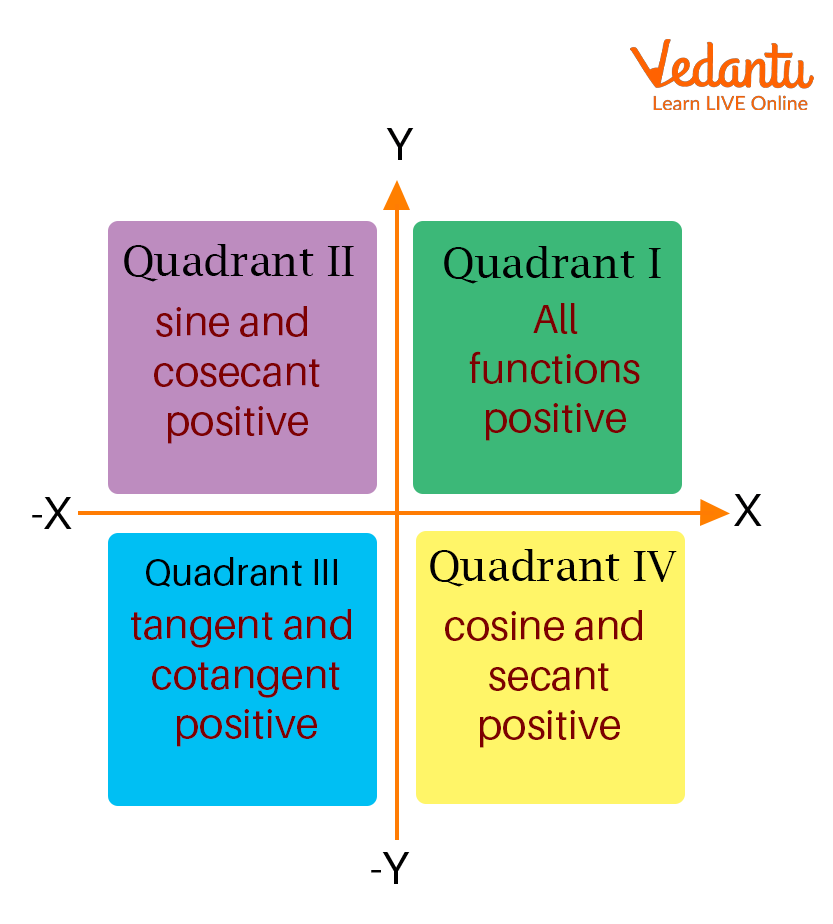
Trigonometric Ratios in Quadrants

Now, \[180^0\] can be mathematically defined as \[tan(90^0 + 90^0)\].

According to the above image, \[tan(90^0 + A)\] is located in the second quadrant, so the tan is positive.

\[= – \cot 90^0\]

\[= –\dfrac{(\cos 90^0)} { \sin 90^0)}\]

\[= – \dfrac{0}{1}\]

\[= 0\]

Consequently, \[\tan 180^0 = 0\]

Alternately, \[\tan 180^0\] can be calculated as:

\[\tan 180^0 = \tan(180^0 – 0) = – \tan 0^0 = 0\]

Solved Examples

Example 1: Find the value of tan 120⁰.

Solution: \[\tan 120^0 = \tan(180^0 – 60^0)\]

\[= –\ tan 60^0\]

$= – \sqrt{3}$

So, the value of $\tan 120^0$ is $-\sqrt{3}$

Example 2: Evaluate 2tan180⁰ - tan150⁰.

Solution: \[2 \tan180^0 - \tan150^0\]

\[= 2\ tan 180^0 - \tan(180^0 -30^0\]

\[= 2(0) - (-\tan 30^0)\]

\[= 0 + \tan 30^0\]

\[= \dfrac{1}{\sqrt{3}}\]

So, the value of $2\tan180^0 – \tan150^0$ is $= \dfrac{1}{\sqrt{3}}$

Example 3: Find tan180⁰ + cot 45⁰.

Solution: The value of \[\tan 180^0 = 0\] and \[\cot 45^0 = 1\].

Therefore,

\[\tan 180^0 + \cot 45^0\]

$= 0 + 1 = 1$

So, the value of $\tan 180^0 + \cot 45^0$ is 1.

Example 4: Find the value of tan240⁰.

Solution: \[\tan 240^0= tan (180 + 60)^0\]

\[= tan 60^0\]

We know \[\tan (180^{\circ} + \theta) = tan \theta\]

$ = \sqrt{3}$

So, the value of $\tan 240^0$ is $ = \sqrt{3}$

Practice Problem

Q 1. Find sec 150⁰.

Q 2. Find tan 120⁰ + cot 45⁰.

Answer

1. $\dfrac{-2}{\sqrt{3}}$

2. $1-\sqrt{3}$

Conclusion

To summarise, the measurement of tan 180 degrees equals 0. $\tan 180 °$ can also be represented by 3.14159, which is the spherical geometry equivalent to the specific angle of 180 degrees. The cot of an angle is the reciprocal of a tan. We hope this article helped you understand how to find the value of $\tan 180$ degrees. Take out a pencil and piece of paper and try to practise the questions given above in this article by yourself.