Refraction Through a Rectangular Glass Slab

Glasses, lenses, and rainbow formation are all examples of refraction of light. A coin placed at the bottom of the beaker containing water seems to be raised, the formation of mirage and other optical illusions occur due to refraction of light. These are examples of refraction of light. When we partly immerse the pencil in a water containing beaker, it appears to be broken at the interface of air and water. This is the real life example of refraction of light that we can observe. The concept of refraction of light plays an important role in optics.

What Is Refraction of Light?

When a light ray travels from one optical medium to another optical medium, it suffers a change of direction at the surface of separation of the two media. This is known as refraction of light. Refraction occurs due to the change in the speed of light in going from one medium to another medium. When light travels from rarer to denser medium, that means when light travels from air to glass, the refracted ray bends towards the normal. In this case, angle of incidence would be greater than angle of refraction. If light ray travels from denser medium to rarer medium, then it will bend away from the normal. Here, angle of incidence would be less than the angle of refraction.

Laws of Refraction

There are two important laws of refraction which plays an important role in Physics:

• The incident ray, refracted ray and the normal to the interface of two transparent media at the point of incidence, all the three lie in the same plane.

• ^{The ratio of the sine of the angle of incidence to the sine of the angle of refraction is a constant for the light of a given colour and for the pair of media.}

$\dfrac{{\sin i}}{{\sin r}} = constant = R.I$

This law is also known as Snell’s law after the name of its discoverer, Willebrord Snell. This law gives us the relation between the incident and refraction angles and the refractive indices of the mediums.

Refractive Index of the Medium:

Here, the ray of light passing from the medium 1 to the medium 2 is shown in the above figure. Here, we will consider medium of air as medium 1 and medium of glass as medium 2. Let the speed of light in medium 1 is ${v_1}$ and the speed of light in medium 2 is ${v_2}$ Let the refractive index of medium 1 is ${n_1}$ and the refractive index of medium 2 is ${n_2}$

Image:

Refraction of light

The extent to which the refractive medium increases or decreases the speed of light is called the refractive index of the medium. It is the ratio of the speed of light in medium 1 to the speed of medium 2. Mathematically, it is denoted as

${n_{21}} = \dfrac{{{v_1}}}{{{v_2}}}$ … … …(i)

Where, ${n_{21}}$ shows the refractive index of medium 2 with respect to the incident medium 1.

Snell’s law tells us that the ratio of the sine of the angle of incidence to the sine of the angle of refraction is equal to the refractive index of the second medium with respect to the first medium.

$\therefore \dfrac{{\sin i}}{{\sin r}} = {n_{21}}$ … … …(ii)

The term ${n_{21}}$ is constant for light of a given colour and for the given pair of media.

Now, from the equation (i) and (ii),

We can write,

$\dfrac{{\sin i}}{{\sin r}} = {n_{21}} = \dfrac{{{v_1}}}{{{v_2}}}$ … … …(iii)

Another way to understand Snell’s Law:

The absolute refractive index is the ratio of the speed of light in vacuum to the speed of light in a given medium. Mathematically, it is expressed as $n = \dfrac{c}{v}$

So, the absolute refractive index of medium 1 can be denoted by ${n_1} = \dfrac{c}{{{v_1}}}$ and the absolute refractive index of medium 2 can be denoted by ${n_2} = \dfrac{c}{{{v_2}}}$

We can write the last term of equation (iii) as,

\[\dfrac{{{v_1}}}{{{v_2}}} = \dfrac{{\left( {\dfrac{c}{{{v_2}}}} \right)}}{{\left( {\dfrac{c}{{{v_1}}}} \right)}}\]

Where $c$ is the speed of light in vacuum and its value is $3 \times {10^8}\dfrac{m}{s}$

Here, the term $\dfrac{c}{{{v_2}}}$ is the absolute refractive index of the medium 2 and the term $\dfrac{c}{{{v_1}}}$ shows the absolute refractive index of the medium 1. So, we can write, $\dfrac{{{v_1}}}{{{v_2}}} = \dfrac{{{n_2}}}{{{n_1}}} = {n_{21}}$ (from equation (i))

So, from the above discussion, we can write Snell’s law as $\dfrac{{\sin i}}{{\sin r}} = {n_{21}} = \dfrac{{{v_1}}}{{{v_2}}} = \dfrac{{{n_2}}}{{{n_1}}}$

Where, ${n_2}$ and ${n_1}$ are the absolute refractive indices of media 2 and 1 respectively.

From the above discussion, we found that the refractive index of medium 2 with respect to the incident medium 1 is written as ${n_{21}} = \dfrac{{{n_2}}}{{{n_1}}}$

So, in the similar way, the refractive index of glass with respect to water is given by ${n_{gw}} = \dfrac{{{n_g}}}{{{n_w}}}$

Where, ${n_g}$ is the absolute refractive index of glass and ${n_w}$ is the absolute refractive index of water.

Ray Diagram to Understand Refraction of Light Through a Rectangular Glass Slab

Here, we are tracing the path of the ray of light when it travels from the rectangular glass slab.

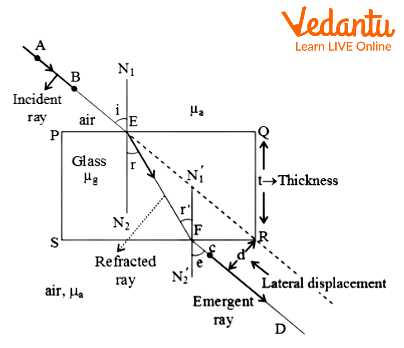
Refraction Through a Rectangular Glass Slab

Here, we have considered an incident ray AE. This incident ray is incident on the surface PQ of the glass slab. Now, if we draw a normal at the point of incidence E, we will find that the ray AE is making an angle ‘$i$’ with the normal. So, here the incident angle would be ‘$i$’.

Now, the ray is incident from an optically rarer to an optically denser medium, that is, from air to glass. So, when light travels from a rarer to a denser medium, the speed of light decreases. As glass is denser compared to air, therefore, in going from air to glass, the ray of light would bend towards the normal to the interface of air and glass. In this way, light changes its path when travelling from rarer to denser medium.

Now, the ray EF represents the refracted ray. Here, the angle that the refracted ray is making with the normal is ‘$r$’. This refracted ray is travelling from surface PQ to surface SR. Now, at surface SR, draw another normal at point F because EF represents the refracted ray which is now incident on the surface SR. So, if we now draw a normal ${N_1}'{N_2}'$ at point F, then we can say that the angle of incidence for this ray at the surface SR is ‘$r'$’.

Now, this ray of light is incident from a denser medium to a rarer medium. Therefore, the light ray will bend away from the normal and it will form an angle ‘e’ with the normal. This angle is known as the angle of emergence. It is a parallel sided glass slab and therefore the incident ray is parallel to the emergent ray.

Lateral Displacement of Light

From the above figure, we can say that the path of the light ray has been shifted. This shift in the path of the light ray is known as the lateral displacement of light. Lateral means sideways. So, we can say that the emergent ray has been shifted from the original path of the incident ray.

Solved Examples

1. Calculate the speed of light in glass. The value of refractive index of glass is 1.5

Ans: Given: Refractive index of glass $n = 1.5$

Here, we will use the formula $n = \dfrac{c}{v}$

Where, $c$ is the speed of light in free space and its value is $c = 3 \times {10^8}\dfrac{m}{s}$ and $v$

is the speed of light in the medium.

So, we will get \[1.5 = \dfrac{{3 \times {{10}^8}}}{v}\]

$ \Rightarrow v = \dfrac{{3 \times {{10}^8}}}{{1.5}} = 2 \times {10^8}\dfrac{m}{s}$

Therefore, the speed of light would be $2 \times {10^8}\dfrac{m}{s}$

2. Refractive indices of glass and water with respect to air are $\dfrac{3}{2}$ and $\dfrac{4}{3}$ . Find the value of the refractive index of glass with respect to water.

Ans: Given: Refractive index of glass ${n_g} = \dfrac{3}{2}$

Refractive index of air ${n_w} = \dfrac{4}{3}$

Now, we need to calculate the refractive index of glass with respect to water.

So, we will use the formula ${n_{gw}} = \dfrac{{{n_g}}}{{{n_w}}}$

$\therefore {n_{gw}} = \dfrac{{(\dfrac{3}{2})}}{{(\dfrac{4}{3})}} = \dfrac{9}{8}$

So, the final answer is $\dfrac{9}{8}$

Interesting Facts

• Stellar Scintillation occurs due to atmospheric refraction of starlight.

• The sun appears to rise 2 minutes before the actual rise and it continues to be seen 2 minutes after it has actually set. Therefore, the day becomes longer by 4 minutes due to atmospheric refraction.

• The human eyes have a lens which refract light onto the retina and we see the surroundings due to refraction.

Conclusion

So, we can conclude that when light travels obliquely from one medium to another medium, it changes its path and we can understand refraction with the help of a glass prism. When a light ray passes from one medium to another, the speed of light will change. The concept of refraction is very useful for invention and development of different types of lenses and also for refracting telescopes.