
Answer
479.4k+ views
Hint: By prime factorization method take the LCM of the number of chocolates of both brands. To get the least number of boxes, find LCM (24, 15) to the number of chocolate per box.
“Complete step-by-step answer:”
Given the number of chocolate of ${{1}^{st}}$ brand in one pack = 24
The number of chocolate of ${{2}^{nd}}$ brand in one pack = 15
To find the least number of boxes of each kind first we have to find the LCM of 24 and 15.
LCM by prime factorization method.
The method of prime factorization is used to “break down” or express a given number as a product of prime numbers.
The prime number will occur more than once in the prime factorization.
Where the prime number is a whole number which is greater than 1, it is only divisible by 1 and itself.
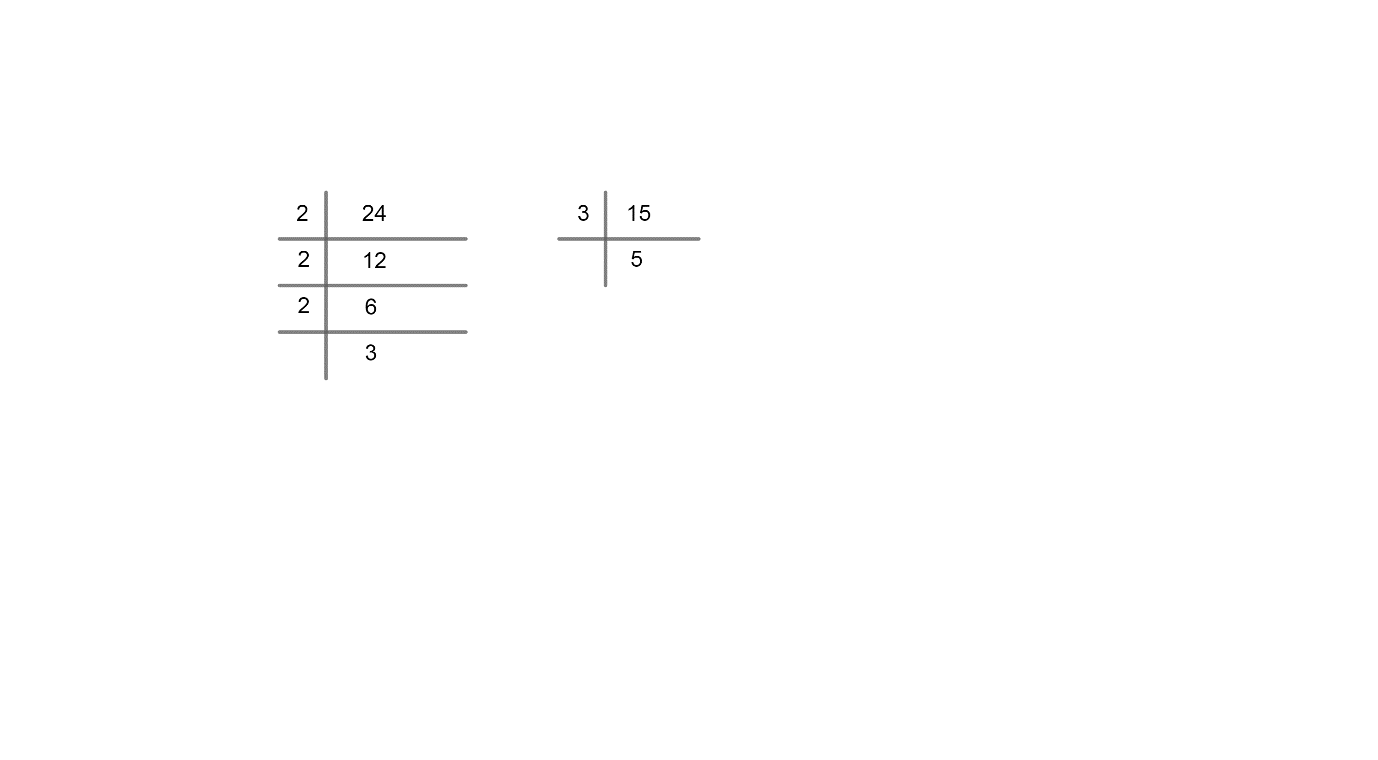
LCM of 24 $=2\times 2\times 2\times 3={{2}^{3}}\times 3$
LCM of 15 $=3\times 5$
LCM of two numbers = product of the greater power of each prime factor involved in the numbers, with highest power.
LCM of 24 \[=2\times 2\times 2\times \times 5\]
LCM of 15 $=\times 5$
$\therefore $ LCM of 24 and 15 $=2\times 2\times 2\times 3\times 5$
LCM (24, 15) = 120
Hence, the number of packet of ${{1}^{st}}$ brand $=\dfrac{\text{LCM }\left( 24,15 \right)}{\text{Number of chocolate in }{{\text{1}}^{st}}\text{ brand}}$
Number of packets in ${{2}^{nd}}$ brand $=\dfrac{\text{LCM }\left( 24,15 \right)}{\text{Number of chocolate in }{{\text{2}}^{nd}}\text{ brand}}$
$=\dfrac{120}{15}=8$
$\therefore $ 5 of first kind, 8 of second kind
Note: Another way of finding the least number of boxes of both kind,
Number of packet of ${{1}^{st}}$ brand = 24 = 3 x 8
Number of packets in ${{2}^{nd}}$ brand = 15 = 3 x 5
24 = 3 x 8
15 = 3 x 5
$\therefore $ 8 of ${{1}^{st}}$kind and 5 of ${{2}^{nd}}$kind.
“Complete step-by-step answer:”
Given the number of chocolate of ${{1}^{st}}$ brand in one pack = 24
The number of chocolate of ${{2}^{nd}}$ brand in one pack = 15
To find the least number of boxes of each kind first we have to find the LCM of 24 and 15.
LCM by prime factorization method.
The method of prime factorization is used to “break down” or express a given number as a product of prime numbers.
The prime number will occur more than once in the prime factorization.
Where the prime number is a whole number which is greater than 1, it is only divisible by 1 and itself.
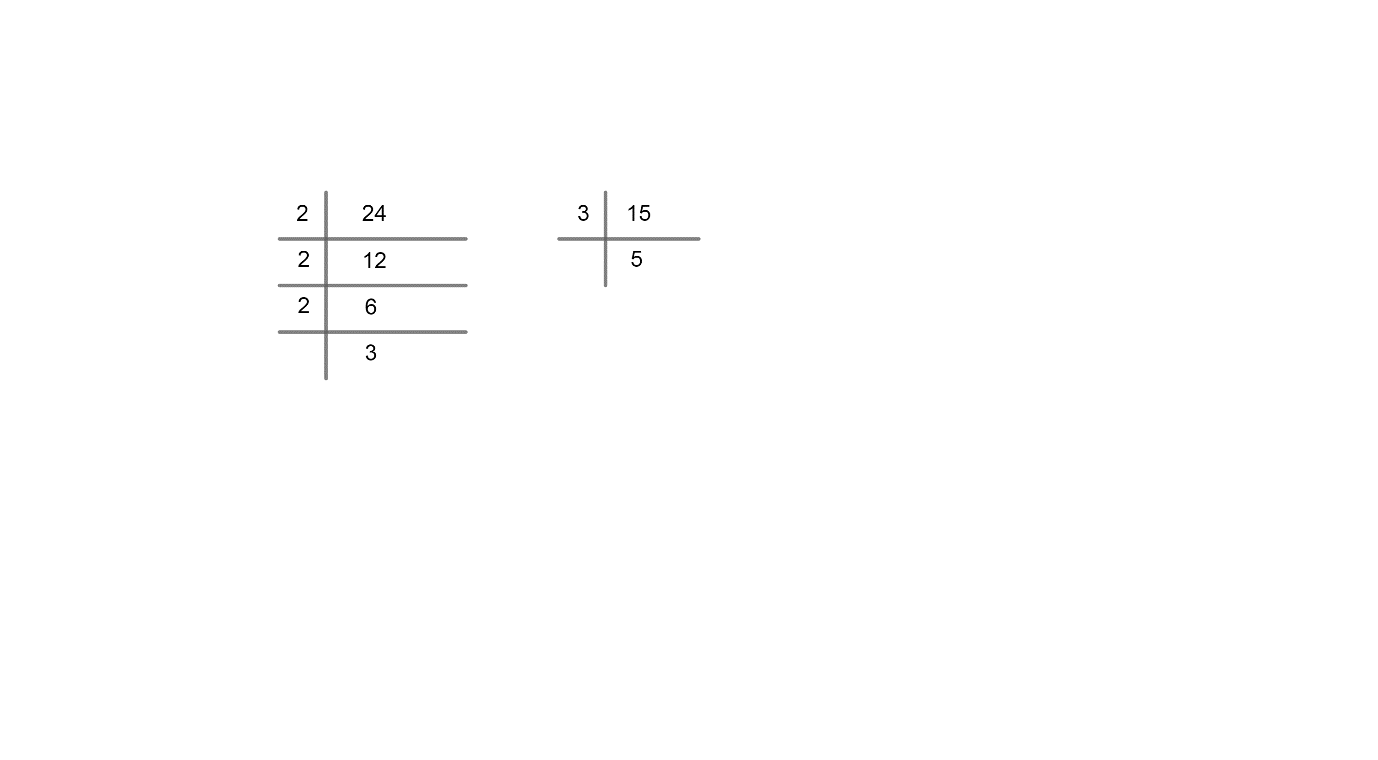
LCM of 24 $=2\times 2\times 2\times 3={{2}^{3}}\times 3$
LCM of 15 $=3\times 5$
LCM of two numbers = product of the greater power of each prime factor involved in the numbers, with highest power.
LCM of 24 \[=2\times 2\times 2\times \times 5\]
LCM of 15 $=\times 5$
$\therefore $ LCM of 24 and 15 $=2\times 2\times 2\times 3\times 5$
LCM (24, 15) = 120
Hence, the number of packet of ${{1}^{st}}$ brand $=\dfrac{\text{LCM }\left( 24,15 \right)}{\text{Number of chocolate in }{{\text{1}}^{st}}\text{ brand}}$
Number of packets in ${{2}^{nd}}$ brand $=\dfrac{\text{LCM }\left( 24,15 \right)}{\text{Number of chocolate in }{{\text{2}}^{nd}}\text{ brand}}$
$=\dfrac{120}{15}=8$
$\therefore $ 5 of first kind, 8 of second kind
Note: Another way of finding the least number of boxes of both kind,
Number of packet of ${{1}^{st}}$ brand = 24 = 3 x 8
Number of packets in ${{2}^{nd}}$ brand = 15 = 3 x 5
24 = 3 x 8
15 = 3 x 5
$\therefore $ 8 of ${{1}^{st}}$kind and 5 of ${{2}^{nd}}$kind.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Mark and label the given geoinformation on the outline class 11 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Give an account of the Northern Plains of India class 9 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE



