Question

The point (2,1) is translated parallel to the line L : x – y = 4 by $2\sqrt 3 $units. If the new point Q lies in the third quadrant, then the equation of the line passing through Q and perpendicular to L is
$
  A.2x + 2y = 1 - \sqrt 6 \\
  B.x + y = 3 - 3\sqrt 6 \\
  C.x + y = 3 - 2\sqrt 6 \\
  D.x + y = 2 - \sqrt 6 \\
$

Answer 1

Hint: Draw the line x –y = 4 and with the help of slope and distance formula we can find the value of Q. With the point Q and it is given that the line is perpendicular to x –y =4, we can find the required equation using slope point formula.

Complete step-by-step answer:
Lets sketch the line x – y =4. Let the point (2 ,1) be P.
It is given that PQ is parallel to the x – y = 4.
Now let's draw the graph with the above details

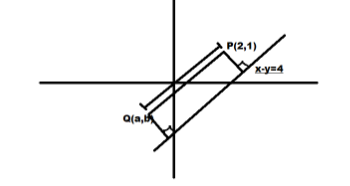
Step 2:
Let the new point be Q(a,b)
Now from the diagram , we can see that AQ is parallel to the given line.
The slope of the given line is 1
And since AQ is parallel , their slopes are equal
Therefore slope of AQ is 1
$
   \Rightarrow \dfrac{{{y_2} - {y_1}}}{{{x_2} - {x_1}}} = 1 \\
   \Rightarrow \dfrac{{1 - b}}{{2 - a}} = 1 \\
   \Rightarrow 1 - b = 2 - a \\
   \Rightarrow a - b = 1 \\
   \Rightarrow a = 1 + b \\
$
Let the above equation be equation (1)
Step 3:
It is also given that P(2 , 1) is at a distance of$2\sqrt 3 $ units from Q
Distance between two points is given by
$ \Rightarrow \sqrt {{{({x_1} - {x_2})}^2} + {{({y_1} - {y_2})}^2}} $
Here we already know that the distance between P and Q is $2\sqrt 3 $ units
Therefore ,
$
   \Rightarrow \sqrt {{{(2 - a)}^2} + {{(1 - b)}^2}} = 2\sqrt 3 \\
    \\
$
Squaring on both sides we get,
$ \Rightarrow {(2 - a)^2} + {(1 - b)^2} = {(2\sqrt 3 )^2}$
Lets substitute equation (1) in the above equation
 $
   \Rightarrow {(2 - (1 + b))^2} + {(1 - b)^2} = 4*3 \\
   \Rightarrow {(1 - b)^2} + {(1 - b)^2} = 12 \\
   \Rightarrow 2{(1 - b)^2} = 12 \\
   \Rightarrow {(1 - b)^2} = 6 \\
   \Rightarrow 1 - b = \pm \sqrt 6 \\
   \Rightarrow b = 1 \pm \sqrt 6 \\
$
Substitute the value of b in equation (1)
$
   \Rightarrow a = b + 1 \\
   \Rightarrow a = 1 \pm \sqrt 6 + 1 = 2 \pm \sqrt 6 \\
$
Step 4:
Here we have $a = 2 \pm \sqrt 6 $ and $b = 1 \pm \sqrt 6 $
Since the point Q lies in the third quadrant both the coordinates should be negative .
So $a = 2 - \sqrt 6 $and $b = 1 - \sqrt 6 $
Step 5:
Now we need to find the equation of the line passing through Q and perpendicular to x – y =4
Slope of x –y=4 is 1
Since our required equation is perpendicular to x –y=4.
The slope of our required line is -1
By using slope point formula
$(y - {y_1}) = m(x - {x_1})$
We have
$
   \Rightarrow \left( {y - (1 - \sqrt 6 )} \right) = - 1\left( {x - (2 - \sqrt 6 )} \right) \\
   \Rightarrow \left( {y - 1 + \sqrt 6 } \right) = - 1\left( {x - 2 + \sqrt 6 } \right) \\
   \Rightarrow y - 1 + \sqrt 6 = - x + 2 - \sqrt 6 \\
   \Rightarrow x + y = 2 - \sqrt 6 + 1 - \sqrt 6 \\
   \Rightarrow x + y = 3 - 2\sqrt 6 \\
$
Therefore the required equation is $x + y = 3 - 2\sqrt 6 $
The correct option is C.

Note:1. Slope of parallel lines are equal.
2.Product of the slope of perpendicular lines is -1.
3.In a graph, there are four quadrants based on the sign of x and y coordinate. In the first quadrant both x and y are positive and in the second quadrant x is negative and y is positive , in the third quadrant both x and y are negative and in the fourth quadrant y is negative and x is positive.