Question

The number of words that can be formed by using the letters of the word MATHEMATICS that start as well as end with T are
(A). 80720
(B). 90720
(C). 20860
(D). 37528

Answer 1

Hint: In this question it is given that we have to find the number of words that can be formed by using the letters of the word MATHEMATICS that start as well as end with T. So to find the solution we need to know that if you have given n numbers of objects which you need to place in r number of places then you can place it in $${}^{n}P_{r}$$ different ways,
where $${}^{n}P_{r}=\dfrac{n!}{\left( n-r\right) !}$$
Also among these objects if k number of objects are repetitive or identical then we have to divide the permutation by k!.

Complete step-by-step solution:
Given word is MATHEMATICS where the total number of letters is 11.
Now since it is given that we have to form those words which start and end with T.

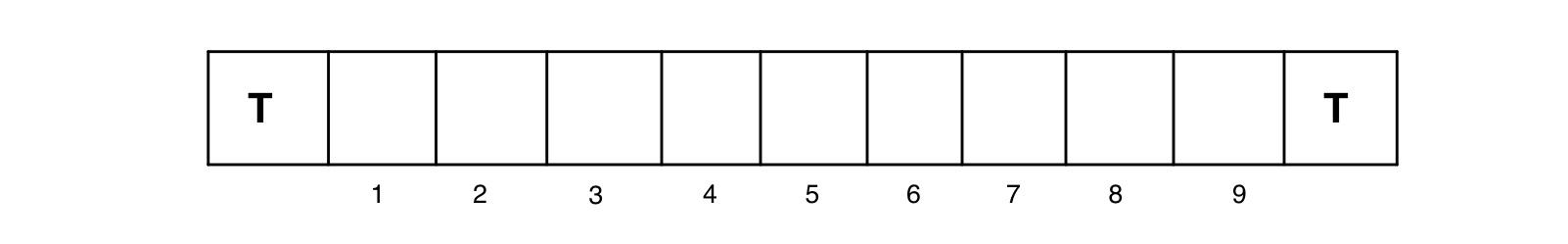
Since there are two identical letters and have to be placed in two positions so we can place it only 1 way.
Now we have the remaining 9 positions and the remaining 9 letters are M, A, H, E, M, A, I, C, S .
So by using permutation we can say that 9 letters can be placed in 9 different places in $${}^{9}P_{9}$$ ways = $$\dfrac{9!}{\left( 9-9\right) !} =\dfrac{9!}{0!} =9!$$
[since, as we know that the value of 0! =1]
Now in the given word there are 2T’s, 2M’s and 2A’s, from where 2T’s are already used, so since there are 2M’s and 2A’s, we have to divide the permutation by 2!(for 2M) and 2!( for 2A).
Therefore, the total number words = $$\dfrac{9!}{2!\times 2!}$$
                                                     =$$\dfrac{9\times 8\times 7\times 6\times 5\times 4\times 3\times 2\times 1}{2\times 1\times 2\times 1}$$
                                                     =$$\dfrac{362880}{4}$$
                                                     =$$90720$$
$$\therefore$$ the number of differential words = 90720
Hence the correct option is option B.

Note: While solving you might get confused about whether to use permutation or combination, so for this you need to know that permutation is an arrangement of things where order of arrangement matters. The position of each thing in a permutation matters. Thus, Permutation can be associated with position. Combination is grouping/selection of things where order doesn't matter. Permutation can be considered as an ordered combination.