
What is the terminal point of a vector?
Answer
448.8k+ views
Hint: First we need to understand the term vector how it is represented. For understanding the representation of vector we will consider two points in a plane as
Complete step-by-step solution:
Here we have been asked to define the term terminal point of a vector. First let us understand the definition of a vector quantity and how we represent a vector.
Now, in mathematics a vector quantity is a quantity that has both magnitude and direction. For example: - weight, force, displacement, velocity, acceleration etc. If we mention a vector only with its magnitude then it will not make any sense. For example: - if we say that a particle has a velocity of 5 m/s then it is of no meaning but we need to specify the direction like the velocity of a particle is 5 m/s along the north – east direction. If we will consider only the magnitude then it will be its speed which is a scalar quantity.
A vector is represented by an arrow
Here,
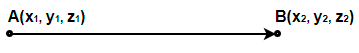
Let us consider an example where the initial point is A (2, 3, 5) and the vector AB is given is
Now, let us assume the terminal point as B(x, y, z). Therefore, using relation (i) we can write:

On comparing the coefficients of
Hence, the terminal point of the vector AB is B (1, 13, 9).
Note: Note that if you are provided with the vector and its terminal point then you can use relation (i) to get the answer and similarly if terminal and initial points are given then we can write the required vector. If we have to reverse the direction of a vector then we just multiply it with -1. The above concepts are very helpful in physics while studying about the position vectors.
Complete step-by-step solution:
Here we have been asked to define the term terminal point of a vector. First let us understand the definition of a vector quantity and how we represent a vector.
Now, in mathematics a vector quantity is a quantity that has both magnitude and direction. For example: - weight, force, displacement, velocity, acceleration etc. If we mention a vector only with its magnitude then it will not make any sense. For example: - if we say that a particle has a velocity of 5 m/s then it is of no meaning but we need to specify the direction like the velocity of a particle is 5 m/s along the north – east direction. If we will consider only the magnitude then it will be its speed which is a scalar quantity.
A vector is represented by an arrow
Here,

Let us consider an example where the initial point is A (2, 3, 5) and the vector AB is given is
Now, let us assume the terminal point as B(x, y, z). Therefore, using relation (i) we can write:

On comparing the coefficients of
Hence, the terminal point of the vector AB is B (1, 13, 9).
Note: Note that if you are provided with the vector and its terminal point then you can use relation (i) to get the answer and similarly if terminal and initial points are given then we can write the required vector. If we have to reverse the direction of a vector then we just multiply it with -1. The above concepts are very helpful in physics while studying about the position vectors.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

An example of ex situ conservation is a Sacred grove class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




