Question

Suppose three of particles each of mass \[m\]are placed at the corners of equilateral triangle of side \[l\]

Find among the options which one is \[/\]are right:

A. Moment of the inertia about axis \['2'\] is \[\dfrac{3}{4}m{{l}^{2}}\]
B. Moment of inertia about axis \['1'\]is \[\dfrac{5}{4}m{{l}^{2}}\]
C. Moment of inertia about an axis passing through one corner and perpendicular to the plane is \[2m{{l}^{2}}\]
D. All above this.

Answer 1

Hint: In order to answer this question we first know about moment of inertia. Moment of inertia , is property of a body by morality of which it goes against any agency that endeavors to place it in motion or, in the event that it is moving, to adjust the direction of its speed.

Complete step-by-step solution:
Moment of inertia is known as the body opposing angular speed which is the result of mass of each molecule i.e. \[I=m{{r}^{2}}\] . Dimension of M.O.I. is \[\left[ {{M}^{1}}{{L}^{2}}{{T}^{0}} \right]\]
Let's draw a fig. to solve this problem quickly,

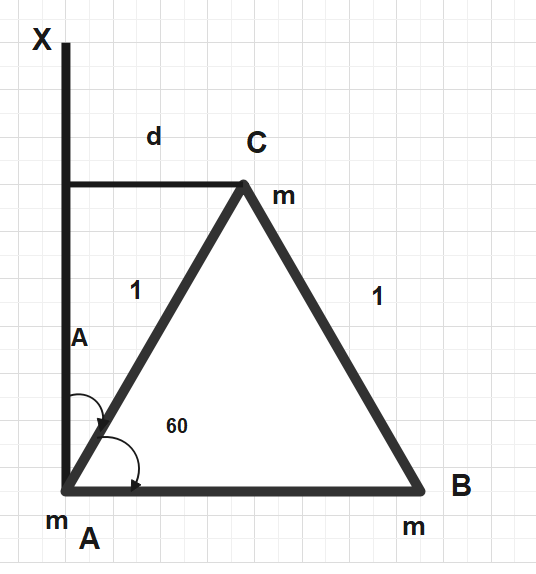
 As , we know moment of inertia about any axis \[I=m{{r}^{2}}\]
From this above fig. we get \[\sin {{30}^{\circ }}=\dfrac{d}{1}\]
Now M.O.I. about axis \['1'\] \[\Rightarrow {{I}_{1}}=m{{\left( \dfrac{l}{2} \right)}^{2}}+m{{l}^{2}}\]
                                                \[\Rightarrow {{I}_{1}}=\dfrac{m{{l}^{2}}}{4}+m{{l}^{2}}\]
                                                  \[\therefore {{I}_{1}}=\dfrac{5}{4}m{{l}^{2}}\]
And M.O.I about axis \['2'\] \[\Rightarrow {{I}_{2}}=m{{\left( \dfrac{\sqrt{3}l}{2} \right)}^{2}}\]
                                                   \[\Rightarrow {{I}_{2}}=\dfrac{3m{{l}^{2}}}{4}\]
Assume that the axis passing through the one corner and also perpendicular to plane \[\Rightarrow {{I}_{{}}}=m{{l}^{2}}+m{{l}^{2}}\]
\[\therefore I=2m{{l}^{2}}\]
Therefore all of the above options are correct.

 Note: Moment of inertia comes from the Latin word “momentum” which simply means movement . Decreasing in rate of speed of rotation , is due to the increase of axis of rotation as the moment of inertia also increases.