Question

Prove that ${{\cos }^{-1}}\left( \dfrac{1}{x} \right)={{\sec }^{-1}}x,x\in \left( -\infty ,-1 \right]\bigcup \left[ 1,\infty \right)$

Answer 1

Hint: Use the fact that if $y={{\cos }^{-1}}x$, then $x=\cos y$. Assume $y={{\cos }^{-1}}\left( \dfrac{1}{x} \right)$. Use the previously mentioned fact and write x in terms of y. Take ${{\sec }^{-1}}$ on both side and use the fact that if $y\in \left[ 0,\pi \right]-\left\{ \dfrac{\pi }{2} \right\}$, then ${{\sec }^{-1}}\left( \sec y \right)=y$ and hence prove the result.

Complete step-by-step answer:
Before dwelling into the proof of the above question, we must understand how ${{\cos }^{-1}}x$ is defined even when $\cos x$ is not one-one.
We know that cosx is a periodic function.
Let us draw the graph of cosx

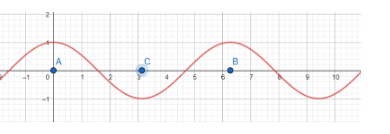
As is evident from the graph cosx is a repeated chunk of the graph of cosx within the interval $\left[ A,B \right]$ , and it attains all its possible values in the interval $\left[ A,C \right]$. Here $A=0,B=2\pi $ and $C=\pi $
Hence if we consider cosx in the interval [A, C], we will lose no value attained by cosx, and at the same time, cosx will be one-one and onto.
Hence ${{\cos }^{-1}}x$ is defined over the domain $\left[ -1,1 \right]$, with codomain $\left[ 0,\pi \right]$ as in the domain $\left[ 0,\pi \right]$, cosx is one-one and ${{R}_{\cos x}}=\left[ -1,1 \right]$.
Now since $\arccos x$ is the inverse of cosx it satisfies the fact that if $y=\arccos x$, then $\cos y=x$.
So let $y={{\cos }^{-1}}\left( \dfrac{1}{x} \right)$, $y\in \left[ 0,\pi \right]-\left\{ \dfrac{\pi }{2} \right\}$ as $\dfrac{1}{x}\ne 0$
Hence we have $\cos y=\dfrac{1}{x}$
Hence we have $x=\dfrac{1}{\cos y}=\sec y$
Taking ${{\sec }^{-1}}$ on both sides, we get
${{\sec }^{-1}}x={{\sec }^{-1}}\left( \sec y \right)$
Now since $y\in \left[ 0,\pi \right]-\left\{ \dfrac{\pi }{2} \right\}$ and we know that if $y\in \left[ 0,\pi \right]-\left\{ \dfrac{\pi }{2} \right\}$, then ${{\sec }^{-1}}\left( \sec y \right)=y$ [Valid only in principal branch]
Hence we have
$\begin{align}
  & {{\sec }^{-1}}x=y \\
 & \Rightarrow y={{\sec }^{-1}}y \\
\end{align}$
Reverting to the original variable, we get
${{\cos }^{-1}}\left( \dfrac{1}{x} \right)={{\sec }^{-1}}\left( x \right)$
Since $\dfrac{1}{x}$ is in the domain of ${{\cos }^{-1}}x$, we get
$\dfrac{1}{x}\in \left[ -1,1 \right]\Rightarrow x\in \left( -\infty ,-1 \right]\bigcup \left[ 1,\infty \right)$
Hence we have ${{\cos }^{-1}}\left( \dfrac{1}{x} \right)={{\sec }^{-1}}x,x\in \left( -\infty ,-1 \right]\bigcup \left[ 1,\infty \right)$

Note: [1] The above-specified codomain for ${{\cos }^{-1}}x$ is called principal branch for${{\cos }^{-1}}x$ . We can select any branch as long as $\cos x$ is one-one and onto and Range $=\left[ -1,1 \right]$. Like instead of $\left[ 0,\pi \right]$, we can select the interval $\left[ \pi ,2\pi \right]$. The proof will remain the same as above.