Question

\[P,Q\] \[and{\text{ }}R\] are three coplanar forces acting at a point and they are in equilibrium. Given\[P = 1.9318Kg{\text{ }}wt\] , \[\sin {\theta _1} = 0.9659\] , Find the value of \[R\left( {in{\text{ }}Kg{\text{ }}wt} \right)\].

Answer 1

Hint: For solving this problem we need to have a clear idea of Lami’s Theorem.
According to this theorem when 3 forces are acting at a point and they are in equilibrium, then each force is proportional to the sine of the angle between the other two forces.
Lami’s theorem is useful for determining the magnitude of unknown forces for a given system.
With this theorem, the property of 3 forces in equilibrium is considered to be a very neat method of solving any kind of three-force problem. If we know the angles between pairs of forces the best method to find these out is Lami’s theorem.
The 3 forces should be inward or outward and opposite.


Formula used:
Simply follow Lami’s Theorem formula in this case
$\dfrac{A}{{\operatorname{Sin} \alpha }} = \dfrac{B}{{\operatorname{Sin} \beta }} = \dfrac{C}{{\operatorname{Sin} \gamma }}$


Complete step-by-step solution:

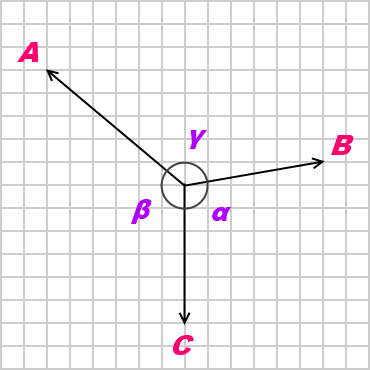
According to the statement of this theorem when 3 forces are acting at a point and they are in equilibrium, then each force is proportional to the sine of the angle between the other two forces.
$\dfrac{A}{{\operatorname{Sin} \alpha }} = \dfrac{B}{{\operatorname{Sin} \beta }} = \dfrac{C}{{\operatorname{Sin} \gamma }}$
Using the above formula for Lami’s theorem
\[\dfrac{P}{{\sin {\theta _1}}} = \dfrac{Q}{{\sin {\theta _2}}} = \dfrac{R}{{\sin 150}}\]
$\therefore \dfrac{P}{{\sin {\theta _1}}} = \dfrac{R}{{\operatorname{Sin} 150}}$
Put values P=1.9318 Kg wt, \[\sin {\theta _1} = 0.9659\] in the above equation we get,
$ \Rightarrow \dfrac{{1.9318}}{{0.9659}} = \dfrac{R}{{0.5}}$
$\therefore \dfrac{{1.9318 \times 0.5}}{{0.9659}} = R$
So, finally the value of\[R = 1Kg{\text{ }}wt\] .

Additional information:
Application of Lami’s Theorem
The derivation of this theorem is from the Sine rule of triangles. In a free body diagram if we represent the forces as lines and translate them in such a way that one head touches the tail of another then we will notice that if the 3 forces are supposed to cancel each other then they will resultantly form a triangle.

Note: The Theorem is named after Benard Lammy. The theorem is related to the magnitude of coplanar, concurrent, non-collinear forces that help to maintain an object in a static equilibrium position.
For analyzing most of the mechanical and structural this theorem is very much helpful. This theorem is applied in a static analysis of structural and mechanical systems.
Besides usefulness, there are also certain limitations of Lami’s Theorem such as
1)There should be only three forces present.
2)The 3 forces should be in a single plane.
3)The 3 forces should remain concurrent.
4)The 3 forces must be in the equilibrium point
5)No angle between these 3 forces should be 180degrees i.e their line of action should not overlap with each other.