Answer
401.7k+ views
Hint:For this question mid-point theorem is to be used.
Mid-point theorem: - It says a line segment joining the mid-points of two sides of a triangle is parallel to the third side and equal to half the length of the third side.
Example: -
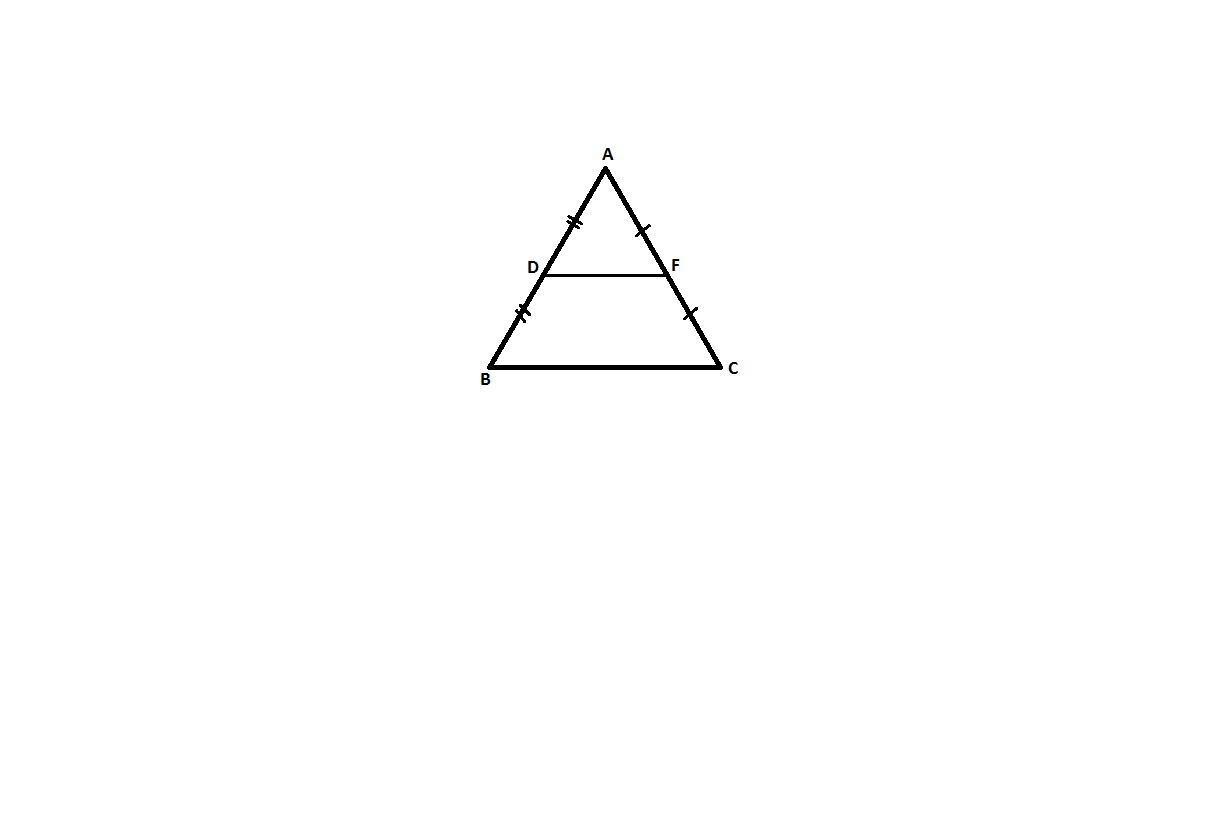
In triangle ABC, D is midpoint of AB and E is midpoint of AC.
$
\therefore DF//BC\\
\therefore DF = \dfrac{1}{2}BC $
So we can say that it bisects the line into two equal parts.
Complete step-by-step answer:
Construction: - Draw DG parallel to BF, which meets FC at G.
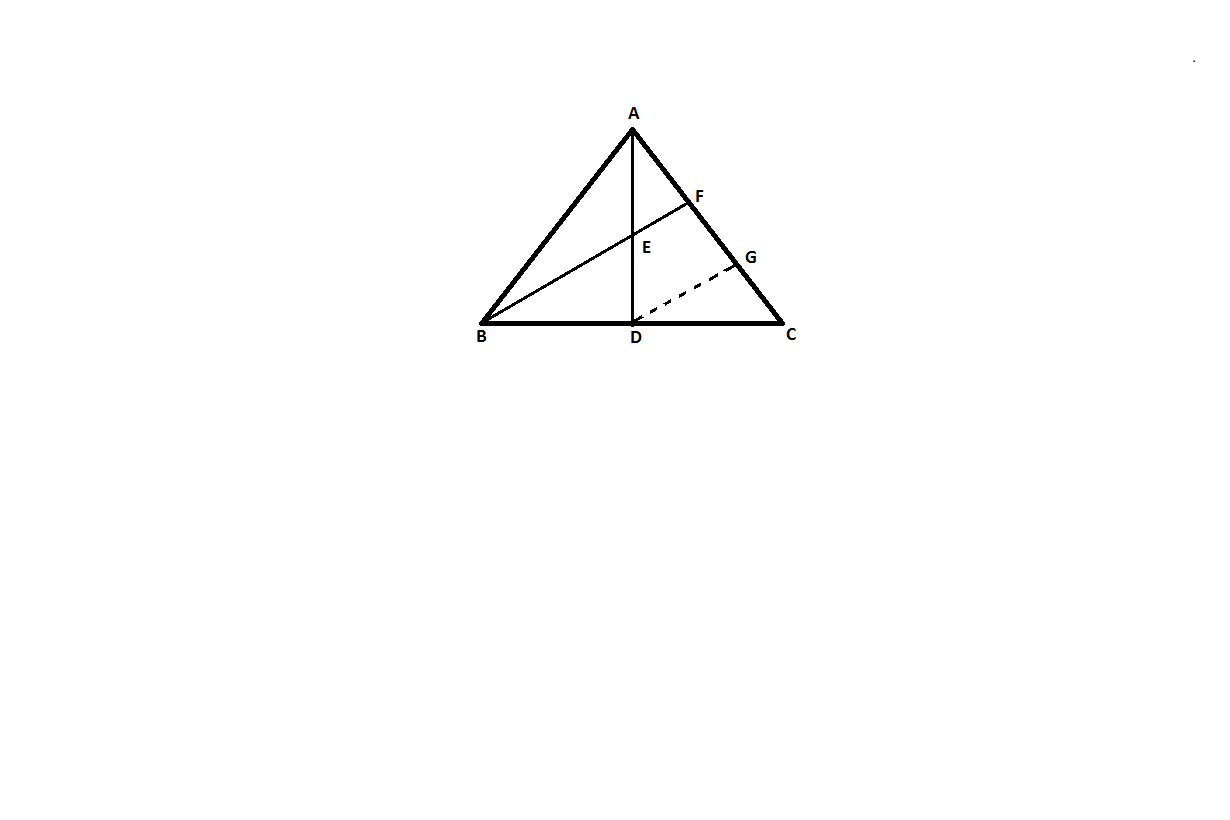
Proof: - In triangle ABC, AD is median and E is the midpoint of AD.
In and E is the midpoint of AD. Hence, F is midpoint of AG (midpoint theorem)
Or, $AF = FG$ ................equation(1)
In and D is the midpoint of BC.
$\therefore $ G is midpoint of CF (midpoint theorem)
Or, $FG = GC$ .................equation(2)
From equation (1) and (2)
$AF = FG = GC$
Since: - $AC = AF + FG + GC$
$AC = 3AF$
Hence proved.
Note: Carefully observe the hint which is given in the question and then proceed for the question. Here $DG\parallel BF$ gives a hint that the mid- point theorem is to be used. Similar questions can be framed with the use of a converse mid-point theorem.
Converse mid-point theorem: - It states that when a line is drawn through the midpoint of a side of a triangle which is parallel to the second side then it will bisect the third side.
Mid-point theorem: - It says a line segment joining the mid-points of two sides of a triangle is parallel to the third side and equal to half the length of the third side.
Example: -
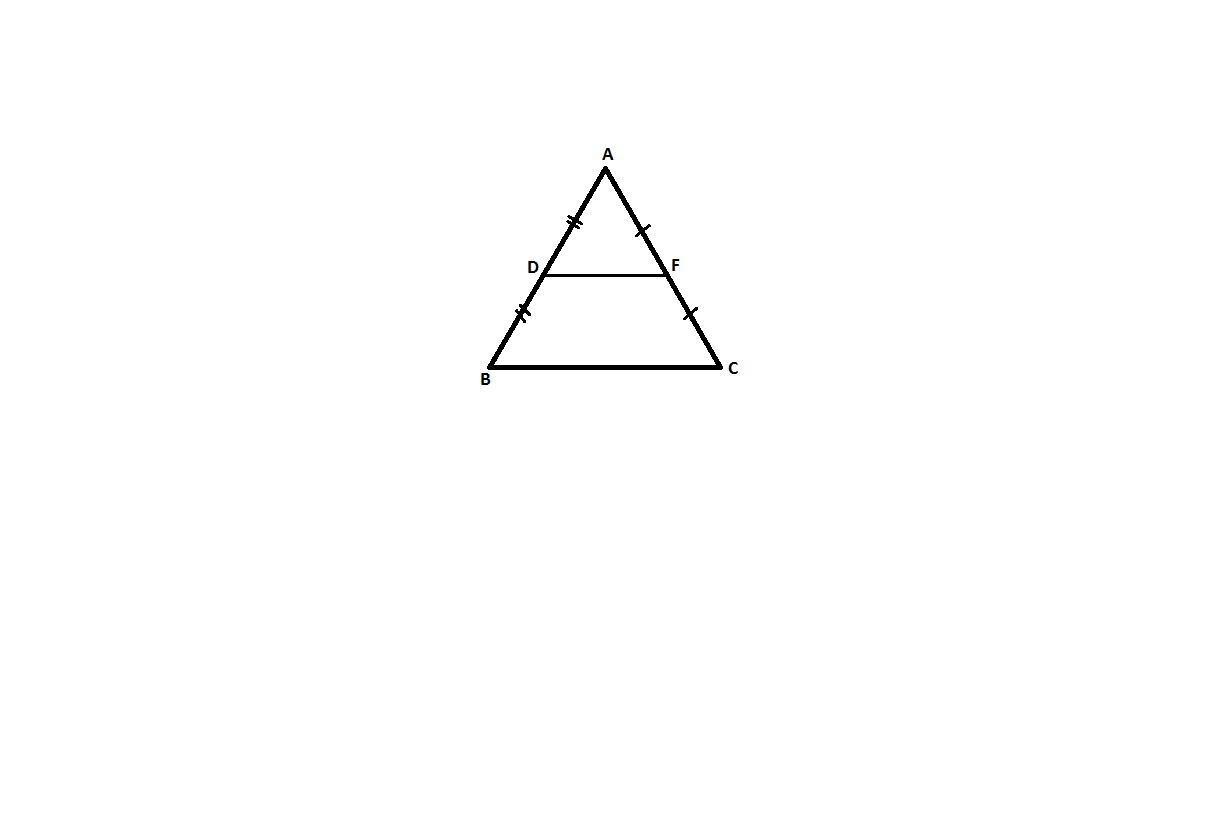
In triangle ABC, D is midpoint of AB and E is midpoint of AC.
$
\therefore DF//BC\\
\therefore DF = \dfrac{1}{2}BC $
So we can say that it bisects the line into two equal parts.
Complete step-by-step answer:
Construction: - Draw DG parallel to BF, which meets FC at G.
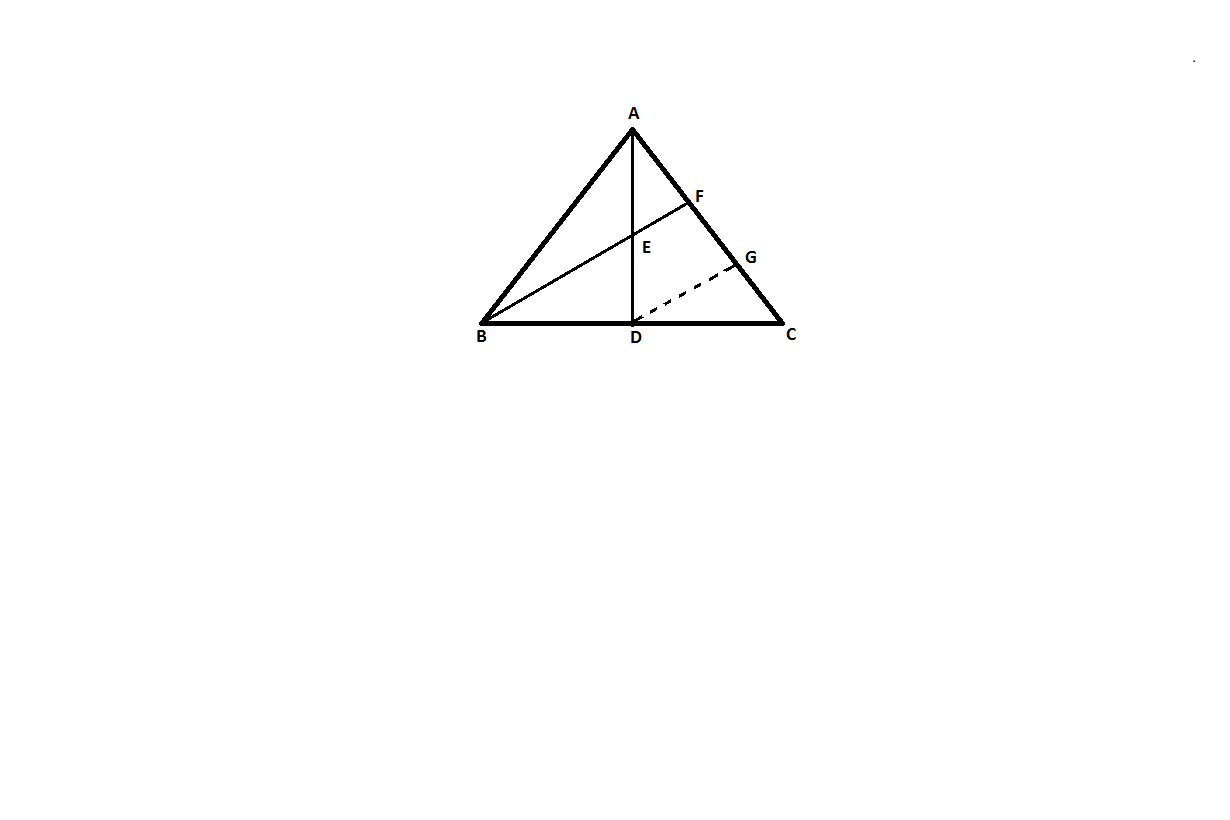
Proof: - In triangle ABC, AD is median and E is the midpoint of AD.
In and E is the midpoint of AD. Hence, F is midpoint of AG (midpoint theorem)
Or, $AF = FG$ ................equation(1)
In and D is the midpoint of BC.
$\therefore $ G is midpoint of CF (midpoint theorem)
Or, $FG = GC$ .................equation(2)
From equation (1) and (2)
$AF = FG = GC$
Since: - $AC = AF + FG + GC$
$AC = 3AF$
Hence proved.
Note: Carefully observe the hint which is given in the question and then proceed for the question. Here $DG\parallel BF$ gives a hint that the mid- point theorem is to be used. Similar questions can be framed with the use of a converse mid-point theorem.
Converse mid-point theorem: - It states that when a line is drawn through the midpoint of a side of a triangle which is parallel to the second side then it will bisect the third side.
Recently Updated Pages
Three beakers labelled as A B and C each containing 25 mL of water were taken A small amount of NaOH anhydrous CuSO4 and NaCl were added to the beakers A B and C respectively It was observed that there was an increase in the temperature of the solutions contained in beakers A and B whereas in case of beaker C the temperature of the solution falls Which one of the following statements isarecorrect i In beakers A and B exothermic process has occurred ii In beakers A and B endothermic process has occurred iii In beaker C exothermic process has occurred iv In beaker C endothermic process has occurred

The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Trending doubts
Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Which of the following is not a primary colour A Yellow class 10 physics CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What organs are located on the left side of your body class 11 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths



