Answer
378k+ views
Hint: In this problem, we have to find the area of the given plot. Here we can see that figure 1 has three triangles and one trapezium in it, where the sides of each figure are given in metres. We can first find the area for each of the triangles and the trapezium using the respective area formulas and then we can add them to get the area of the given figure. In figure 2, we can find the required side measure using Pythagoras theorem, we can find the area of each shape and add them.
Complete step by step answer:
1.Here we have to find the area of the below figure.
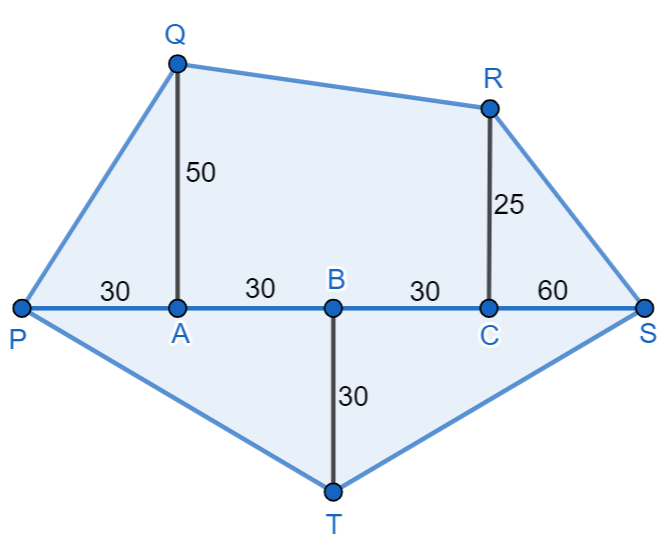
Here, we can see that \[\Delta QAP,\Delta RCS\] are right angled triangles and QACR is a trapezium.
We are given that, in triangle QAP,
Length = 50m, breadth = 30m.
We know that,
Area of a triangle = \[\dfrac{1}{2}\times l\times b\].
We can now substitute the above values in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 50\times 30=750\]
The area of triangle QAP = 750 square meter. …….. (1)
We know that in triangle RCS,
Length = 25m, breadth = 60m
We can now substitute the above values in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 25\times 60=750\]
The area of triangle RCS = 750 square meter. ……….. (2)
We can now find the area of the trapezium QACR, whose measures given are
\[l\left( QA \right)=50m,l\left( RC \right)=25m,l\left( AC \right)=60m\]
We know that,
The area of the trapezium = \[\dfrac{1}{2}\times \text{ Sum of length of parallel sides }\times \text{ height}\].
We can now substitute the value in the above formula, we get
\[\begin{align}
& \Rightarrow \dfrac{1}{2}\times \left( 50+25 \right)\times 60 \\
& \Rightarrow \dfrac{1}{2}\times 75\times 60=2250 \\
\end{align}\]
The area of trapezium QACR = 2250 square meters. ……. (3)
We can now find the are of the triangle PTS, whose measures are,
Length = 30, breadth = 150.
We can now substitute in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 30\times 150=2250\]
The area of the triangle PTS = 2250 square meter. ……… (4)
We can now add (1), (2), (3), (4), we get
Area of the plot QPTSR = Area of triangle QAP + Area of triangle RCS + Area of trapezium QACR + Area of triangle PTS.
\[\Rightarrow 750+750+2250+2250=6000sq.m\]
Therefore, the area of the given plot is 6000 square meters.
2. Here we can find the area of the below diagram.
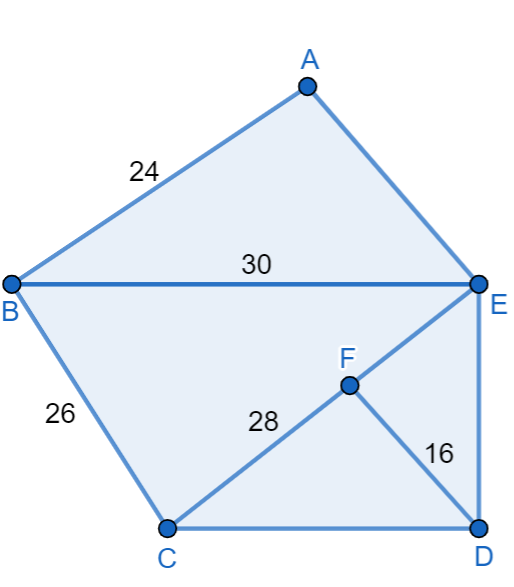
We can see that, in triangle ABE, \[m\angle BAE={{90}^{\circ }}\].
We can now use the Pythagoras theorem to find AE, we get
\[\begin{align}
& \Rightarrow {{\left( BE \right)}^{2}}={{\left( AB \right)}^{2}}+{{\left( AE \right)}^{2}} \\
& \Rightarrow {{\left( 30 \right)}^{2}}={{\left( 24 \right)}^{2}}+{{\left( AE \right)}^{2}} \\
& \Rightarrow AE=\sqrt{900-576}=\sqrt{324}=18m \\
\end{align}\]
Therefore, the breadth AE = 18m.
We can now find the area of triangle ABE, we get
Area of triangle ABE = \[\dfrac{1}{2}\times 18\times 24=216sq.m\].
Area of triangle ABE = 216 square meter. ….. (1) \[\]
We can now find the semi perimeter of triangle BCE,
\[\begin{align}
& \Rightarrow s=\dfrac{1}{2}\left( a+b+c \right) \\
& \Rightarrow s=\dfrac{30+28+26}{2} \\
& \Rightarrow s=42m \\
\end{align}\]
We can now find the area using the semi perimeter, we get
Area of triangle BCE,
\[\begin{align}
& \Rightarrow \sqrt{s\left( s-a \right)\left( s-b \right)\left( s-c \right)} \\
& \Rightarrow \sqrt{42\left( 42-30 \right)\left( 42-28 \right)\left( 42-26 \right)} \\
& \Rightarrow \sqrt{42\times 12\times 14\times 16} \\
& \Rightarrow 336sq.m \\
\end{align}\]
The area of BCE is 336 square meter …….. (2)
Now area of triangle EDC is,
\[\Rightarrow \dfrac{1}{2}\times 28\times 16=224\]
Therefore, the area of triangle EDC is 224 square meter…… (3)
We can now add (1), (2), (3), we get
Area of the plot ABCDE = 216 + 336 + 224 = 776 square meter.
Therefore, the area of the given plot is 776 square meter.
Note: We should always remember that the area of the triangle formula is \[\dfrac{1}{2}\times l\times b\] and the area of the trapezium formula is \[\dfrac{1}{2}\times \text{ Sum of length of parallel sides }\times \text{ height}\]. We can find the area of each shape and add them to find the area of the given region.
Complete step by step answer:
1.Here we have to find the area of the below figure.

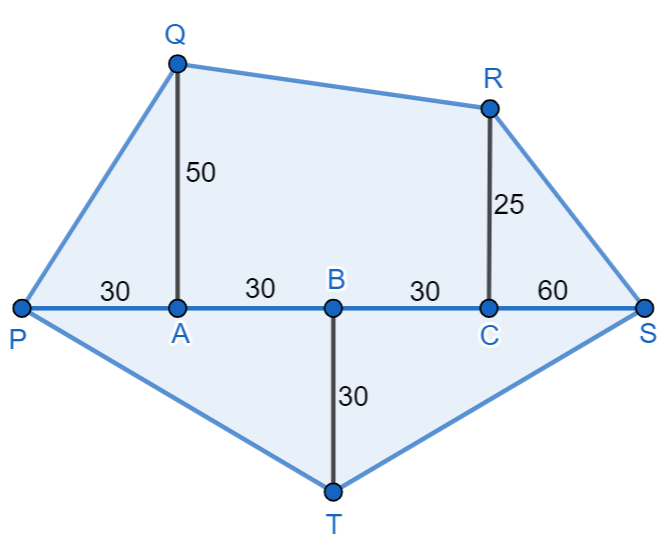
Here, we can see that \[\Delta QAP,\Delta RCS\] are right angled triangles and QACR is a trapezium.
We are given that, in triangle QAP,
Length = 50m, breadth = 30m.
We know that,
Area of a triangle = \[\dfrac{1}{2}\times l\times b\].
We can now substitute the above values in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 50\times 30=750\]
The area of triangle QAP = 750 square meter. …….. (1)
We know that in triangle RCS,
Length = 25m, breadth = 60m
We can now substitute the above values in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 25\times 60=750\]
The area of triangle RCS = 750 square meter. ……….. (2)
We can now find the area of the trapezium QACR, whose measures given are
\[l\left( QA \right)=50m,l\left( RC \right)=25m,l\left( AC \right)=60m\]
We know that,
The area of the trapezium = \[\dfrac{1}{2}\times \text{ Sum of length of parallel sides }\times \text{ height}\].
We can now substitute the value in the above formula, we get
\[\begin{align}
& \Rightarrow \dfrac{1}{2}\times \left( 50+25 \right)\times 60 \\
& \Rightarrow \dfrac{1}{2}\times 75\times 60=2250 \\
\end{align}\]
The area of trapezium QACR = 2250 square meters. ……. (3)
We can now find the are of the triangle PTS, whose measures are,
Length = 30, breadth = 150.
We can now substitute in the formula, we get
\[\Rightarrow \dfrac{1}{2}\times 30\times 150=2250\]
The area of the triangle PTS = 2250 square meter. ……… (4)
We can now add (1), (2), (3), (4), we get
Area of the plot QPTSR = Area of triangle QAP + Area of triangle RCS + Area of trapezium QACR + Area of triangle PTS.
\[\Rightarrow 750+750+2250+2250=6000sq.m\]
Therefore, the area of the given plot is 6000 square meters.
2. Here we can find the area of the below diagram.

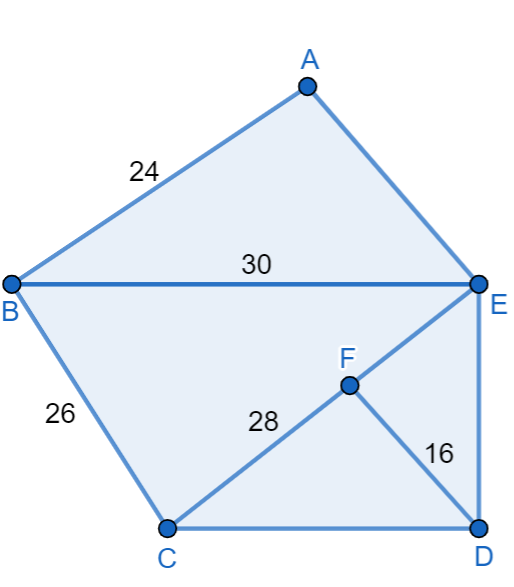
We can see that, in triangle ABE, \[m\angle BAE={{90}^{\circ }}\].
We can now use the Pythagoras theorem to find AE, we get
\[\begin{align}
& \Rightarrow {{\left( BE \right)}^{2}}={{\left( AB \right)}^{2}}+{{\left( AE \right)}^{2}} \\
& \Rightarrow {{\left( 30 \right)}^{2}}={{\left( 24 \right)}^{2}}+{{\left( AE \right)}^{2}} \\
& \Rightarrow AE=\sqrt{900-576}=\sqrt{324}=18m \\
\end{align}\]
Therefore, the breadth AE = 18m.
We can now find the area of triangle ABE, we get
Area of triangle ABE = \[\dfrac{1}{2}\times 18\times 24=216sq.m\].
Area of triangle ABE = 216 square meter. ….. (1) \[\]
We can now find the semi perimeter of triangle BCE,
\[\begin{align}
& \Rightarrow s=\dfrac{1}{2}\left( a+b+c \right) \\
& \Rightarrow s=\dfrac{30+28+26}{2} \\
& \Rightarrow s=42m \\
\end{align}\]
We can now find the area using the semi perimeter, we get
Area of triangle BCE,
\[\begin{align}
& \Rightarrow \sqrt{s\left( s-a \right)\left( s-b \right)\left( s-c \right)} \\
& \Rightarrow \sqrt{42\left( 42-30 \right)\left( 42-28 \right)\left( 42-26 \right)} \\
& \Rightarrow \sqrt{42\times 12\times 14\times 16} \\
& \Rightarrow 336sq.m \\
\end{align}\]
The area of BCE is 336 square meter …….. (2)
Now area of triangle EDC is,
\[\Rightarrow \dfrac{1}{2}\times 28\times 16=224\]
Therefore, the area of triangle EDC is 224 square meter…… (3)
We can now add (1), (2), (3), we get
Area of the plot ABCDE = 216 + 336 + 224 = 776 square meter.
Therefore, the area of the given plot is 776 square meter.
Note: We should always remember that the area of the triangle formula is \[\dfrac{1}{2}\times l\times b\] and the area of the trapezium formula is \[\dfrac{1}{2}\times \text{ Sum of length of parallel sides }\times \text{ height}\]. We can find the area of each shape and add them to find the area of the given region.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Mark and label the given geoinformation on the outline class 11 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE

Name the states which share their boundary with Indias class 9 social science CBSE

Give an account of the Northern Plains of India class 9 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference Between Plant Cell and Animal Cell

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Change the following sentences into negative and interrogative class 10 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE