Answer
384k+ views
Hint: We solve this by linear programming that is by drawing a graph of inequalities. Let ‘\[x\]’ grams of wheat are mixed and ‘\[y\]’ grams of rice are mixed. Using this we will get two inequities. We find the values by equating ‘\[x\]’ to zero, we get ‘\[y\]’ and vice versa. Plotting a graph we can find the minimum cost.
Complete step-by-step solution:
Let \[x\] gram of wheat and y grams of rice are mixed in a daily diet.
Given, every gram of wheat provides 0.1g of proteins and every gram of rice gives 0.05g of proteins. Therefore, \[x\] grams of wheat and \[y\] grams of rice will provide \[0.1x + 0.05y\] grams of proteins. But the minimum requirement of proteins is 50g.
That is, \[0.1x + 0.05y \geqslant 50\].
To get the simplified form multiply and divide by 100 on each term,
\[ \Rightarrow 0.1x \times \dfrac{{100}}{{100}} + 0.05y \times \dfrac{{100}}{{100}} \geqslant 50 \times \dfrac{{100}}{{100}}\]
\[ \Rightarrow \dfrac{{10x}}{{100}} + \dfrac{{5y}}{{100}} \geqslant 50\]
Cancelling, we get,
\[ \Rightarrow \dfrac{x}{{10}} + \dfrac{y}{{20}} \geqslant 50\] ----- (1)
Similarly \[x\]grams of wheat and \[y\]grams of rice will provide\[0.25x + 0.5y\]grams of carbohydrates and the minimum daily requirement is 200 grams.
That is, \[0.25x + 0.5y \geqslant 200\].
To get the simplified form multiply and divide by 100 on each term,
\[ \Rightarrow 0.25x \times \dfrac{{100}}{{100}} + 0.5y \times \dfrac{{100}}{{100}} \geqslant 200 \times \dfrac{{100}}{{100}}\]
\[ \Rightarrow \dfrac{{25x}}{{100}} + \dfrac{{50y}}{{100}} \geqslant 200\]
Cancelling, we get:
\[ \Rightarrow \dfrac{x}{4} + \dfrac{y}{2} \geqslant 200\] ----- (2)
Also quantities of wheat and rice will never be negative.
\[x \geqslant 0\]and \[y \geqslant 0\]
It is given that wheat costs Rs.4 per kg and rice Rs.6 per kg. So we have,
\[\dfrac{{4x}}{{1000}} + \dfrac{{6y}}{{1000}} = z\]minimum.
Subject to the constraints
\[\dfrac{x}{{10}} + \dfrac{y}{{20}} \geqslant 50\], \[\dfrac{x}{4} + \dfrac{y}{2} \geqslant 200\], \[x \geqslant 0\], \[y \geqslant 0\].
Now to find the points ,
Put \[x = 0\] in equation (1) we have,
\[ \Rightarrow \dfrac{0}{{10}} + \dfrac{y}{{20}} = 50\]
\[ \Rightarrow \dfrac{y}{{20}} = 50\]
\[ \Rightarrow y = 1000\]. Thus we have (0, 1000).
Similarly put \[y = 0\] in equation (1)
\[ \Rightarrow \dfrac{x}{{10}} = 50\]
\[ \Rightarrow x = 500\]. Thus we have (500, 0).
Similarly put \[x = 0\] in equation (2), we have:
\[ \Rightarrow \dfrac{y}{2} = 200\]
\[ \Rightarrow y = 400\]. Thus we have (0, 400)
Similarly put \[y = 0\] in equation (2)
\[ \Rightarrow \dfrac{x}{4} = 200\]
\[ \Rightarrow x = 800\]. Thus we have (800, 0).
Thus we have the points, \[(0,1000)\], \[(500,0)\], \[(0,400)\]and \[(800,0)\]. Plotting a graph for this.
Scale: X-axis = 1 unit =100 units, Y-axis = 1 unit =100 units.
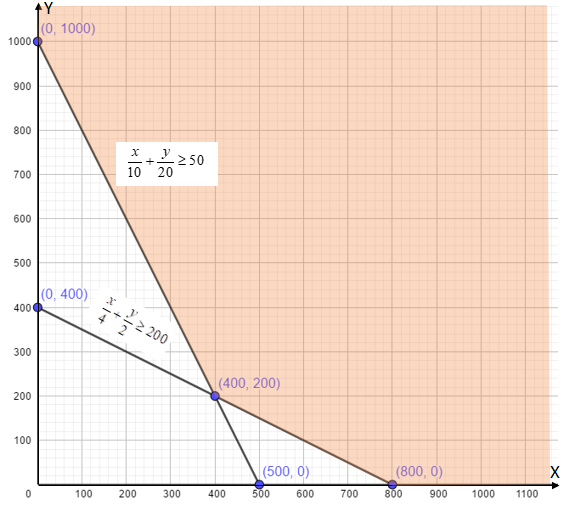
The values of the objective function at these points are given in the following table:
Clearly, Z is minimum for \[x = 400\]grams of wheat and \[y = 200\] grams of rice. The minimum cost is Rs.\[2.8\]
Note: All we did in above is converting the given word problem into inequality problems. As we know that we need a point to join a line, hence we find the inequality value at ‘x’ is zero and then at ‘y’ is zero. Also if they mention minimum we take greater than or equal to(\[ \geqslant \]). If they mention maximum we take less than or equal to (\[ \leqslant \]).
Complete step-by-step solution:
Let \[x\] gram of wheat and y grams of rice are mixed in a daily diet.
Given, every gram of wheat provides 0.1g of proteins and every gram of rice gives 0.05g of proteins. Therefore, \[x\] grams of wheat and \[y\] grams of rice will provide \[0.1x + 0.05y\] grams of proteins. But the minimum requirement of proteins is 50g.
That is, \[0.1x + 0.05y \geqslant 50\].
To get the simplified form multiply and divide by 100 on each term,
\[ \Rightarrow 0.1x \times \dfrac{{100}}{{100}} + 0.05y \times \dfrac{{100}}{{100}} \geqslant 50 \times \dfrac{{100}}{{100}}\]
\[ \Rightarrow \dfrac{{10x}}{{100}} + \dfrac{{5y}}{{100}} \geqslant 50\]
Cancelling, we get,
\[ \Rightarrow \dfrac{x}{{10}} + \dfrac{y}{{20}} \geqslant 50\] ----- (1)
Similarly \[x\]grams of wheat and \[y\]grams of rice will provide\[0.25x + 0.5y\]grams of carbohydrates and the minimum daily requirement is 200 grams.
That is, \[0.25x + 0.5y \geqslant 200\].
To get the simplified form multiply and divide by 100 on each term,
\[ \Rightarrow 0.25x \times \dfrac{{100}}{{100}} + 0.5y \times \dfrac{{100}}{{100}} \geqslant 200 \times \dfrac{{100}}{{100}}\]
\[ \Rightarrow \dfrac{{25x}}{{100}} + \dfrac{{50y}}{{100}} \geqslant 200\]
Cancelling, we get:
\[ \Rightarrow \dfrac{x}{4} + \dfrac{y}{2} \geqslant 200\] ----- (2)
Also quantities of wheat and rice will never be negative.
\[x \geqslant 0\]and \[y \geqslant 0\]
It is given that wheat costs Rs.4 per kg and rice Rs.6 per kg. So we have,
\[\dfrac{{4x}}{{1000}} + \dfrac{{6y}}{{1000}} = z\]minimum.
Subject to the constraints
\[\dfrac{x}{{10}} + \dfrac{y}{{20}} \geqslant 50\], \[\dfrac{x}{4} + \dfrac{y}{2} \geqslant 200\], \[x \geqslant 0\], \[y \geqslant 0\].
Now to find the points ,
Put \[x = 0\] in equation (1) we have,
\[ \Rightarrow \dfrac{0}{{10}} + \dfrac{y}{{20}} = 50\]
\[ \Rightarrow \dfrac{y}{{20}} = 50\]
\[ \Rightarrow y = 1000\]. Thus we have (0, 1000).
Similarly put \[y = 0\] in equation (1)
\[ \Rightarrow \dfrac{x}{{10}} = 50\]
\[ \Rightarrow x = 500\]. Thus we have (500, 0).
Similarly put \[x = 0\] in equation (2), we have:
\[ \Rightarrow \dfrac{y}{2} = 200\]
\[ \Rightarrow y = 400\]. Thus we have (0, 400)
Similarly put \[y = 0\] in equation (2)
\[ \Rightarrow \dfrac{x}{4} = 200\]
\[ \Rightarrow x = 800\]. Thus we have (800, 0).
Thus we have the points, \[(0,1000)\], \[(500,0)\], \[(0,400)\]and \[(800,0)\]. Plotting a graph for this.
Scale: X-axis = 1 unit =100 units, Y-axis = 1 unit =100 units.

The values of the objective function at these points are given in the following table:
| Corner points | Value of \[z = \dfrac{{4x}}{{1000}} + \dfrac{{6y}}{{1000}}\] |
| \[(800,0)\] | \[z = \dfrac{{4 \times 800}}{{1000}} + \dfrac{{6 \times 0}}{{1000}} = \dfrac{{32}}{{10}} = 3.2\] |
| \[(400,200)\] | \[z = \dfrac{{4 \times 400}}{{1000}} + \dfrac{{6 \times 200}}{{1000}} = 1.6 + 1.2 = 2.8\] |
| \[(0,1000)\] | \[z = \dfrac{{4 \times 0}}{{1000}} + \dfrac{{6 \times 1000}}{{1000}} = 6\] |
Clearly, Z is minimum for \[x = 400\]grams of wheat and \[y = 200\] grams of rice. The minimum cost is Rs.\[2.8\]
Note: All we did in above is converting the given word problem into inequality problems. As we know that we need a point to join a line, hence we find the inequality value at ‘x’ is zero and then at ‘y’ is zero. Also if they mention minimum we take greater than or equal to(\[ \geqslant \]). If they mention maximum we take less than or equal to (\[ \leqslant \]).
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Why Are Noble Gases NonReactive class 11 chemistry CBSE

Let X and Y be the sets of all positive divisors of class 11 maths CBSE

Let x and y be 2 real numbers which satisfy the equations class 11 maths CBSE

Let x 4log 2sqrt 9k 1 + 7 and y dfrac132log 2sqrt5 class 11 maths CBSE

Let x22ax+b20 and x22bx+a20 be two equations Then the class 11 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

At which age domestication of animals started A Neolithic class 11 social science CBSE

Which are the Top 10 Largest Countries of the World?

Give 10 examples for herbs , shrubs , climbers , creepers

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Difference Between Plant Cell and Animal Cell

Write a letter to the principal requesting him to grant class 10 english CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Fill in the blanks A 1 lakh ten thousand B 1 million class 9 maths CBSE



