Question

How do you draw the graph for \[y=-cotx\] for \[0\le x\le 2\pi \] ?

Answer 1

Hint: First of all, we will see in which quadrant value of cotangent is positive and in which quadrant, it is negative. Then we can just reverse the quadrants for \[-cot\text{ }x\] to check where positive values lie and where negative. Then we will plot the x-y graph, where the x-axis will represent the angle in radians while the y-axis will denote the value of the function. The value of \[x\] lies between $0$ and $2\pi $ so the x-quadrant will contain an angle between them. The range of the function is \[cotx<-1\] or \[cotx>1\].
Then \[-cotx>1\] or \[-cotx\text{ }<-1\]. This implies \[y>1\] or \[y<1\].

Complete step by step solution:
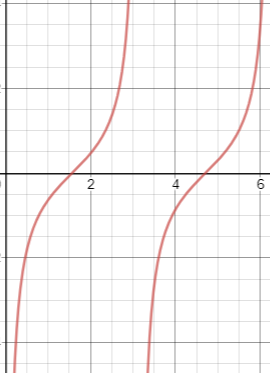
We have to plot the graph of \[-cot\text{ }x\]
For this we should have the value of \[cot\text{ }x\] in the given domain \[\left[ 0,2\pi \right]\].
We know that cot gives positive values in the first and fourth quadrant and negative values in the second and third quadrant.
So, -cot will give negative values in the first and fourth quadrant and positive values in the second and third quadrant.
The cycle changes after $\pi $ radians. So, there is one cycle between $0$ and $\pi $, another between $\pi $ and $2\pi $ and so on.
Every cycle has vertical asymptotes at the end of the cycle.
The value of cot in each cycle is decreasing so \[-cot\text{ }x\] will have increasing values in each cycle.
So , keeping all these points in mind , we plot the following graph:

Note:
The asymptotes of \[\text{cot }x\] are the x-intercepts of the function \[\text{tan }x\]. While the asymptotes of \[\text{tan }x\] is the x-intercept of the function \[\text{cot }x\].