
How do you calculate the mass of the sun,
Answer
478.2k+ views
Hint: Kepler discovered three empirical laws that accurately described the motion of the planets. The three laws may be states as,
(I) Planets move in an elliptical orbit, with the sun at one focus of the ellipse. This law is also known as the law of elliptical orbits and obviously gives the shape of the orbits of the planets around the sun.
(ii) The radius vector which is drawn from the sun to a planet, sweeps out equal areas in equal intervals of time, i.e. its areal velocity (or the area swept out by it per unit time) will remain constant. This law is also known as the law of areas and gives the relationship between the orbital speed of the planet and its distance from the sun.
(iii) The square of the time taken in one revolution of a planet is proportional to the cube of the semi-major axis of its orbit. This law is also known as the law of time period and gives the relationship between the size of the orbit of a planet and the time period of revolution.
Complete step by step answer:
To calculate the required expression for Kepler’s third law for planetary motion:
i.e.
The necessary condition that planets revolve around the sun is that- the centripetal force to the planet is being provided by the gravitational force exerted by the earth on the planet.
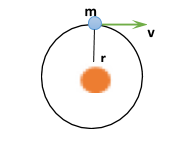
Thus,
Where,
-m is the mass of the planet.
-
-r is the size of orbit.
-v is speed or the orbital speed of the planet.
On solving the equation (i), we get
Now, calculation for the expression of time period (T)
Since,
On substituting the value of v from equation (ii), in the above expression, We get
On squaring both sides in equation (iii), we get
Note:
The derivation of Kepler's third law is one of the special cases of the circular orbits
(I) Planets move in an elliptical orbit, with the sun at one focus of the ellipse. This law is also known as the law of elliptical orbits and obviously gives the shape of the orbits of the planets around the sun.
(ii) The radius vector which is drawn from the sun to a planet, sweeps out equal areas in equal intervals of time, i.e. its areal velocity (or the area swept out by it per unit time) will remain constant. This law is also known as the law of areas and gives the relationship between the orbital speed of the planet and its distance from the sun.
(iii) The square of the time taken in one revolution of a planet is proportional to the cube of the semi-major axis of its orbit. This law is also known as the law of time period and gives the relationship between the size of the orbit of a planet and the time period of revolution.
Complete step by step answer:
To calculate the required expression for Kepler’s third law for planetary motion:
i.e.
The necessary condition that planets revolve around the sun is that- the centripetal force to the planet is being provided by the gravitational force exerted by the earth on the planet.

Thus,
Where,
-m is the mass of the planet.
-
-r is the size of orbit.
-v is speed or the orbital speed of the planet.
On solving the equation (i), we get
Now, calculation for the expression of time period (T)
Since,
On substituting the value of v from equation (ii), in the above expression, We get
On squaring both sides in equation (iii), we get
Note:
The derivation of Kepler's third law is one of the special cases of the circular orbits
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

An example of ex situ conservation is a Sacred grove class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




