Question

Are triangles formed in each figure similar? If so, name the criterion of similarity. Write the similarity relation in symbolic form.
i)

ii)

iii)

iv)

v)

vi)

vii)

viii)

Answer 1

Hint: The main similarity rules of triangles are AAA, SAS, SSS and RHS. Remember that corresponding sides of similar polygons are in proportion, and corresponding angles of similar polygons have the same measure. Use this to check corresponding angles and sides in all the pair of triangles given in all eight-figures.

Complete step-by-step answer:
In the very beginning, we have defined Mohit’s son’s present age as $x$ years. We can take Mohit’s Here In the question, we are given eight diagrams of triangles in pairs. And we need to check whether they are similar triangles or not. We will use the triangle similarity rules for that.
Before we start with the solution, we first need to understand the concept of similarity of triangles. If two angles of a triangle have measures equal to the measures of two angles of another triangle, then the triangles are similar. Corresponding sides of similar polygons are in proportion, and corresponding angles of similar polygons have the same measure.
There are four similarity tests for triangles.
Angle-Angle-Angle (AAA): If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar.
It is sufficient to prove that only two pairs of angles are respectively equal to each other. Because the angle sum of a triangle is always $180^\circ $, the third pair of angles will automatically be equal. Re-stating this fact is not required when using the AAA test in a similarity proof.
Side- Angle-Side (SAS): If the ratio of the lengths of two sides of one triangle is equal to the ratio of the lengths of two sides of another triangle, and the included angles are equal, then the two triangles are similar.
Side-Side-Side (SSS): If each of the sides of one triangle can be matched up with each of the sides of another so that the ratios of matching sides are equal, then the two triangles are similar.
Right-angle-Hypotenuse-Side (RHS): If the ratio of the hypotenuse and one side of a right-angled triangle is equal to the ratio of the hypotenuse and one side of another right-angled triangle, then the two triangles are similar.
Let's start with the figure (i),
Here side FG and IK are parallel. Also $\angle FHG = \angle IHK$ , since they are opposite angles.
And $\angle GFH$ and $\angle IKH$ are alternate angles for the transverse line FK, and similarly $\angle FGH$ and $HIK$ are alternate angles for the transverse GI between parallel sides FG and IK.
$ \Rightarrow \angle GFH = \angle IKH{\text{ and }}\angle FGH = \angle HIK$
So, in this case, we have all the three angles equal for the two triangles.
Thus, by the AAA similarity rule, we can say that $\Delta FHG \sim \Delta IHK$
Now, let’s consider figure (ii),
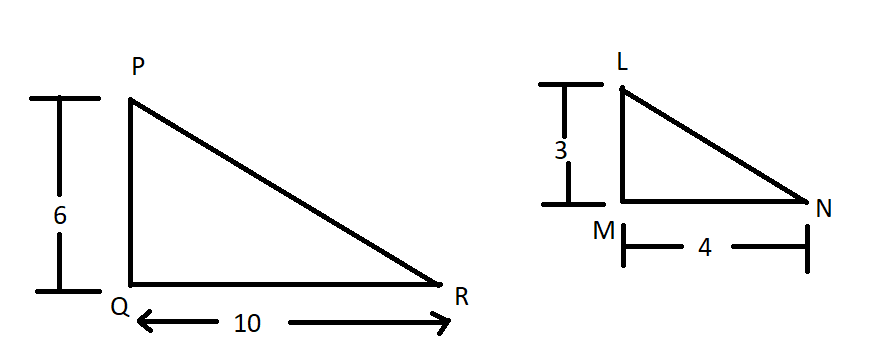
Here we have two triangles named $\Delta PQR{\text{ and }}\Delta LMN$. The measures of sides PQ, QR, LM and MN are given.
So, we know that, for triangles to be similar, their corresponding sides should be in the same ratio
$ \Rightarrow \dfrac{{PQ}}{{LM}} = \dfrac{6}{3} = 2{\text{ and }}\dfrac{{QR}}{{MN}} = \dfrac{{10}}{4} = \dfrac{5}{2} = 2.5 \ne 2$
Since here the ratios of the corresponding sides are not equal.
Therefore, the triangle $\Delta PQR{\text{ and }}\Delta LMN$ are not similar triangles.
Now, let’s consider figure (iii),
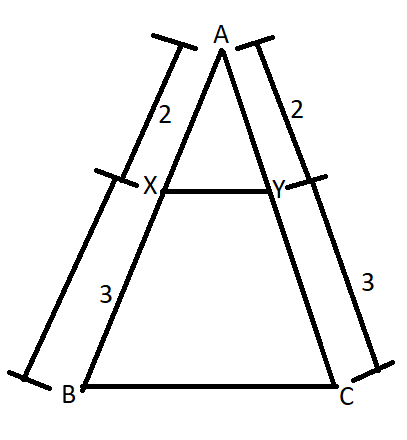
Here we have two triangles $\Delta AXY{\text{ and }}\Delta ABC$, sharing a common vertex and angle A. The measure of sides $AX = 2$ , $AY = 2$ , $AB = 3 + 2 = 5$ and $AC = 3 + 2 = 5$ are given to us.
Let’s check for the ratio corresponding sides in this case:
$\dfrac{{AX}}{{AB}} = \dfrac{2}{{2 + 3}} = \dfrac{2}{5}{\text{ and }}\dfrac{{AY}}{{AC}} = \dfrac{2}{{2 + 3}} = \dfrac{2}{5}$
Therefore, we have a ratio of two corresponding sides equal for these triangles, i.e. $\dfrac{{AX}}{{AB}} = \dfrac{{AY}}{{AC}} = \dfrac{2}{5}$ . And they share one angle $\angle A$ together formed by these pair of sides.
Thus, by the SAS similarity rule, we can say $\Delta AXY \sim \Delta ABC$
Now, we consider figure (iv),
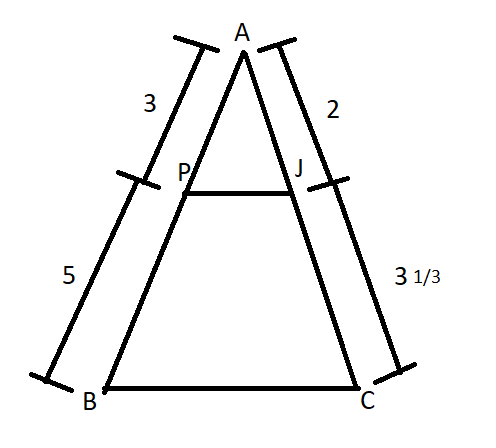
This case is similar to figure (iii) but there the measure of sides are different. Here we have two triangles $\Delta APJ{\text{ and }}\Delta ABC$ , sharing a common vertex and angle A. Measures of side AP, AB, AJ and AC is given
Checking ratio of corresponding sides, we get:
$ \Rightarrow \dfrac{{AP}}{{AB}} = \dfrac{3}{{3 + 5}} = \dfrac{3}{8}{\text{ and }}\dfrac{{AJ}}{{AC}} = \dfrac{2}{{2 + 3\dfrac{1}{3}}} = \dfrac{2}{{\dfrac{{6 + 10}}{3}}} = \dfrac{{2 \times 3}}{{16}} = \dfrac{3}{8}$
Therefore, we have a ratio of two corresponding sides equal for these triangles, i.e. $\dfrac{{AP}}{{AB}} = \dfrac{{AJ}}{{AC}} = \dfrac{3}{8}$. And they already share one angle $\angle A$together, formed in between these pair of sides.
Thus, by the SAS similarity rule, we can say that $\Delta APJ \sim \Delta ABC$
Now, let’s consider figure (v),
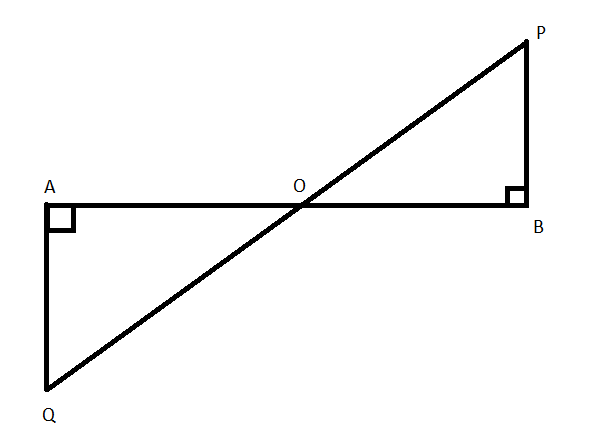
Here we have two right-angled triangles $\Delta OAQ{\text{ and }}\Delta OBP$ . They also have a pair of opposite angles, which therefore will be equal, i.e. $\angle AOQ = \angle BOP$ .
Therefore, we got two pairs of equal angles in $\Delta OAQ{\text{ and }}\Delta OBP$
Thus, by the AAA or AA similarity rule, we can say that $\Delta OAQ \sim \Delta OBP$
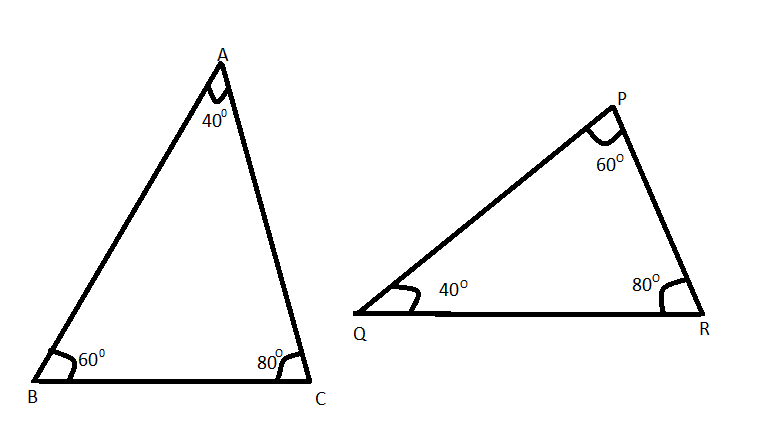
Now, let’s consider figure (vi),
Here we have two triangles $\Delta ABC{\text{ and }}\Delta PQR$ , in which the measures of all the angles are given.
In $\Delta ABC{\text{ and }}\Delta PQR$, $\angle B = \angle P = 60^\circ $ , $\angle C = \angle R = 80^\circ $ and $\angle A = \angle Q = 40^\circ $
Therefore, we have all the angles equal in the triangle $\Delta ABC{\text{ and }}\Delta PQR$
Thus, by using the AAA similarity rule, we can say $\Delta ABC \sim \Delta PQR$
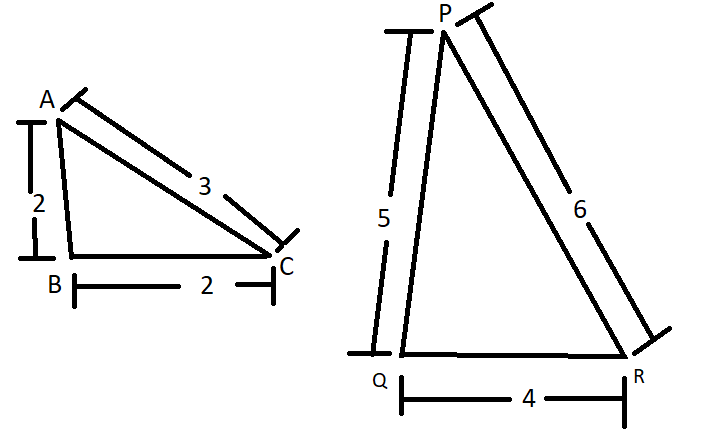
Now, let’s consider figure (vii),
Here we have two triangles $\Delta ABC{\text{ and }}\Delta PQR$ , in which the measures of each side are given.
Checking for the ratio of corresponding sides in both triangles:
$\dfrac{{AB}}{{PQ}} = \dfrac{2}{5} = 0.4$ , $\dfrac{{BC}}{{QR}} = \dfrac{2}{4} = 0.5$ and $\dfrac{{AC}}{{PR}} = \dfrac{3}{6} = 0.5$
Therefore, the ratio of the corresponding side is not equal
Thus, we can say that $\Delta ABC{\text{ and }}\Delta PQR$ are not similar
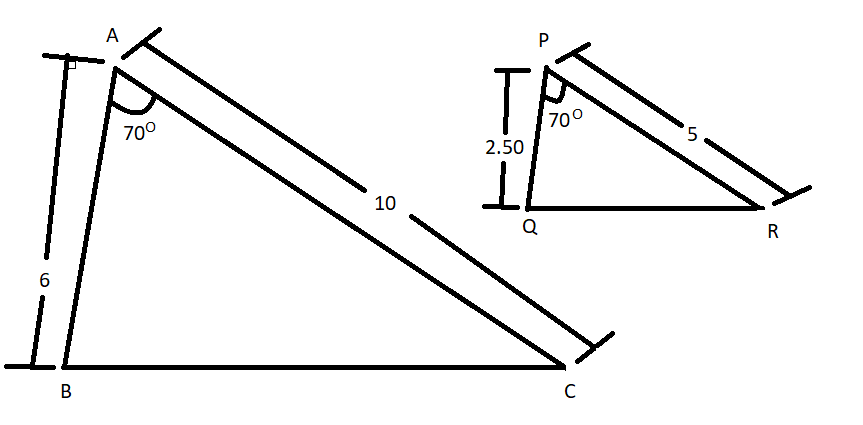
Now, let’s consider figure (viii),
Here we have two triangles $\Delta ABC{\text{ and }}\Delta PQR$ , in which measures of sides AB, AC, PQ and PR are given. Also the measure of angle $\angle BAC = \angle QPR = 70^\circ $
Checking the ratio of corresponding sides in both triangles, we get:
$\dfrac{{AB}}{{PQ}} = \dfrac{6}{{2.5}} = \dfrac{{12}}{5}{\text{ and }}\dfrac{{AC}}{{PR}} = \dfrac{{10}}{5} = 2$
Therefore, the ratio of corresponding sides in the triangle is not equal.
Thus, we can conclude that these triangles are not similar.

Note: In similarity of triangles, the symbol $' \sim '$ represents a similar triangle. Notice that in figure (v) we used two equal angles in both triangles to prove the similarity. As the sum of three internal angles in a triangle is $180^\circ $. Thus, we can say if two corresponding angles are equal in a pair of triangles then the third angle will also be equal. So, A-A-A rule can also be used as A-A similarity rule. Also, note that if a triangle is congruent then it will necessarily be similar, but vice-versa is not true.