
An α−particle and a proton moving with the same speed enter the same magnetic field region at right angles to the direction of the field. Show the trajectories followed by the two particles in the region of the magnetic field. Find the ratio of the radii of the circular paths which the two particles may describe,


Answer
503.7k+ views
Hint:Here we have two charged particles one is proton and the other is an alpha particle and they both are moving in a straight line towards the positive x-axis. They enter a region of magnetic field perpendicularly and we need to fight the trajectory since they both are charged they will be acted upon by the magnetic force
Complete step by step answer:
The magnetic force on a charged particle placed in a magnetic field is given by
The angle between the velocity vector and the magnetic field is
The direction is given as
Since we are interested in finding the direction, taking only unit vectors we get,
So the force acts towards the positive y-axis that is in an upward direction for both the particles. Now let us find the magnitude for both of them. Since both have the same velocity and the value of the magnetic field is the same for both, F=qvB,
Force is directly proportional to the magnitude of charge and the charge on the alpha particle is twice the value of charge on the proton, so the force acting on the alpha particle will be two times in magnitude the force acting on the proton.
So the trajectory looks like,
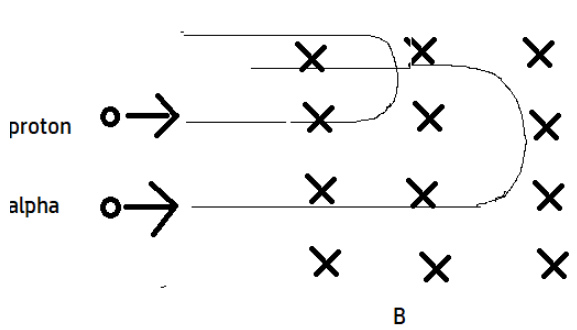
Note:Proton has a charge q and the charge on the alpha particle which is a doubly ionized helium atom is 2q. Both are positive and when they enter the magnetic field, they experience the force. If the angle between the velocity vector and the magnetic field was zero, then both of them would have passed undeflected.
Complete step by step answer:
The magnetic force on a charged particle placed in a magnetic field is given by
The angle between the velocity vector and the magnetic field is
The direction is given as
Since we are interested in finding the direction, taking only unit vectors we get,
So the force acts towards the positive y-axis that is in an upward direction for both the particles. Now let us find the magnitude for both of them. Since both have the same velocity and the value of the magnetic field is the same for both, F=qvB,
Force is directly proportional to the magnitude of charge and the charge on the alpha particle is twice the value of charge on the proton, so the force acting on the alpha particle will be two times in magnitude the force acting on the proton.
So the trajectory looks like,

Note:Proton has a charge q and the charge on the alpha particle which is a doubly ionized helium atom is 2q. Both are positive and when they enter the magnetic field, they experience the force. If the angle between the velocity vector and the magnetic field was zero, then both of them would have passed undeflected.
Latest Vedantu courses for you
Grade 10 | ICSE | SCHOOL | English
Vedantu ICSE 10 Pro Course (2025-26)
School Full course for ICSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

An example of ex situ conservation is a Sacred grove class 12 biology CBSE

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




