Answer
405.3k+ views
Hint: When some amount of work is done against the conservative force then the work done will be stored in the system as the potential energy as energy can neither be created nor destroyed. Along the direction of conservative force then potential energy of the system decreases. By using these two principles we will solve the question.
Formula used:
$\eqalign{
& P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr
& P.E = \dfrac{{ - GMm}}{r} \cr
& K.E = \dfrac{1}{2}m{v^2} \cr} $
Complete answer:
Since the mass is only acted upon the gravitational force, and gravitational force is conservative in nature, according to conservation of mechanical energy, total mechanical energy of the system is always conserved as long as only conservative forces acting on it.
That means change in mechanical energy is zero and mechanical energy(ME) will be constant always. Mechanical energy is the sum of potential and kinetic energy
$\eqalign{
& ME = {\text{ constant}} \cr
& \Rightarrow KE + PE = {\text{constant}} \cr
& \therefore P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr} $
Now due to gravitational force acting, the potential of the mass(m) due to gravitational force of attraction between shell of mass(M) and when distance between them is ‘r’ is given by
$P.E = \dfrac{{ - GMm}}{r}$ where ‘G’ is gravitational constant
Kinetic energy of mass m is given by
$K.E = \dfrac{1}{2}m{v^2}$
Where ‘m’ is the mass of the block and ‘v’ is the velocity of the block.
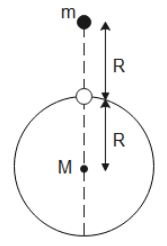
From the diagram below we will conserve energy between initial point and surface point to get velocity of mass ‘m’ at the shell surface.
Here point at height ‘R’ from the surface is initial point and surface point is final point
$\eqalign{
& P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr
& \Rightarrow \dfrac{{ - GMm}}{{2R}} + \dfrac{1}{2}m{v^2} = \dfrac{{ - GMm}}{R} \cr
& \therefore v = \sqrt {\dfrac{{GM}}{R}} \cr} $
Now this velocity will be constant till mass reaches a diametrically opposite point because there will be no force acting on it inside the shell. So the time taken will be
$\eqalign{
& s = vt \cr
& \Rightarrow 2R = vt \cr
& \Rightarrow \dfrac{{2R}}{v} = t \cr
& \therefore t = 2\sqrt {\dfrac{{{R^3}}}{{GM}}} \cr} $
Hence the time taken will be $2\sqrt {\dfrac{{{R^3}}}{{GM}}} $ to reach the opposite end.
Note:
Inside the shell there will be no mass. When there is no mass then there will be no gravitational field produced. If we consider a uniform sphere where mass is uniformly distributed throughout the volume and we make a hole exactly at the center then if we take any point in that cavity, the gravitational field will be zero.
Formula used:
$\eqalign{
& P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr
& P.E = \dfrac{{ - GMm}}{r} \cr
& K.E = \dfrac{1}{2}m{v^2} \cr} $
Complete answer:
Since the mass is only acted upon the gravitational force, and gravitational force is conservative in nature, according to conservation of mechanical energy, total mechanical energy of the system is always conserved as long as only conservative forces acting on it.
That means change in mechanical energy is zero and mechanical energy(ME) will be constant always. Mechanical energy is the sum of potential and kinetic energy
$\eqalign{
& ME = {\text{ constant}} \cr
& \Rightarrow KE + PE = {\text{constant}} \cr
& \therefore P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr} $
Now due to gravitational force acting, the potential of the mass(m) due to gravitational force of attraction between shell of mass(M) and when distance between them is ‘r’ is given by
$P.E = \dfrac{{ - GMm}}{r}$ where ‘G’ is gravitational constant
Kinetic energy of mass m is given by
$K.E = \dfrac{1}{2}m{v^2}$
Where ‘m’ is the mass of the block and ‘v’ is the velocity of the block.
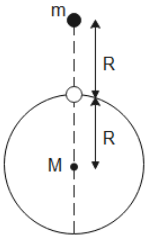
From the diagram below we will conserve energy between initial point and surface point to get velocity of mass ‘m’ at the shell surface.

Here point at height ‘R’ from the surface is initial point and surface point is final point
$\eqalign{
& P.{E_i} + K.{E_i} = P.{E_f} + K.{E_f} \cr
& \Rightarrow \dfrac{{ - GMm}}{{2R}} + \dfrac{1}{2}m{v^2} = \dfrac{{ - GMm}}{R} \cr
& \therefore v = \sqrt {\dfrac{{GM}}{R}} \cr} $
Now this velocity will be constant till mass reaches a diametrically opposite point because there will be no force acting on it inside the shell. So the time taken will be
$\eqalign{
& s = vt \cr
& \Rightarrow 2R = vt \cr
& \Rightarrow \dfrac{{2R}}{v} = t \cr
& \therefore t = 2\sqrt {\dfrac{{{R^3}}}{{GM}}} \cr} $
Hence the time taken will be $2\sqrt {\dfrac{{{R^3}}}{{GM}}} $ to reach the opposite end.
Note:
Inside the shell there will be no mass. When there is no mass then there will be no gravitational field produced. If we consider a uniform sphere where mass is uniformly distributed throughout the volume and we make a hole exactly at the center then if we take any point in that cavity, the gravitational field will be zero.
Recently Updated Pages
How many sigma and pi bonds are present in HCequiv class 11 chemistry CBSE

Why Are Noble Gases NonReactive class 11 chemistry CBSE

Let X and Y be the sets of all positive divisors of class 11 maths CBSE

Let x and y be 2 real numbers which satisfy the equations class 11 maths CBSE

Let x 4log 2sqrt 9k 1 + 7 and y dfrac132log 2sqrt5 class 11 maths CBSE

Let x22ax+b20 and x22bx+a20 be two equations Then the class 11 maths CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

Write a letter to the principal requesting him to grant class 10 english CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

Fill in the blanks A 1 lakh ten thousand B 1 million class 9 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Difference Between Plant Cell and Animal Cell

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE