Question

A square loop of side 5cm enters a magnetic field with \[1cm{{s}^{-1}}\]. The front edge enters the magnetic field at \[t=0\] then which graph best depicts emf induced?
                                  

Answer 1

Hint: A moving coil of conductor in a magnetic field is induced by a motional electromotive force according to Faraday's law of electromagnetic induction and Lenz’s law. The force on the loop is determined by the Lorentz force.

Complete answer:
We know that a moving charge in a magnetic field experiences a Lorentz force resulting in the change of the particle’s path. Similarly, a conductor is a large group of electrons which in a magnetic field experiences force. In addition to the force experienced, an electromotive force is induced in the conductor. This is due to the change in the electric flux. The reason for change in electric flux will be due to various reasons.
Let us consider the magnetic flux due to a closed loop,
\[\phi =BA\cos \theta \]
The magnetic flux is dependent on the magnetic field B, the area of loop A and the angle between the magnetic field B and area of the loop A.
From this, we can understand that any change in magnetic field or area can change the flux.
Now, let us consider the Faraday’s law of electromagnetic induction,
\[\varepsilon =-\dfrac{d\phi }{dt}\]
From this condition we can understand that an electromotive force is induced whenever there is a change in the magnetic flux.
So, we can conclude that any change in the area of the loop, the orientation in the system or a change in the magnetic field can induce an emf in the loop.
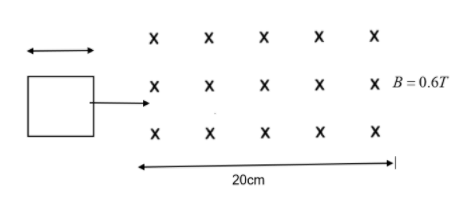
We consider the square loop entering the magnetic field,
A) As the square loop moves into the field, there is a steady rate of change of area inside the field, i.e., there is a change in the area of the loop, thus an emf is induced.
B) The rate of change of area is constant as the square loop moves in with a constant velocity, i.e., the emf remains constant as long as the square is entering the loop.
C) Now, Once the square has completely entered the field, there are no further changes in area or orientation, i.e., the emf reduces to zero.
D) The above conditions A) and B) repeats at the time when the square loop gets out of the loop also.
Now, let us draw the graph using all these factors:
                                 

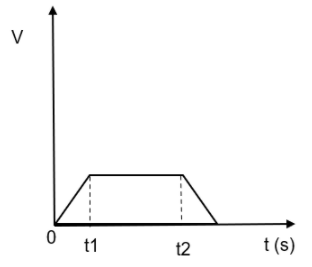
So, this is the required graph.

Additional Information:
We can calculate the force on the loop due to this magnetic flux change using the Lorentz force.

Note:
The emf produced in this case is due to the motion of the loop with respect to the magnetic field and it is a motional electromotive force that is induced in the loop. In the same an emf can be generated in the loop with a stationary loop in a changing magnetic field. The force in such a case will be due to Electric field alone as the velocity term in the Lorentz force turns out to be zero.