Question

A conical tank (with vertex down) is \[10\] feet across the top and \[12\] feet deep. Water is flowing into the tank at a rate of \[5\] cubic feet per minute. Find the rate of change of the depth of the water when the water is \[4\] feet deep.

Answer 1

Hint: In this question,we need to find the rate of change of the depth of the water when the water is \[4\] feet deep. Given that the radius of the tank is \[10\] feet. From this, we can find the radius of the tank. Also the height of the tank is \[12\] feet and the water is \[8\] feet deep. The rate of change of the depth of the water can be found by differentiating the volume of the cone. The differentiation is nothing but a rate of change of function with respect to an independent variable given in the function. First let us consider the radius of the tank to be \[r\] and \[h\] be the height of the tank . We need to substitute the value of \[r\] in the volume formula. Then on differentiating we can find the rate of change of the depth of the water when the water is \[4\] feet deep.

Formula used:
The volume of the cone ,
\[V = \dfrac{1}{3}\ \pi r^{2}\ h\]
Where \[r\] is the radius of the cone and \[h\] is the height of the cone.
Derivative rule used :
\[\dfrac{d}{dx}x^{n} = nx^{n – 1}\]

Complete step-by-step answer:

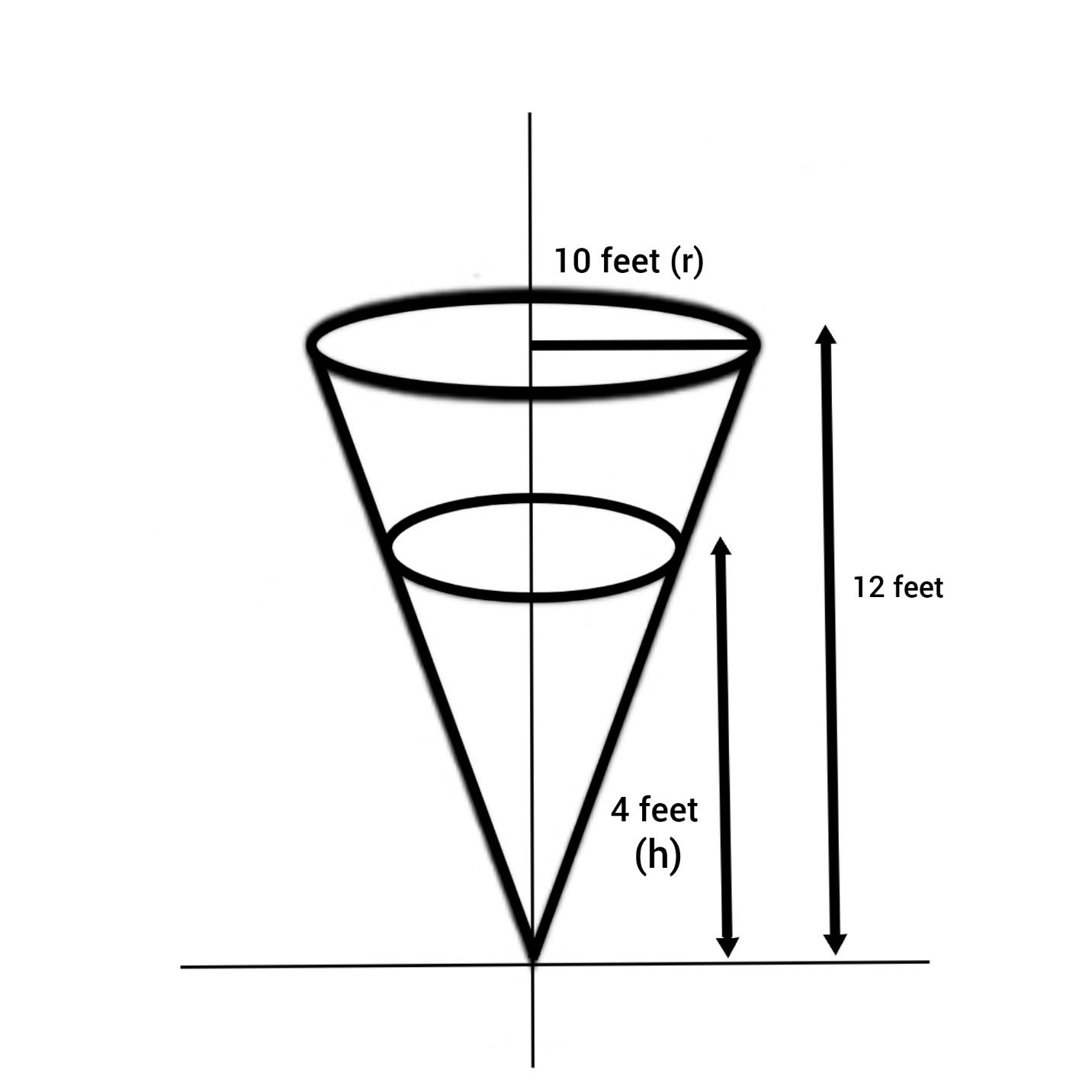
Let us consider the radius of the tank to be \[r\] and \[h\] be the height of the tank .
Given, the conical tank is \[10\] feet across and \[12\] feet deep, that is the radius of the tank is \[10\] feet and height is \[12\] feet.
\[r = 10\]
By relating the radius \[r\] to the height \[h\] ,
\[\Rightarrow \dfrac{r}{h} = \dfrac{10}{12}\]
Thus \[r = \dfrac{5}{6}h\]
We know volume of the cone is \[\dfrac{1}{3}\pi r^{2}h\]
\[V = \dfrac{1}{3}\pi r^{2}h\]
By substituting \[r = \dfrac{5}{6}h\]
We get,
\[V = \dfrac{1}{3}\pi{(\dfrac{5}{6}h)}^{2}h\]
On simplifying,
We get,
\[V = \dfrac{25}{108}\pi h^{3}\]
On differentiating \[V\] with respect to time \[t\] ,
We get,
\[\dfrac{dV}{dt} = \dfrac{25}{108}\pi\left( 3h^{2}\dfrac{dh}{dt} \right)\]
Also given the rate change of volume is \[5\] cubic feet per minute.
By substituting the value of volume rate,
We get,
\[5 = \dfrac{25}{108}\pi\left( 3h^{2}\dfrac{dh}{dt} \right)\]
We need to find the rate of change of the depth of the water when the water is \[4\] feet deep,
By substituting the value of \[h = 4\] and \[\pi = 3.14\] ,
We get,
\[5 = \dfrac{25}{108}\left( 3.14 \right)\left( 3\left( 4 \right)^{2}\dfrac{dh}{dt} \right)\]
On simplifying,
We get,
\[5 = 0.719\left( (48)\dfrac{dh}{dx} \right)\]
On multiplying the term inside,
We get,
\[5 = 34.5\dfrac{dh}{dt}\]
By rewriting the terms,
We get,
\[\dfrac{dh}{dx} = \dfrac{5}{34.5}\]
On simplifying,
We get \[\dfrac{dh}{dt} \approx 0.146\] feet/min
Thus we get the rate of change of the depth of the water is approximately \[0.146\] feet/min.
Final answer :
The rate of change of the depth of the water is approximately \[0.146\] feet/min.

Note: Mathematically , Derivative helps in solving the problems in calculus and in differential equations. The derivative of \[y\] with respect to \[x\] is represented as \[\dfrac{dy}{dx}\] . Here the notation \[\dfrac{dy}{dx}\] is known as Leibniz's notation . In derivation, there are two types of derivative namely first order derivative and second order derivative. A simple example for a derivative is the derivative of \[x^{3}\] is \[3x\] . Derivative is applicable in trigonometric functions also .