Question

A ball of mass \[M\] is thrown upward if the air resistance is considered constant (\[R\]). What will be times of ascent and descent? Give mathematical proof.

Answer 1

Hint:Calculate the net acceleration of the ball in the presence of constant air resistance. Use the kinematic expressions relating displacement, initial velocity, final velocity, acceleration and time.

Formulae used:
The expression for Newton’s second law of motion is
\[\Rightarrow {F_{net}} = Ma\] …… (1)
Here, \[{F_{net}}\] is the net force on the object, \[M\] is the mass of the object and \[a\] is the acceleration of the object.
The kinematic expression relating initial velocity \[u\], final velocity \[v\], acceleration \[a\] and displacement \[s\] in a free fall is
\[\Rightarrow {v^2} = {u^2} - 2as\] …… (2)
The kinematic expression relating displacement \[s\], initial velocity \[u\], time \[t\] and acceleration \[a\] in a free fall is
\[\Rightarrow s = ut - \dfrac{1}{2}a{t^2}\] …… (3)

Complete step by step answer:
Calculate the time of ascent of the ball while going upward.Calculate the net acceleration \[{a_1}\] on the ball when thrown upward.

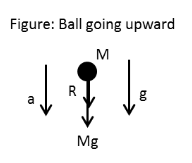
When the ball of mass \[M\] is going upward, the weight of the ball and the air resistance acts in downward direction.
Apply Newton’s second law to the ball.
\[ \Rightarrow - R - Mg = - M{a_1}\]
\[ \Rightarrow {a_1} = g + \dfrac{R}{M}\]
Hence, net acceleration on the ball going upward is \[g + \dfrac{R}{M}\].
The final velocity of the ball when it reaches its maximum height is zero.
Let \[{u_1}\] and \[{v_1}\] are the initial and final velocities of the ball while going upward and rewrite equation (2).
\[ \Rightarrow {v_1}^2 = {u_1}^2 - 2{a_1}s\]
Substitute \[g + \dfrac{R}{M}\] for \[{a_1}\] and \[0\,{\text{m/s}}\] for \[{v_1}\] in the above equation and rearrange it for the displacement \[s\] of the ball.
\[{\left( {0\,{\text{m/s}}} \right)^2} = u_1^2 - 2\left( {g + \dfrac{R}{M}} \right)s\] …… (4)
\[ \Rightarrow s = \dfrac{{u_1^2}}{{2\left( {g + \dfrac{R}{M}} \right)}}\]
Rewrite equation (3) for the displacement of the ball while going upward.
\[\Rightarrow s = {u_1}{t_a} - \dfrac{1}{2}{a_1}t_a^2\]
Here, \[{t_a}\] is the time of ascent of the ball.
Substitute \[g + \dfrac{R}{M}\] for \[{a_1}\]a in the above equation and rearrange it for \[{t_a}\].
\[\Rightarrow s = {u_1}{t_a} - \dfrac{1}{2}\left( {g + \dfrac{R}{M}} \right)t_a^2\]
\[ \Rightarrow \dfrac{1}{2}\left( {g + \dfrac{R}{M}} \right)t_a^2 - {u_1}{t_a} + s = 0\]
\[ \Rightarrow {t_a} = \dfrac{{{u_1} \pm \sqrt {u_1^2 - 2\left( {g + \dfrac{R}{M}} \right)s} }}{{g + \dfrac{R}{M}}}\]
Substitute \[0\] for \[u_1^2 - 2\left( {g + \dfrac{R}{M}} \right)s\] in the above equation.
\[ \Rightarrow {t_a} = \dfrac{{{u_1} \pm \sqrt 0 }}{{g + \dfrac{R}{M}}}\] …… (from equation (4))
\[ \Rightarrow {t_a} = \dfrac{{{u_1}}}{{g + \dfrac{R}{M}}}\]
Hence, the expression for time ascent the ball is \[\dfrac{{{u_1}}}{{g + \dfrac{R}{M}}}\].
Calculate the time of descent of the ball while going downward.
Calculate the net acceleration \[{a_2}\] on the ball when coming downward.

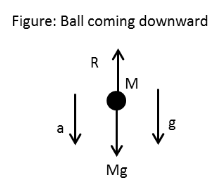
When the ball of mass \[M\] is coming downward, the weight of the ball acts in downward direction and the air resistance acts in the upward direction.
Apply Newton’s second law to the ball.
\[\Rightarrow R - Mg = - M{a_2}\]
\[ \Rightarrow {a_2} = g - \dfrac{R}{M}\]
Hence, net acceleration on the ball coming downward is \[g - \dfrac{R}{M}\].
The final velocity of the ball when it reaches the ground is zero.
Let \[{v_1}\] and \[{v_2}\] are the initial and final velocities of the ball while coming downward and rewrite equation (3).
\[\Rightarrow s = {v_1}{t_d} - \dfrac{1}{2}{a_2}t_d^2\]
Here, \[{t_d}\] is the time of descent of the ball.
Substitute \[g - \dfrac{R}{M}\] for \[{a_2}\] and \[0\,{\text{m/s}}\] for \[{v_1}\] in the above equation.
\[\Rightarrow s = \left( {0\,{\text{m/s}}} \right){t_d} - \dfrac{1}{2}\left( {g - \dfrac{R}{M}} \right)t_d^2\]
\[ \Rightarrow s = - \dfrac{1}{2}\left( {g - \dfrac{R}{M}} \right)t_d^2\]
The displacement of the ball while moving upward and coming downward is the same.
Substitute \[\dfrac{{u_1^2}}{{2\left( {g + \dfrac{R}{M}} \right)}}\] for \[s\] in the above equation and solve for \[{t_d}\].
\[ \Rightarrow \dfrac{{u_1^2}}{{2\left( {g + \dfrac{R}{M}} \right)}} = - \dfrac{1}{2}\left( {g - \dfrac{R}{M}} \right)t_d^2\]
\[ \Rightarrow u_1^2 = \left( {g - \dfrac{R}{M}} \right)\left( {g + \dfrac{R}{M}} \right)t_d^2\]
\[ \Rightarrow {t_d} = \dfrac{{u_1^2}}{{\left( {g - \dfrac{R}{M}} \right)\left( {g + \dfrac{R}{M}} \right)}}\]
\[ \Rightarrow {t_d} = \sqrt {\dfrac{{u_1^2}}{{{g^2} - {{\left( {\dfrac{R}{M}} \right)}^2}}}} \] \[\Rightarrow\because {a^2} - {b^2} = \left( {a + b} \right)\left( {a - b} \right)\]
\[ \Rightarrow {t_d} = \dfrac{{{u_1}}}{{\sqrt {{g^2} - {{\left( {\dfrac{R}{M}} \right)}^2}} }}\]
Hence, the expression for the time of descent of the ball is \[\dfrac{{{u_1}}}{{\sqrt {{g^2} - {{\left( {\dfrac{R}{M}} \right)}^2}} }}\].

Note: The time of ascent for the ball moving upward and the time of descent for the ball coming downward are different when a constant air resistance is considered and same when air resistance is neglected.