ICSE Class 10 Mathematics Chapter 17 Selina Concise Solutions - Free PDF Download
Updated ICSE Class 10 Mathematics Chapter 17 - Circle Selina Solutions are provided by Vedantu in a step-by-step method. Selina is the most famous publisher of ICSE textbooks. Studying these solutions by Selina Concise Mathematics Class 10 Solutions which are explained and solved by our subject matter experts will help you in preparing for ICSE exams. Concise Mathematics Class 10 ICSE Solutions can be easily downloaded in the given PDF format. These solutions for Class 10 ICSE will help you to score good marks in ICSE Exams 2024-25.
The updated solutions for Selina textbooks are created by the latest syllabus. These are provided by Vedantu in a chapter-wise manner to help the students get a thorough knowledge of all the fundamentals.






Access ICSE Selina Solutions for for Class 10 Mathematics Chapter 17 - Circles
Exercise-17A
Long Answer Type
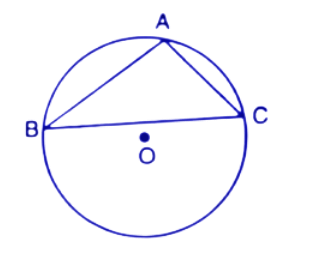
1. In the given figure O is the centre of the circle. ∠AOB and ∠OCB are
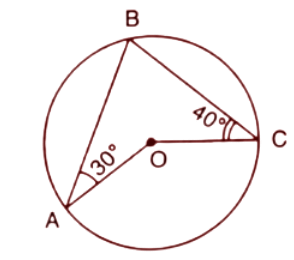
Ans: On joining the points A and C we get the triangles
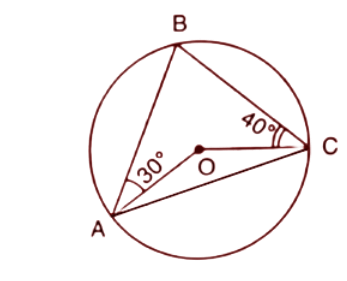
In
In
∠BAC=
We know that in a triangle sum of angles is
Since the angle at centre is double the angle at the circumference subtended by the same chord.
So from eq.1 the value of
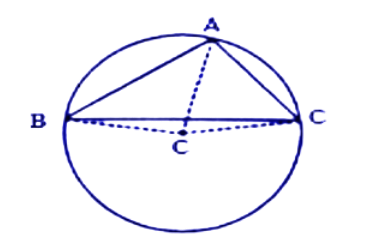
2. In the given figure,
(i). Prove that AC is diameter of the circle
Ans: In
Since,
Hence the triangle
(ii). Find
Ans: We know that angles in the same segments of circle are equal.
Hence,
3. Given O is the centre of the circle and
(i).
Ans: We know that the angle at the centre is double
the angle at the circumference subtended by the same chord.
So the value of
ACB and OAC are b/w the same line segments.
Hence
(ii).
Ans: ∵ OA=OC (radii of circle)
Thus the values
4. In each of the following figures, O is the centre of the circle. Find the values of a,b and c.
(i).
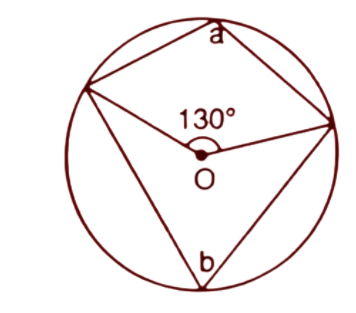
Ans: The angle at the centre is double the angle at the circumference subtended by the same chord.
By the theorem, the opposite angles of a cyclic quadrilateral are supplementary.
Hence
Thus the values of a and b are respectively
(ii)
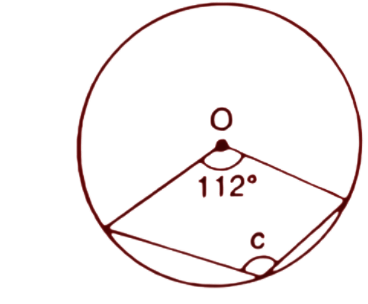
Ans: Given, central angle =
Reflex angle of
We know that the angle at the centre is double the angle at the circumference by the same arc.
Hence,
Thus the value of angle c is
5. In each of the following figures, O is the centre of the circle. Find the values of a,b,c and d.
(i).
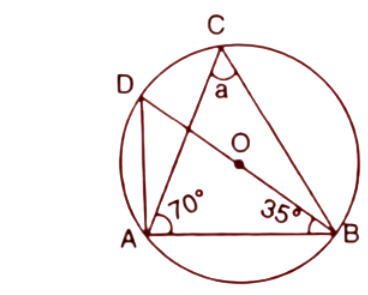
Ans: Here O is the centre of the circle and BD is the Diameter of the given circle and side of the triangle ABD as well.
We know the angle at the semicircle is a right angle triangle. Hence
The sum of angles in a triangle is
We know that angles in the same segment of circle are always equal.
Thus the value of a is
(ii).
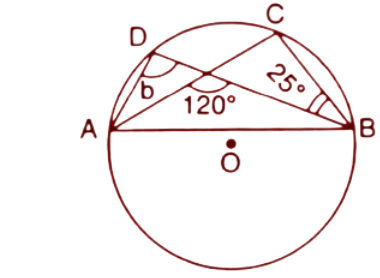
Ans: In a circle angles at the circumference subtended by the same chord are always equal. Angles
By the exterior angle property the exterior angle in a triangle is equal to the sum of opposite interior angles.
Thus the value of
(iii).
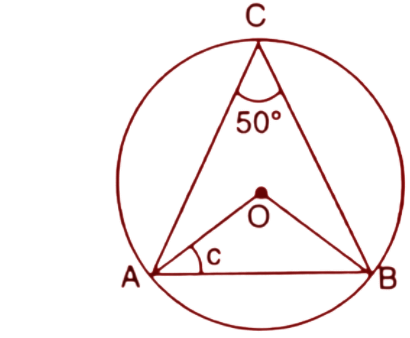
Ans: We know that the angle at the centre is double the angle at the circumference subtended by the same chord.
Also, OA=OB (Radii of the circle)
Hence the
So,
The sum of angles in a triangle is always
Thus the value of c is
(IV).
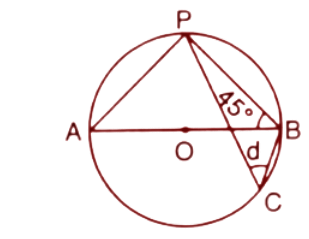
Ans: Given, angle
AB is the diameter of the circle hence
The sum of angles in a triangle is always
Now, the angles subtended by the same chord are equal. Here the angels
Hence
From eq. 1
Thus the value of angle d is
6. In the figure, AB is the common chord of the two circles. If AC and AD are diameters; prove that D,B and C are in a straight line.
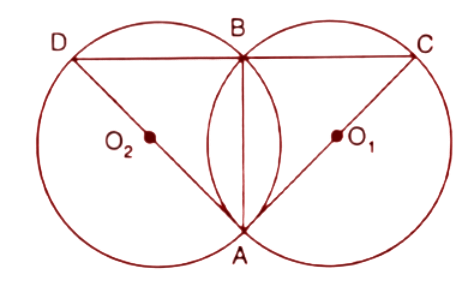
Ans:
Given,
Here AD and AC are the diameter of the circles. So the angles
Hence,
On Adding both the angles
Thus D,B and C form a straight line.
7. In the figure, given below, find:
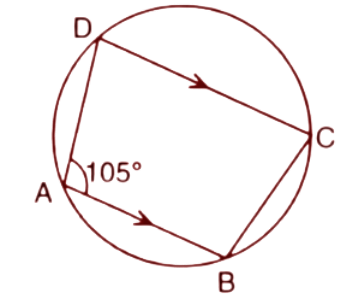
(ii).
(iii).
Show steps of your working.
(i).
Ans: In a cyclic quadrilateral the sum of
opposite angles are always
Given,
Now,
Thus the value of
(ii).
Ans: From the figure,
Thus the value of
(iii).
Ans: In a cyclic quadrilateral the sum of opposite angles is always
Thus the value of
8. In the given figure, O is the centre of the circle. If
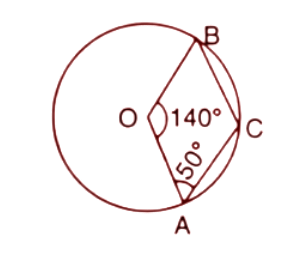
(i).
Ans: Given,
Reflex angle of
We know that the angle at the centre is double the angle at the circumference.
Reflex angle of
Thus the angle
(ii).
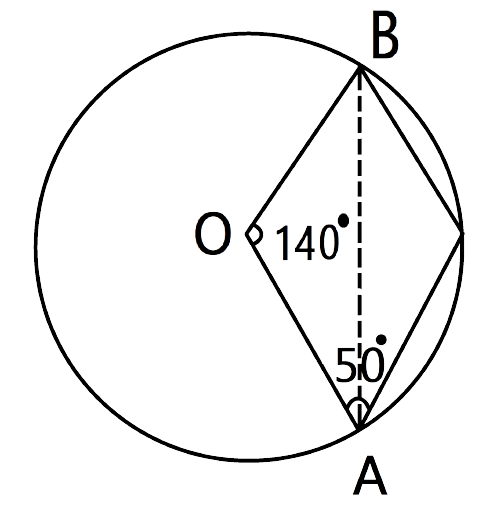
Ans: Since OA=OB (Radii of circle)
Hence
And we know that the sum of angles of a triangle
is always
So,
From eq. 1
∵
Só,
Thus the value of
(iii).
Ans: In
Since,
(iv).
Ans: In
From eq. 2 the value of
9. Calculate:
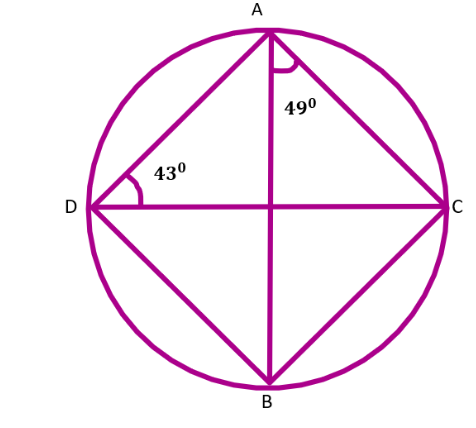
(i).
Ans: We know that the angle subtended by the same chord on the circle is equal.
Here in the given circle
Hence
So,
Thus the value of
(ii).
Ans: The angle subtended by the same chord on the circle is equal.
Here in the given circle
Hence
So,
Thus the value of
(iii).
Ans: By the angle sum property the sum of angles in a triangle is
In
Thus the value of
10. In the figure, given below, ABCD is a cyclic quadrilateral in which
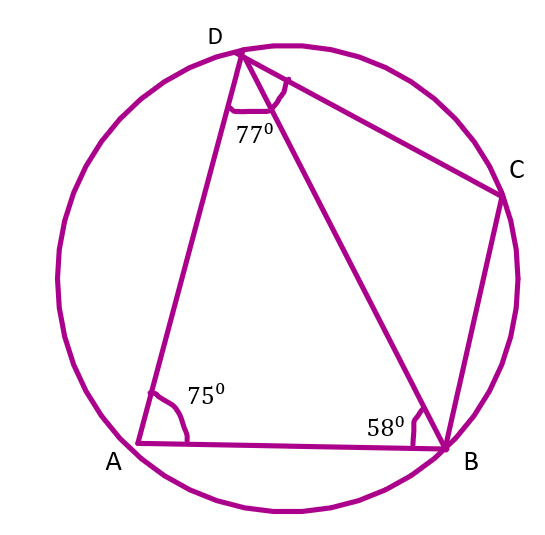
(i).
Ans: By the angle sum property we know that the sum
of all interior angles in a triangle is
In
Thus the value of
(ii).
Ans: By the property of cyclic quadrilaterals, the opposite angles of a cyclic quadrilateral are supplementary. Here
So,
Thus the value of
(iii).

Ans: Angles subtended by the same chord in the same segment of a circle are always equal. Here the angels
Hence,
Then,
Thus the value of
11. In the following figure, O is the centre of the circle and
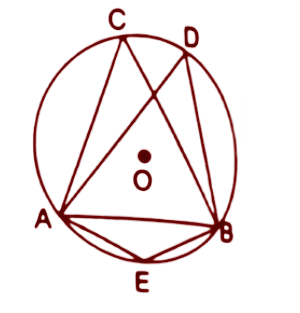
Find:
(i).
Ans: Given that
So all the angles will be equal.
So, In
We know that angles subtended by the same chord are always equal. Here
Hence,
Thus the value of
(ii).
Ans: Join OA and OB.
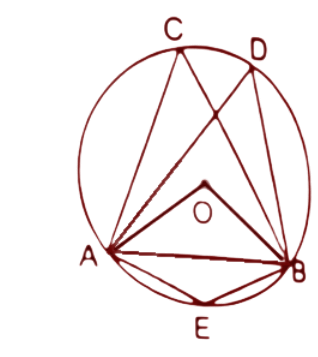
The angle at the centre is double the angle at the circumference of the circle by the same chord.
∴
Here the reflex angle of
Hence, Reflex angle of
Thus the value of
12. Given:
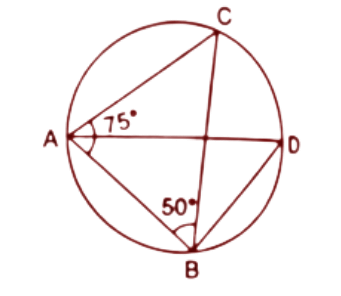
Ans: By the angle sum property the sum of angles in a triangle is always
In
The angle subtended by the same chord is always equal.
Here
Hence,
In
Therefore the value of
13. ABCD is a cyclic quadrilateral in a circle with centre O. If
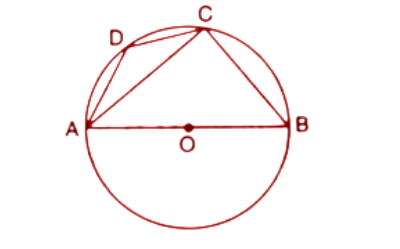
Ans: We know that the angle made in a semicircle is the right angle.
Here AB is a diameter and
So,
In a cyclic quadrilateral, opposite angles are supplementary.
So,
Now, By using angle sum property in
Thus the value of angle
14. In the figure, given alongside, AOB is a diameter of the circle and
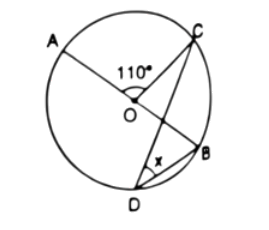
Ans: First we join points A and D.
In a circle the angle at the centre is double the angle at the circumference subtended by the same chord.
Now, Angle made in the semicircle is a right angle. Here angle
Therefore,
Since,
Thus the value of angle
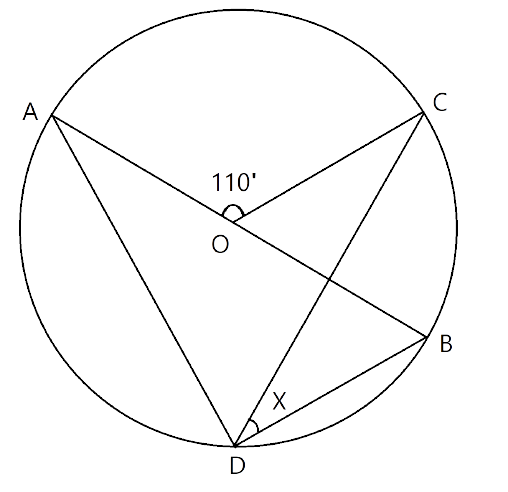
15. In the following figure, O is the centre of the circle,
Ans: In a circle the angle at the centre is double the angle at the circumference by the same chord. Here angle
Hence,
In
Thus the value of
16. In cyclic quadrilateral ABCD,

(i).
Ans: In a circle, Angles at the circumference subtended by the same chord are equal. Here the
Hence
So the value of
(ii).
Ans: In a circle, Angles at the circumference subtended by the same chord are equal.
From the figure,
From eq. 1 and eq.2
So the value of
(iii).
Ans: In a cyclic quadrilateral the sum of opposite angles is
Thus the value of
17. In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If
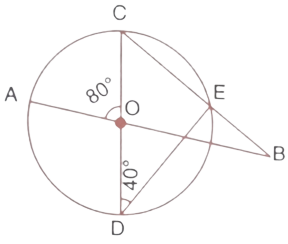
(i).
Ans: The angle made in a semicircle is the right angle. Here CD is the diameter so the angle
Now, By using the angle sum property in
Thus the value of
(ii).
Ans: We know that In a triangle the exterior angle is equal to the sum of a pair of opposite angles.
In
Since,
Thus the value of
18. In the given figure, AC is the diameter of a circle,whose centre is O. A circle is described on AO as diameter. AE, is a chord of the larger circle, intersects the smaller circle at B. Prove that AB=BE.
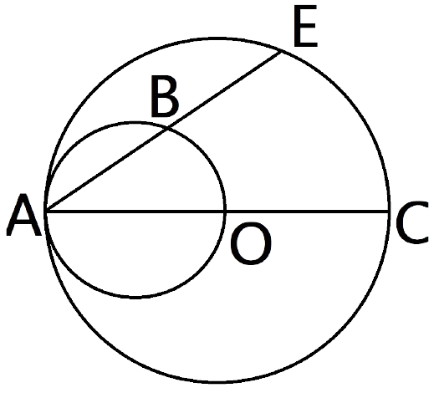
Ans: First we join points B and O.
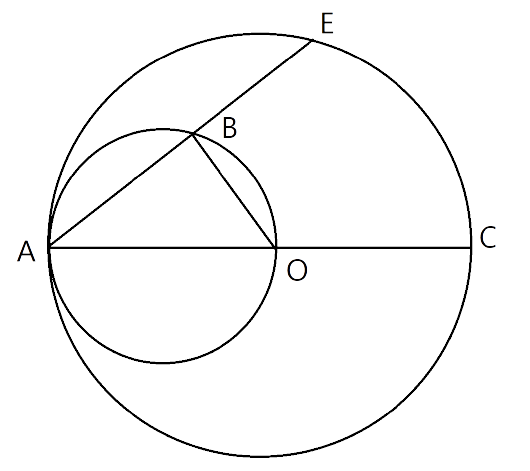
Now In triangle
So,
And we know that if we draw the perpendicular on the chord from the centre then the perpendicular bisects the chord.
Hence, AB=BE.
19. (a). In the following figure,
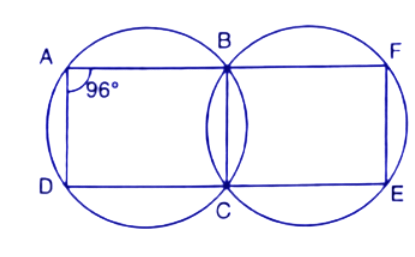
(i). If
Ans: ABCD is a cyclic quadrilateral and we know that the sum of opposite angles in a cyclic quadrilateral is
So,
Now,
Here BCEF is also a cyclic quadrilateral.
So,
Thus the value of
(ii). Prove that AD is parallel to FE.
Ans: ADEF is a cyclic quadrilateral
Here
Here
By converse of the co-interior angle theorem, if a transversal line intersects two lines in such a way that the sum of a pair of co-interior angles is
Hence, AD||EF.
(b). ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q. Show that quadrilateral PCDQ is cyclic.

Ans: ABPQ is cyclic quadrilateral.
From eq.(1)&(2)
Here ABCD is a parallelogram, So the sum of the co-interior angles on the same side is
Hence,
From eq.(3)
We know that if the sum of opposite angles of a quadrilateral is
Therefore PCDQ is a cyclic quadrilateral.
20. Prove that:
(i)The parallelogram inside the circle is a rectangle.
Ans:
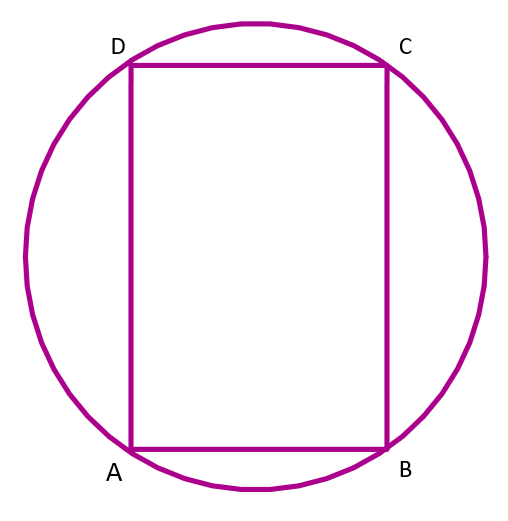
Let ABCD is a parallelogram inside the circle.
In parallelogram, Opposite sides and opposite angles are equal.
Therefore, AB=CD and
Since, ABCD is also a cyclic quadrilateral.
So,
Therefore,
Similarly, The other two angles will be right angles and the other pair of opposite sides are equal. Hence all the respective sides are equal.
AB=CD and BC=AD
So the parallelogram ABCD is a rectangle.
(ii). The rhombus, inscribed in a circle, is a square.
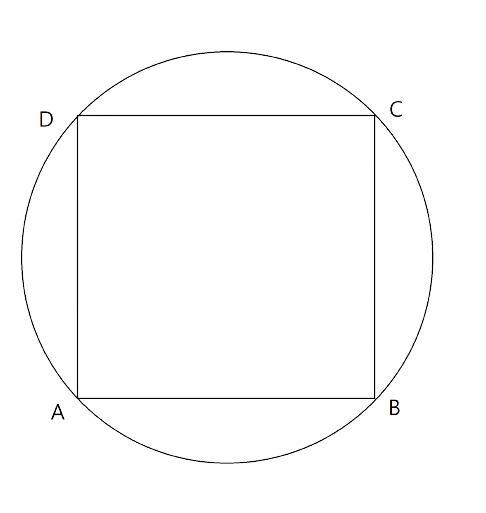
Ans: In a rhombus opposite angles are always equal.
Hence,
Here ABCD is also a cyclic quadrilateral.
So,
∴
Similarly the other two angles are right angle and equal.
So,
Therefore the quadrilateral ABCD is a square.
21. In the given figure, AB=AC. Prove that DECB is an isosceles trapezium.
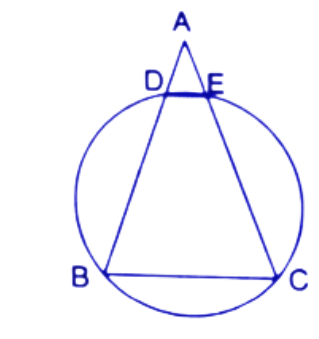
Ans: Given AB=AC------(1)
At first we join the points D and E.
∵ AB=AC
Therefore
Here DBEC is a cyclic quadrilateral.
So,
So, DE||BC and given AB=AC
∴
Hence,
Therefore the sides AD=AE-----(2)
From eq.(1)-eq.(2)
Therefore DECB is an isosceles trapezium.
22. Two circles intersect at P and Q. Through P diameter PA and PB of the two circles are drawn. Show that the points A, Q and B are collinear.
Ans:
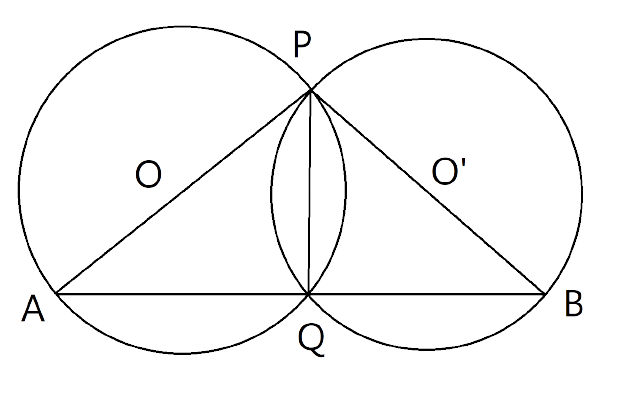
Let O and O’are the centres of the two intersecting circles and PA and PB are the diameters. Here Angles
So,
Now on adding the above angles,
Thus AQB is a straight line. Hence point A,Q and b are collinear points.
23. The figure given below, shows a circle with centre O.
Given:
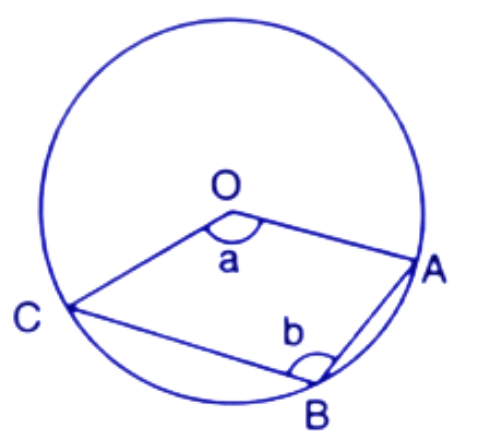
(i). Find the relationship between a and b.
Ans: We know that in a circle the angle at the centre is double the angle at any point on circumference subtended by the same chord.
So,
From the given figure,
Thus the relation b/w a and b is
(ii). Find the measure of angle OAB, if OABC is a parallelogram.
Ans: Since OABC is a parallelogram, therefore opposite angles are equal i.e.
a=b
From eq.1
Since OABC is a parallelogram, Therefore opposite sides are parallel and the sum of corresponding angles is
So, OC||AB
Thus the value of angle
24. Two chords AB and CD intersect at point P inside the circle. Prove that the sum of the angles subtended by the arcs AC and BD at the centre O is equal to twice the angle APC.
Ans:
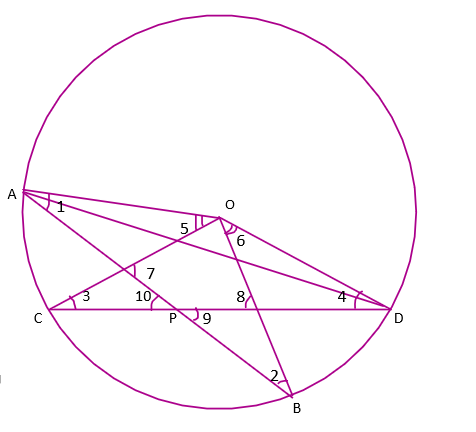
In a circle the angle at the centre is double the angle at any point on circumference subtended by the same chord.
Similarly,
On adding eq. (1)&(2)
By the exterior angle property, In a triangle the exterior angle is equal to the sum of the opposite angles.
Now in
By eq. (3) & (4)
Thus the sum of the angles subtended by the arcs AC and BD at the centre O is equal to twice the angle APC.
25. In the given figure, RS is the diameter of the circle. NM is parallel to RS and
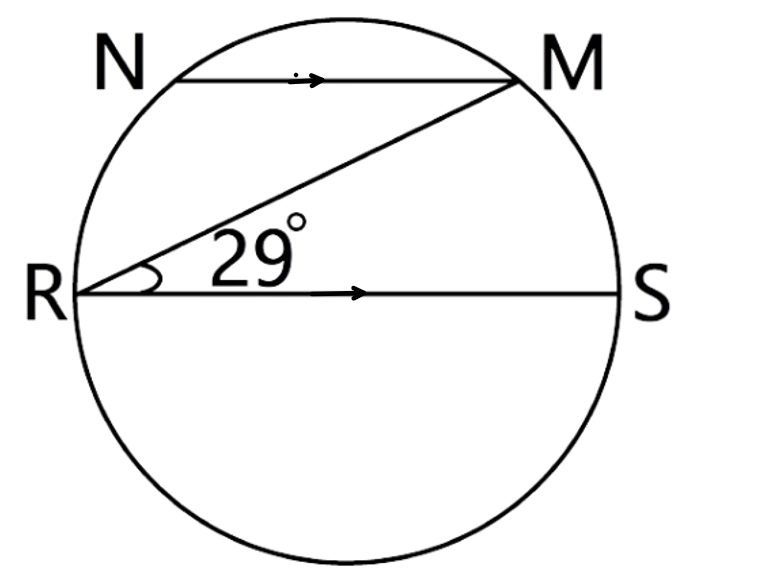
(i).
Ans: First we join the point N to R and M to S as
Here RS is the diameter and angle
So,
By the angle sum property, the sum of the interior angles in a triangle is
In
Since, NMRS is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
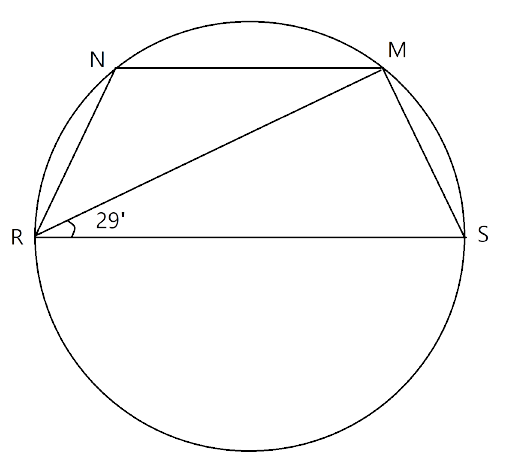
(ii).
Ans: Since, NM||RS (Given)
Thus,
In a cyclic quadrilateral the opposite angles are supplementary.
Thus the value of angle
26. In the figure, given alongside, AB//CD and O is the centre of the circle. If
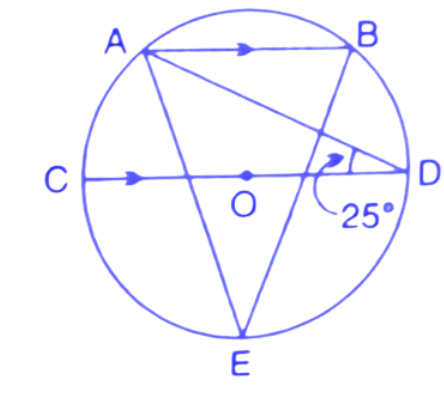
Ans: First we join the points A to C, B to D and B to C.
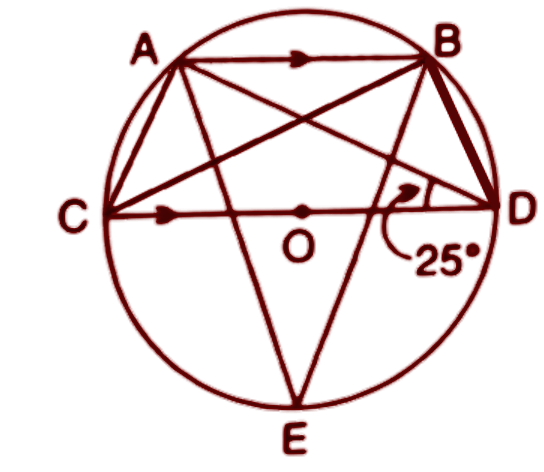
We know that the angle made in a semicircle is the right angle. Here CD is the diameter and
From the figure, AB||CD and AD is a transversal line.
So,
ABDC is a cyclic quadrilateral and we know that in a cyclic quadrilateral the sum of opposite angles is
In a circle, the angle subtended by the same chord on the circumference is always equal. Here
Hence,
Thus the value of angle
27. Two circles intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at C and D. Prove that AC is parallel to BD.

Ans: At first we join the points AC , PQ and BD.
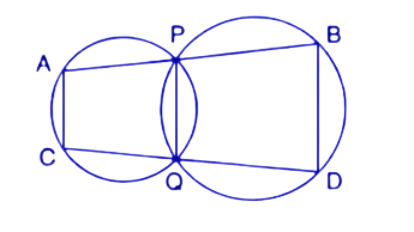
Since, APQC is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
So,
Similarly, PBDQ is a cyclic quadrilateral.
Since, CQD is a straight line. Hence,
From eq.(1),(2)&(3)
Here AB is the transversal line and angles
Hence, AC||BD.
28. ABCD is a cyclic quadrilateral in which AB and DC on being produced, meet at P such that PA=PD. Prove that AD is parallel to BC.
Ans:
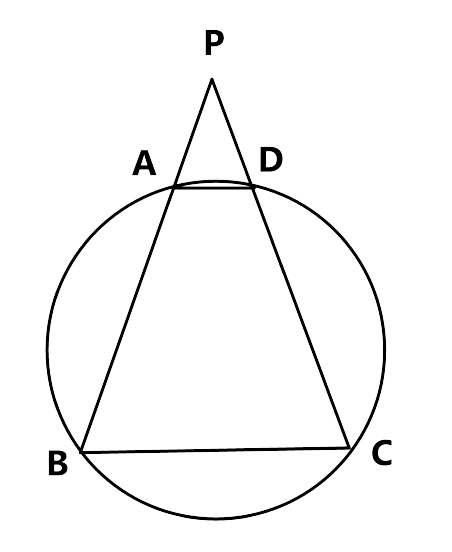
Given, PA=PD
Hence,
Here PAB and PDC are straight lines.
Therefore,
and, for straight line PDC
ABCD is a cyclic quadrilateral and we know that in a cyclic quadrilateral the sum of opposite angles is
And,
By eq. (1),(2),(3) and (4), we get
We know that if corresponding angles are equal then the lines are parallel.
Hence, AD||BC.
29. AB is a diameter of the circle APBR as shown in a figure. APQ and RBQ are straight lines, Find:
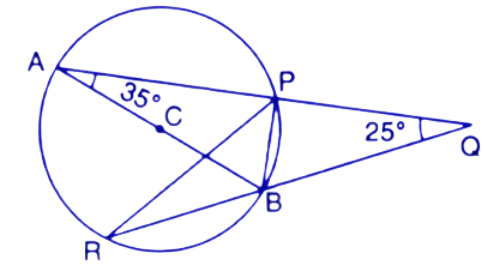
(i).
Ans: In a circle, Angles subtended by the same chord on the circumference are always the same.
Here Angles
So,
Thus the value of
(ii).
Ans: In a circle angle made by the diameter is a right angle. Here
Is made by the diameter AB. Hence
Given that APQ is a straight line , So
Now, In a triangle the exterior angle is equal to the sum of opposite interior angles of the triangle.
Thus the value of angle
(iii).
Ans: By the angle sum property, the sum of all interior angles is a triangle is always
By using angle sum property in
Thus the value of angle
30. In the given figure, SP is a bisector of
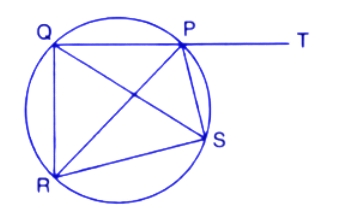
Ans: Given that PQRS is a cyclic quadrilateral.
In a cyclic quadrilateral, the sum of opposite angles is always
So,
Since QPT is a straight line. So,
From eq.(1) and (2)
PS is the bisector of
Therefore,
In a circle the angles subtended by the same chord are always equal.
Here angles
Hence
From eq.(3),(4) and (5)
We know that in a triangle if two angles are equal then their opposite sides are also equal.
Therefore, QS=SR (∵
31. In the figure, O is the centre of the circle,
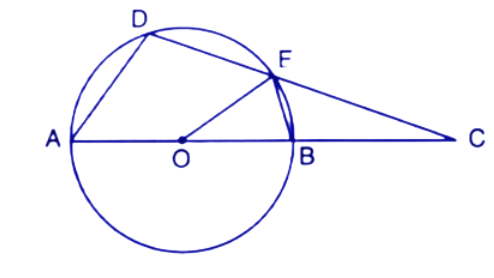
Ans: In a circle the angle at the centre is doubled the angle at the circumference by the same chord.
In a cyclic quadrilateral the sum of opposite angles is
DEC is a straight line and the sum of angle on a straight line is
Thus the value of
By using the angle sum property in
Thus the value of angle
32. In the figure, given below, P and Q are the centres of two circles intersecting at B and C. ACD is a straight line. Calculate the numerical value of x.
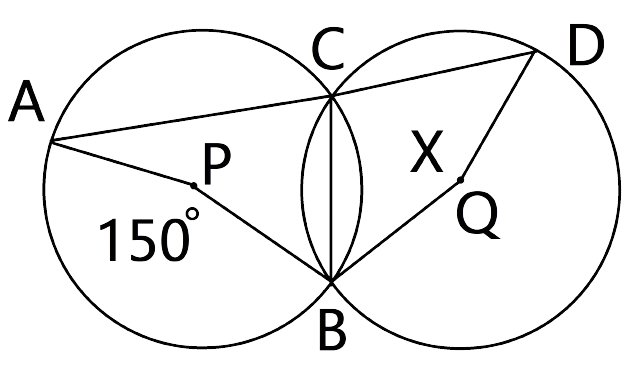
Ans: We know that the angle at the centre is doubled the angle at any point of circumference subtended by the same chord.
Sum of the angles in a straight line is
For straight line ACD,
In a circle, The angle at the centre is doubled the angle at the circumference subtended by the same chord.
Now,
Thus the value of x is
33. The figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given
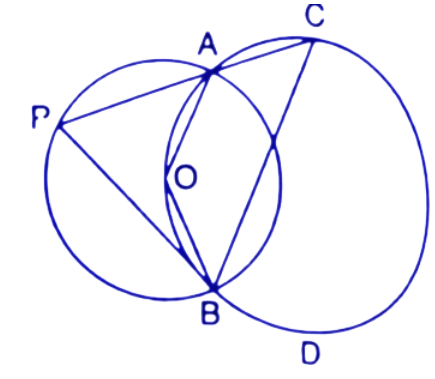
(i). Obtuse
(ii).
(iii).
Give reasons for your answer clearly.
(i). obtuse
Ans: In a circle the angle at the centre is doubled the angle at the circumference subtended by the same arc.
Here in smaller circles angle
Therefore,
Thus the value of
(ii).
Ans: We know that in a cyclic quadrilateral the sum of opposite angles is
So,
Thus the value of
(iii).
Ans: Here join AB, AD and BD.
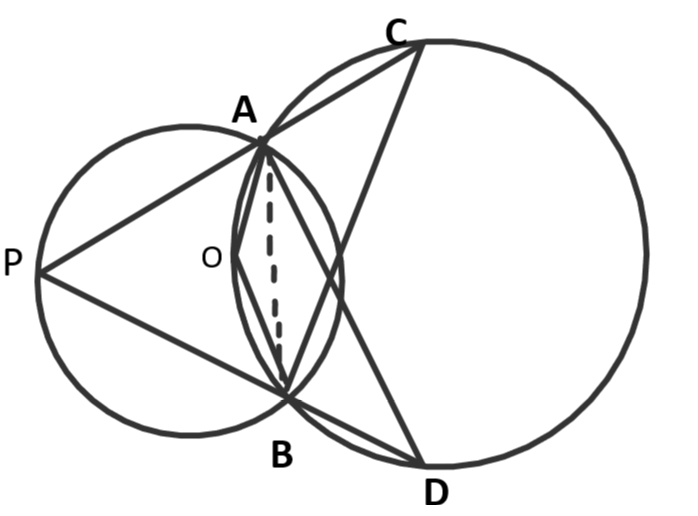
In a circle angles subtended by the same chord are always equal. Here angles
Therefore,
Thus the value of
34. In the given figure, O is the centre of the circle and
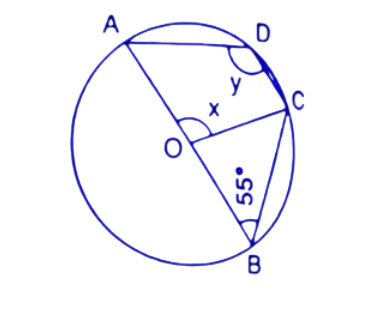
Ans: In a circle angle at the centre is doubled the angle at the circumference subtended by the same chord.
So,
From the figure,
ABCD is a cyclic quadrilateral and we know that in a cyclic quadrilateral the sum of opposite angles is
Since ,
So,
Thus the values of x, y are
35. In the given figure, A is the centre of the circle, ABCD is a parallelogram and CDE is a straight line. Prove that:
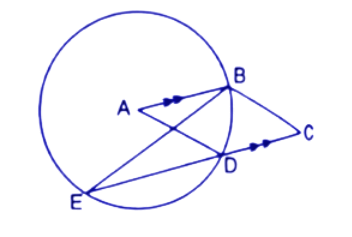
Ans: In a circle the angle at the centre is double the angle at the circumference subtended by the same chord.
Since
From eq.(1) and (2).
ABCD is a parallelogram hence the opposite angles in a parallelogram are equal.
So,
From eq.(3) and (4)
Hence proved.
36. ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given
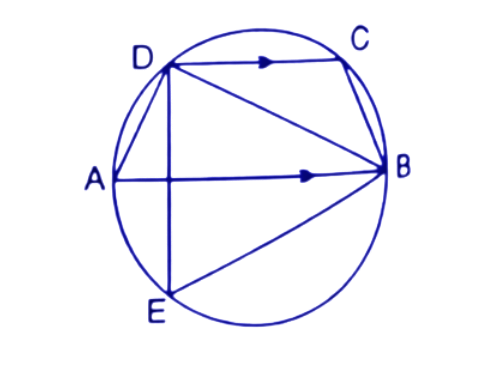
(i).
Ans: The angle subtended by the same chord
Are always equal. Here angles
Hence,
Thus the value of
(ii).
Ans: In a triangle by the angle sum property the sum of all the interior angles is
In
Since, Lines AB and CD are parallel.
Thus the value of
37. In the given figure, AB is the diameter of the circle. Chord ED is parallel to AB and
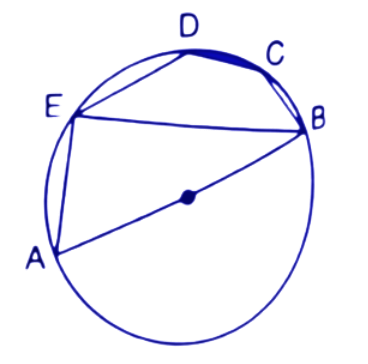
(i).
Ans: The angle made in the semicircle is always
Here
Hence,
By the angle sum property in a triangle the sum of all interior angles is
In
Thus the value of
(ii).
Ans: Given that ED||AB
Hence
Since BCDE is a cyclic quadrilateral and we know that in a cyclic quadrilateral the sum of opposite angles is
Hence
Thus the value of
38. In the given figure, AB is the diameter of the circle with centre o. DO is parallel to CB and
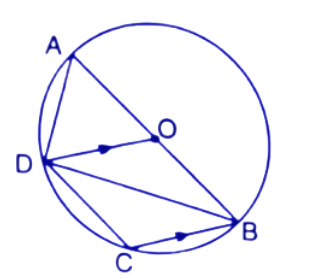
(i).
Ans: Since ABCD is a cyclic quadrilateral. The sum of opposite angles in a cyclic quadrilateral is
Thus the value of
(ii).
Ans: Angle subtended by the diameter at any point on circumference is
So,
By the angle sum property in
Thus the value of
(iii).
Ans: Since OD=OB (Radii of circle)
∴
Given, OD||BC
Thus the value of
(iv).
Ans: Since ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
So,
By using angle sum property in
Now,
Thus the value of
In
OA=OD (Radii of circle)
∴
From Part (i)
Therefore the triangle AOD is an equilateral triangle.
39. In the given figure, I is the incentre of
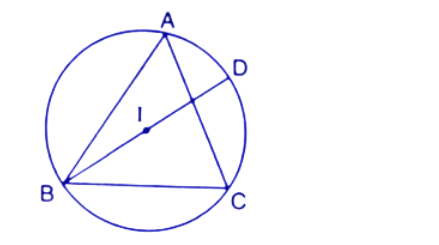
(i).
Ans: Join the Points IA, IC , AD and CD.
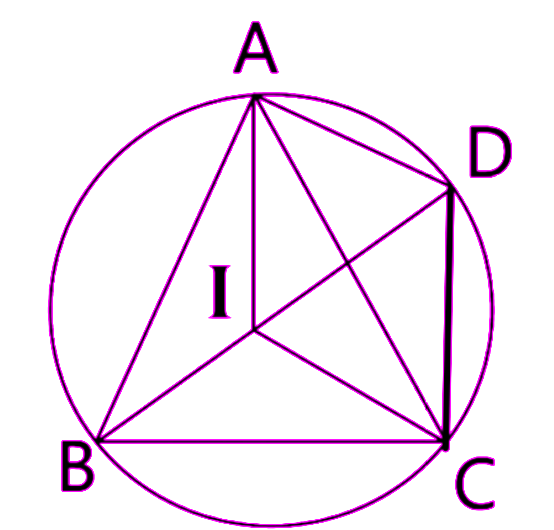
IB is angle bisector of angle
By using the angle sum property in
By putting above value in eq. (i)
The angles subtended by the same chord are equal. Angles
So,
Thus the value of
(ii).
Ans: IB is the angle bisector of
Angles subtended by the same chord at any point on circumference are equal.
Angles
So,
Thus the value of
(iii).
Ans: CI is the angle bisector of
Thus the value of
(iv).
Ans: AI is the angle bisector of
Therefore,
Now, by using the angle sum property in
Thus the value of
40. In the triangle ABC is inscribed in a circle. The bisectors of angles BAC, ABC and ACB meet the circumcircle of the triangle at points P,Q and R respectively. Prove that:
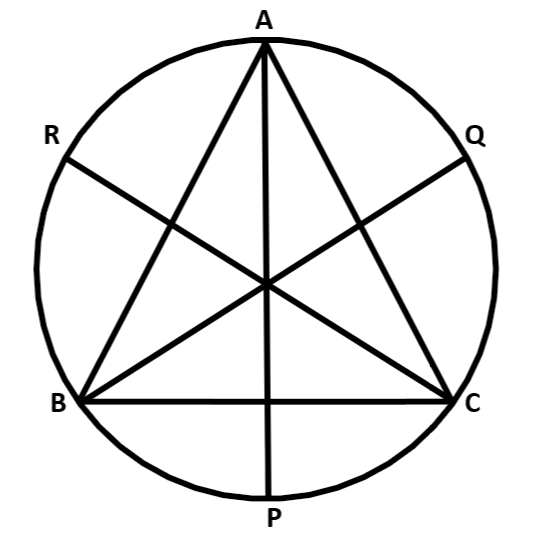
Ans: Join Points PR and PQ.
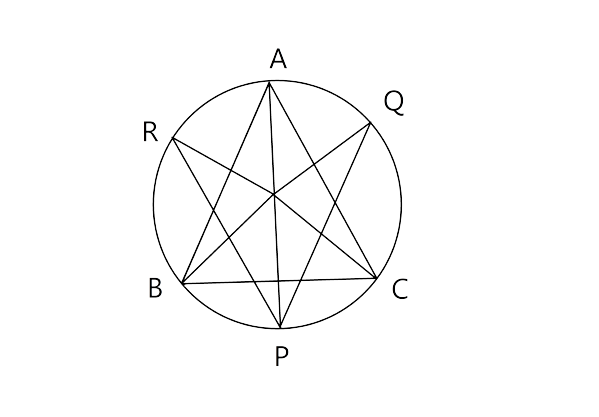
(i).
Ans: BQ is the angle bisector of
So,
Angles subtended by the same arc on circumference are always equal. Angles
From eq.(1) and (2).
Hence proved.
(ii).
Ans: CR is an angle bisector of
In a circle angles subtended by the same arc on circumference are equal. Angles
So,
From eq.(3) and (4)
Hence Proved.
(iii).
Ans: Since From the (i) and (ii) parts
Now, On additing
Hence proved.
41. Calculate the angles x,y and z if :
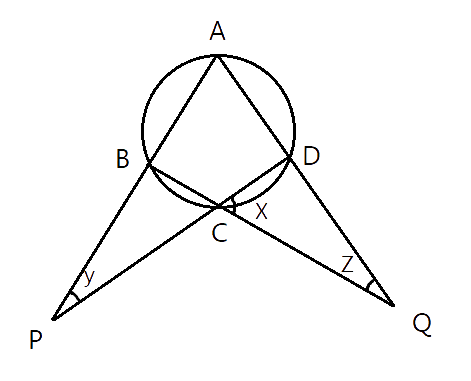
Ans:
Let
Now by using exterior angle property, the exterior angle is equal to the sum of opposite angles.
Also,
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Therefore the values of x,y andz.
42. In the given figure, AB=AC=CD and
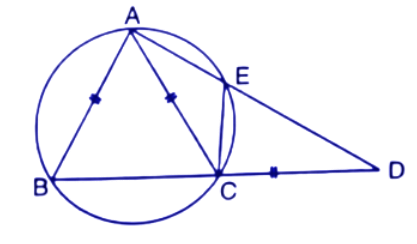
(i).
Ans: Given AC=CD
By using angle sum property in
From the figure, BCD is a straight line.
Given, AB=AC
Thus the value of
(ii). Angle BEC
By using the angle sum property in
Angles subtended by the same chord on the circumference are equal.
Angles
Therefore,
43. In the given figure, AC is the diameter of the circle, centre O. Chord BD is perpendicular to AC. Write down the angles p,q and r in terms of x.
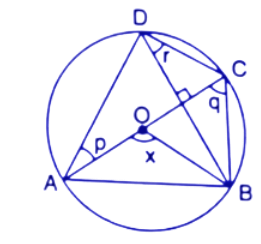
Ans: In a circle the angle at the centre is double the angle at any point on circumference subtended by the same chord.
Angles subtended by the same chord on the circumference are equal.
From eq.(1) and (2)
The angles subtended by the same chord on the circumference are equal. Here angles
By exterior angle property,
In a circle, the angle made by the diameter is the right angle.
Thus the values of p,q and r are
44. In the given figure, AC is the diameter of the circle with centre O. CD and BE are parallel. Angle
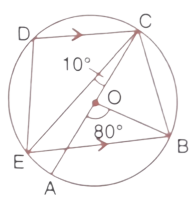
(i). Angle BEC,
Ans: AOC is a straight line.
Hence,
Angle at the centre is doubled the angle at the circumference subtended by the same chord.
So,
Thus the value of
(ii)Angle BCD,
Ans: DC||EB
The angle at the centre is doubled the angle at the circumference subtended by the same chord.
(iii). Angle CED
Ans: BCDE is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
45. In the given figure, AE is the diameter of the circle. Write down the numerical value of
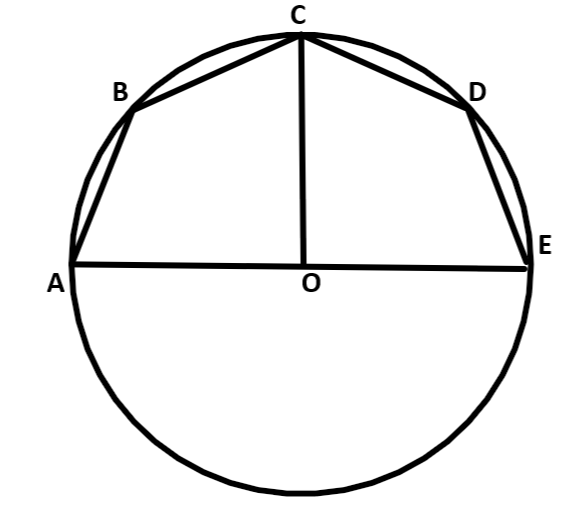
Ans:
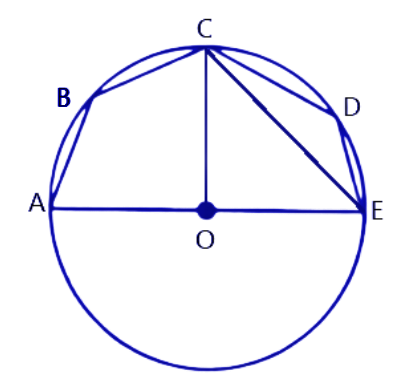
Join the points CE and OC.
AOE is a straight line.
Hence,
In a circle angle at the centre is doubled the angle at the circumference subtended by the same arc.
ABCE is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Similarly,
Thus the value of
46. In the given figure, AOC is a diameter and AC is parallel to ED. If
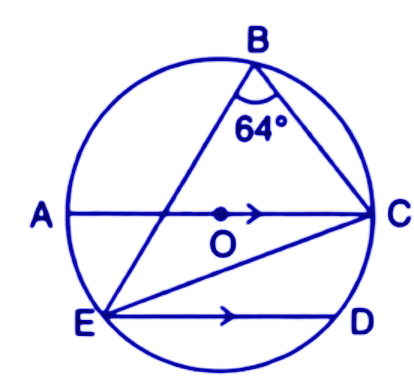
Ans: Join the point AB.
In a circle angle subtended by the diameter at any point on the circumference is a right angle.
From the figure,
Angles subtended by the same arc on the circle are always equal.
Since, AC||ED
Therefore,
Thus the value of
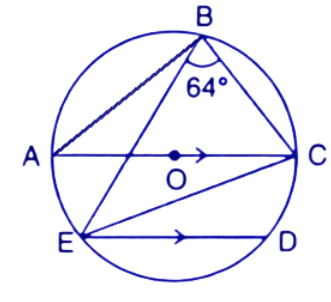
47. Use the given figure to find :
(i).
Ans: By the angle sum property the sum of all interior angles in a triangle is
In
Thus the value of
(ii).
Ans: ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Now by using angle sum property in
Thus the value of
48. In the given figure, AOB is a diameter and DC is parallel to AB. If
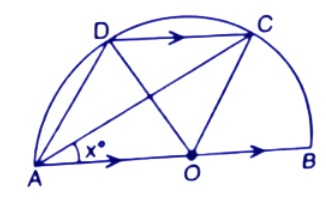
(i).
Ans: In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same arc.
Thus the value of
(ii).
Ans: Since DC||AB
Hence,
OC=OD (Radii of circle)
By using angle sum property in
(iii).
Ans: In a circle, Angle at the centre is doubled the angle at circumference subtended by the same chord.
Thus the value of
(iv).
Ans: Since, DC||AO
By using angle sum property in
Thus the value of
49. In the given figure, AB is the diameter of a circle with centre O.
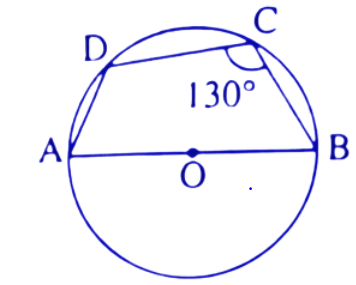
(i).
Ans: ABCD is a cyclic quadrilateral. In a cyclic quadrilateral the sum of opposite angles is
Hence,
(ii).
Ans: In the given figure join BD.
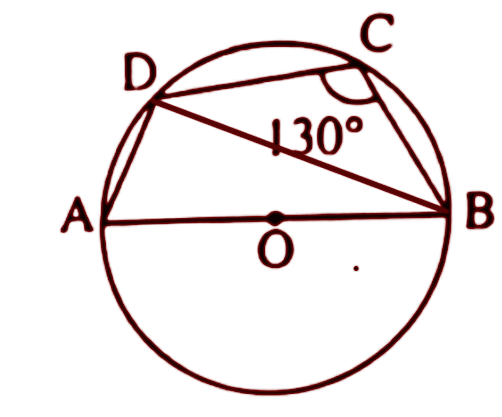
In a circle angle subtended by the diameter at the circumference is right angle. Hence
By using the angle sum property in
Thus the value of
50. In the given figure. PQ is the diameter of the circle whose centre is O. Given
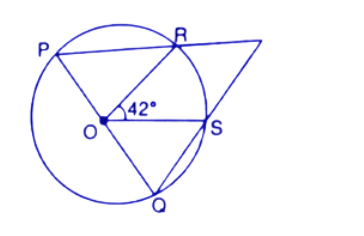
Ans: Join points RS and PS.
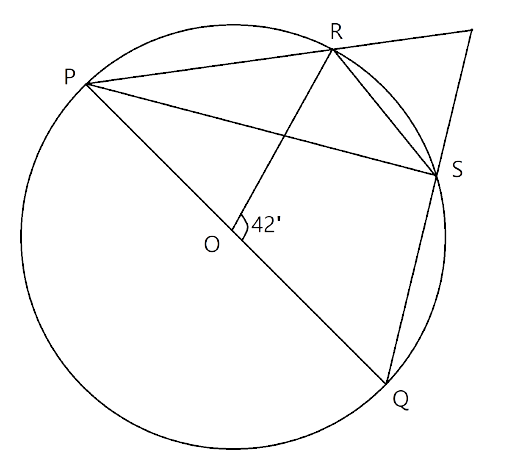
In a circle angle subtended by the diameter at circumference is right angle.
In a circle angle at the centre is doubled the angle at the circumference subtended by the same chord.
Therefore
Now in
Thus the value of
51. In the given figure, PQ is a diameter. Chord SR is parallel to PQ. Given that
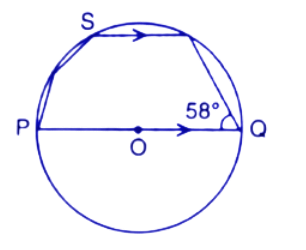
(i).
Ans: Join Points PR
Angle subtended by the diameter on the circumference is the right angle.
Now by using angle sum property in
Thus the value of
(ii).
Ans: Since SR||PQ
∴
STPR is a cyclic quadrilateral. In a cyclic quadrilateral the sum of opposite angles is
Thus the value of
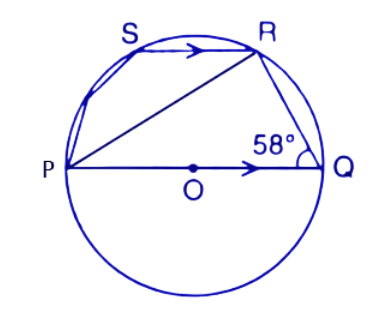
52. AB is the diameter of the circle with centre O. OD is parallel to BC and
Calculate the numerical values of :
(i).
Ans: Join points BD.

Angle at the centre is doubled the angle at the circumference subtended by the same chord.
Thus the value of
(ii).
Ans: AOB is a straight line.
Now in
Since OD||BC
∴
Thus the value of
(iii).
Ans: ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Now,
Thus the value of
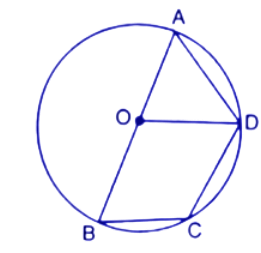
53. In the given figure, the centre O of the small circle lies on the circumference of the bigger circle. If

(i).
Ans: Join points AB and AD.
In a circle, the angle at the centre is twice the angle at any point on circumference subtended by the same chord.
Thus the value of
(ii).
Ans: AOBC is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
(iii).
Ans: Since
From (ii) part
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
(iv).
Ans: OABD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
54. In the given figure,
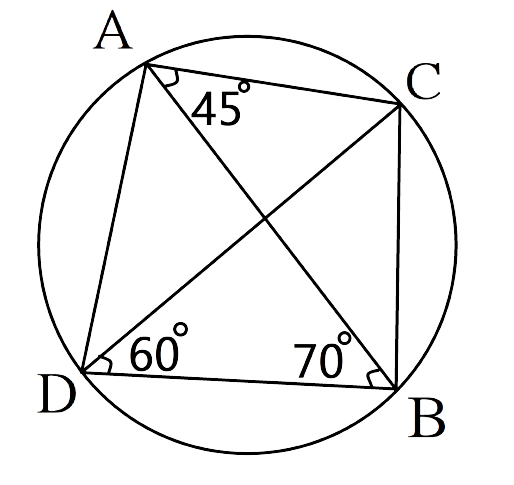
(i).
(ii).
Hence show that AC is a diameter.
(i).
Ans: ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
(ii).
Hence show that AC is a diameter.
Ans: In
In a circle angles subtended by the same chord on the circumference are equal. Angles
Hence,
Thus the value of
Since,
Thus AC is the diameter.
55. In a cyclic quadrilateral ABCD,
Ans: Let the cyclic quadrilateral ABCD be as below:
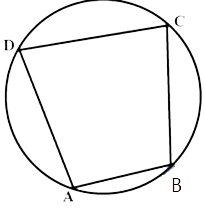
and
In a cyclic quadrilateral the sum of opposite angles is
Thus angles
Now, Let
Therefore angles
56. The given figure shows a circle with centre O and
Calculate the measure of :
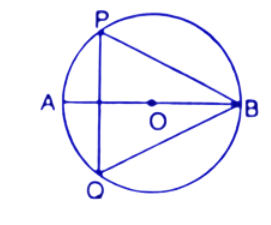
(i).
Ans: Join the points AP
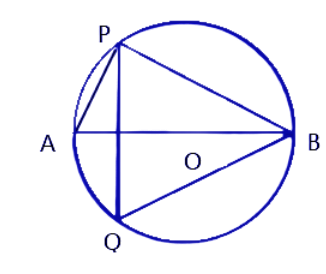
In a circle Angle subtended by diameter at the circumference is the right angle.
By using angle sum property in
In a circle, Angles at the circumference subtended by the same chord are always equal. Here
∴
Thus the value of
(ii).
By using angle sum property in
Thus the value of
57. In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If

(i). Express
Ans: AM=MD(Radii of circle)
Opposite angles of equal sides are always equal.
∴
By using angle sum property in
Thus the value of
(ii). Express
Ans: In a circle Angle at the centre is doubled the angle at the circumference subtended by the same chord.
In
In a circle angles subtended by the same chord at the circumference are always equal.
Thus the value of
(iii). Prove that:
From eq. (1) and (2).
Hence proved.
Exercise-17B
1. In a cyclic trapezium, the non-parallel sides are equal and the diagonals are equal, Prove it.
Ans:
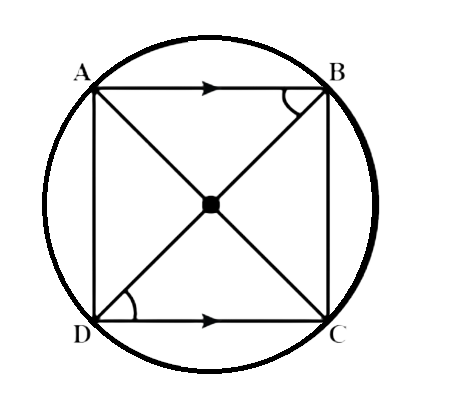
ABCD is a cyclic trapezium and AB||CD
In a circle, the angle at the centre is twice the angle at the circumference subtended by the same chord.
And,
Since,
We know that if the angles at the centre subtended by different chords are equal then the chords are equal to each other.
Since,
Therefore, AD=BC
Now, In
DC=DC (Common side)
AD=BC (Proved above)
Therefore,
2. In the following figure, AD is the diameter of the circle with centre O. Chords AB, BC and CD are equal. If
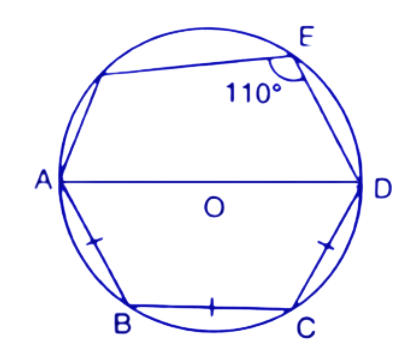
(i).
Ans: We Join points OB,OC and AE.
In a circle, Angle subtended by diameter at any point of the circumference is a right angle.
∴
Given,
Thus the value of
(ii).
Ans: Given that Chord OA=OB=OC
Equal chords subtend the equal angles at the centre.
The sum of all angles on one side of a straight line is always
Since OA=OB (Radii of circle)
By using angle sum property in
Therefore,
AFED is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Now, From the figure:
Thus the value of
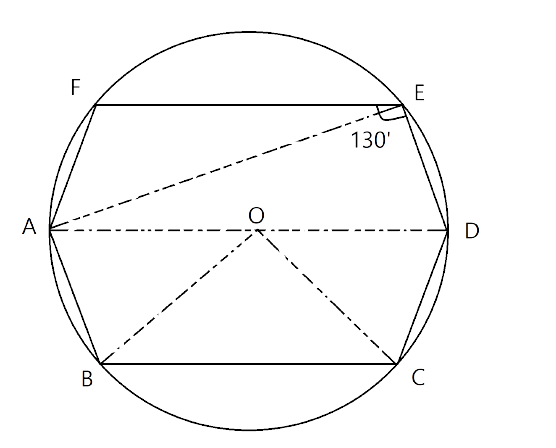
3. If two sides of a cyclic-quadrilateral are parallel; Prove that:
(i). Its other two sides are equal.
Ans:
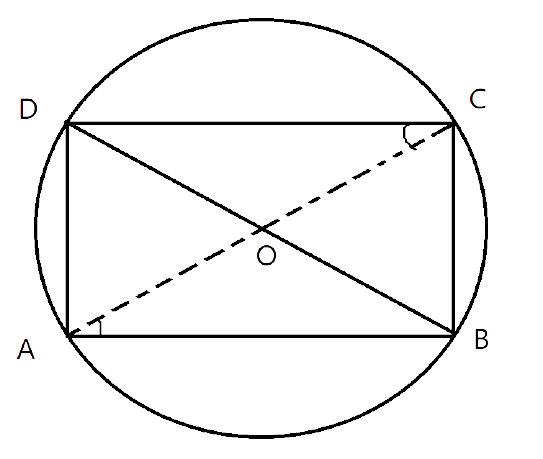
Let DC||AB
In a circle, Angle at the centre is twice the angle at the circumference subtended by the same chord.
Since
∴
By the circle theorem, We know that if angles at the centre subtended by the different chords are equal then chords are equal to each other.
∴ chord AD=chord BC
⇒AD=BC (Hence proved)
(ii). Its diagonals are equal.
Ans: In
AB=AB (common side)
BC=AD (Proved in i part)
Therefore,
⇒ AC=BD (Hence proved)
4. The given figure shows a circle with centre O. Also, PQ=QR=RS and

(i).
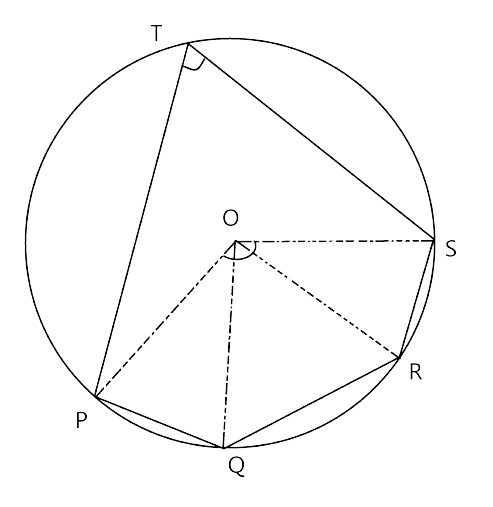
Ans: Join the lines OP, OQ , OQ and OS.
Given, PQ=QR=RS
Equal chords subtend the equal angles at centre.
In a circle angle at the centre is doubled the angle at circumference subtended by the same arc.
Thus the value of
(ii).
Ans: since
Thus the value of
(iii).
Ans: In
OP=OQ (Radii of circle)
∴
Now, By using angle sum property.
Similarly in
Now,
Thus the value of
5. In the given figure, AB is a side of a regular six-sided polygon and AC is a side of a regular eight-sided polygon inscribed in the circle with centre O. Calculate the sizes of :
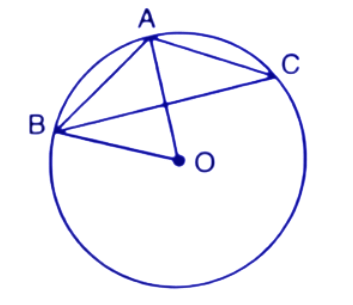
(i).
Ans: AB is the a side of a regular six-sided
polygon so this subtend the angle
(ii).
Ans: In a circle, Angle at the centre is twice the angle at any point of circumference subtended by the same chord.
(iii).
Ans:
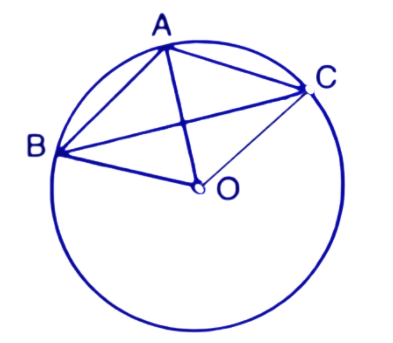
Join the line OC.
AC is a side of a eight-sided regular polygon, So AB subtends the angle at centre as:
In a circle, Angle at the centre is twice the angle at the circumference subtended by the same chord.
Thus the value of
6. In a regular pentagon ABCDE, inscribed in a circle; find the ratio between angle EDA and angle ADC.
Ans:
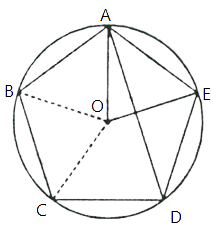
ABCDE is regular pentagon and each side subtends the angle at centre as:
So the angles
In a circle, Angle at the centre is twice the angle at circumference subtended by the same chord.
Similarly,
From the figure,
Now,
Thus the ratio is
7. In the given figure, AB=BC=CD and
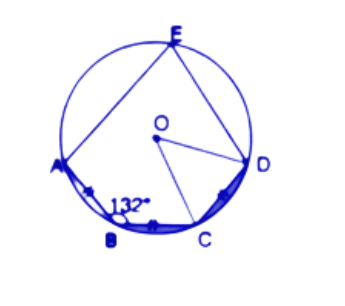
(i).
Ans: Join points EB and EC
ABCE is cyclic quadrilateral and in ac cyclic quadrilateral the sum of opposite angles is
Given, chord AB= chord BC. So, they will subtend the same angle.
And,
Thus the value of
(ii).
Ans: Since, AB=BC=CD
Equal chords subtend the equal angles.
Hence,
And,
Thus the value of
(iii).
Ans: In a circle, Angle at the centre is twice the angle at the circumference subtended by the same chord. Here angles
Thus the value of
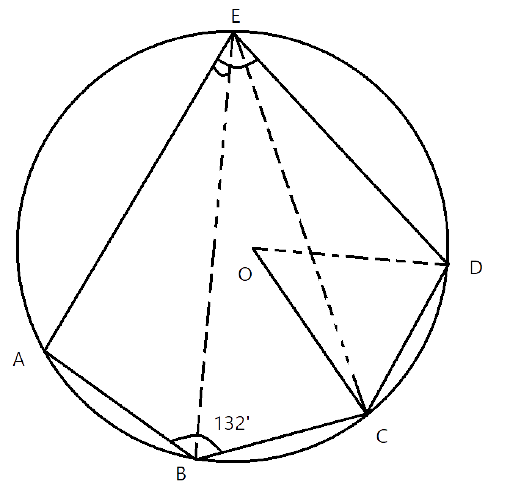
8. In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle
(i).
Ans: Join AD and BD.
Given, arc AB= 2 arc BC
Therefore,
In a circle angle at the centre is doubled the angle at the circumference subtended by the same chord. Here
So,
Thus the value of
(ii).
Ans: In a circle angle at the centre is doubled the angle at the circumference
Subtended by the same chord.
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
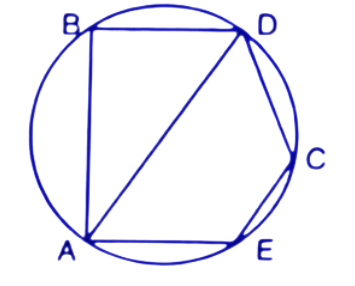
9. The figure shows a circle with centre O. AB is the side of the rectangular pentagon and AC is the side of the regular hexagon. Find the angles of triangle ABC.
Ans: Join the points OA, OB and OC
Given that AB is a side of regular pentagon and we know that each side of pentagon inscribed in a circle subtends the angle of measure
Thus the angle AOB subtended by chord AB=
AC is a side of regular hexagon and we know that each side of hexagon inscribed in a circle subtends the angle of measure
Thus the angle AOC subtended by chord AC=
In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.
And,
By using angle sum property in
Thus the value of angles of
10. In the given figure, BD is a side of a regular hexagon, DC is a side of a regular pentagon and AD is a diameter. Calculate :
Ans: Join the points CO, EO, BC and BO.
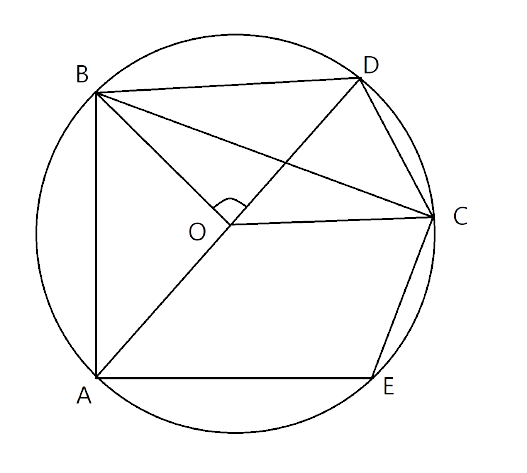
(i).
Ans: Since, BD is a side of regular hexagon, hence it subtends the angle at centre as:
Similarly, CD is a side of regular pentagon, hence it subtends the angle at centre as:
In
And, In
OC=OD (Radii of circle)
Thus the value of
(ii).
Ans: From (i) part
(iii).
Ans: In a circle, Angle at the centre is doubled the angle at circumference subtended by the same chord.
Thus the value of
(iv).
Ans:
Thus the value of
Exercise-17(C)
1. In the given circle with diameter AB, find the value of x.
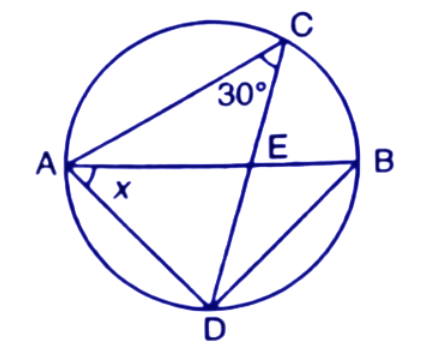
Ans: In a circle, Angles at the circumference subtended by the same chord are equal.
The angle on the circumference subtended by the diameter is a right angle.
Now, By using the angle sum property in
Thus the value of
2. In the given figure, ABC is a triangle in which

Ans: Join the points OB, OC.
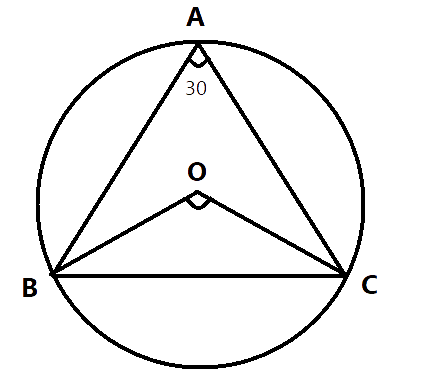
In a circle, Angle at the centre is doubled the angle at the circumference subtended by the same chord.
Since, OB=OC (Radii of same circle)
Now, by using the angle sum property in
Thus,
Therefore
Hence, OB=OC=BC.
So, BC is equal to the radius of the circumcircle of the triangle ABC.
3. Prove that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
Ans:

Given AB is a diameter.
In a circle angle subtended by diameter is a right angle.
SO,
BC is a straight line and the sum of angles on a straight line is
Now, In
AD=AD (Common side)
AB=AC (Given)
Therefore, BD=DC
Thus D is a midpoint of BC.
4. In the given figure, chord ED is parallel to diameter AC of the circle. Given
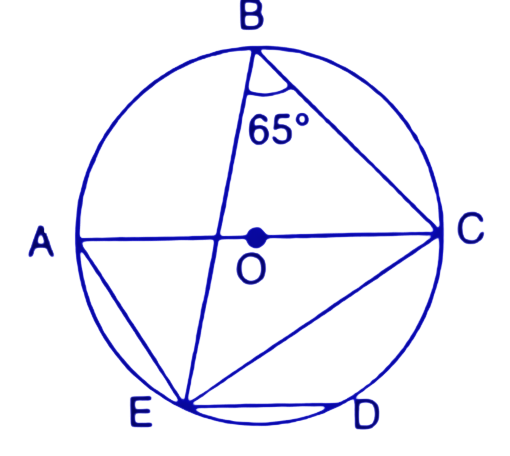
Ans: Join the points AB, EO and DC.
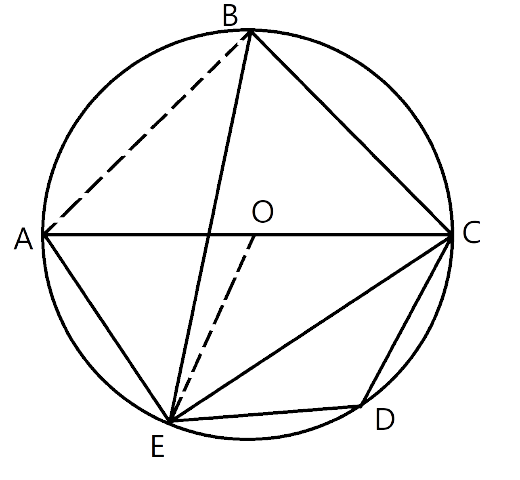
In a circle, Angle at the centre is doubled, the angle subtended by the same chord.
Since, OE=OC (Radii of same circle)
Now, In
Hence,
Given, ED||AC
Therefore,
Thus the value of
5. The quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic. Prove it.
Ans:
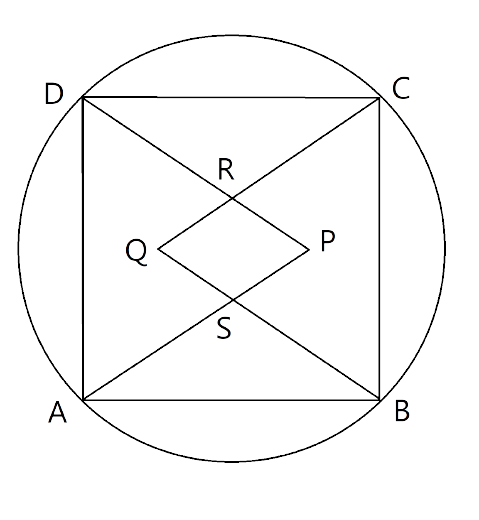
In
In
By adding eq. (1) and (2)
Since AP, BQ, CQ and DP are angle bisectors of
Therefore,
By adding Eq. (4), (5), (6) and (7)
Now by putting this value from eq. (8) in eq. (3)
We know that the sum of all interior of a quadrilateral is
Thus PSQR is a cyclic quadrilateral.
6. In the figure,

(i).
Ans: In a circle, Angle at the circumference subtended by the diameter is the right angle.
Now by using angle sum property in
Thus the value of
(ii).
Ans: BECD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
(iii).
Ans: In a circle, Angles at the circumference subtended by the same chord are equal.
Therefore,
7. D and E are points on equal sides AB andAC of an isosceles triangle ABC such that AD=AE. Prove that the points B, C, E and D are concyclic.
Ans:
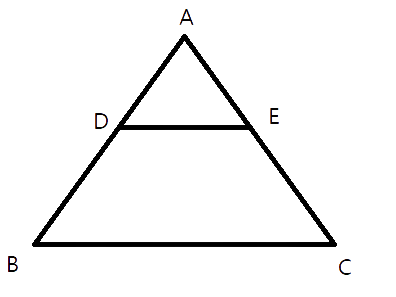
Given, AB=AC
And, AD=AE
In
Therefore, DE||BC
And,
From eq. (1) and (2)
Thus BCDE is a cyclic quadrilateral. Hence, B,C,D and E are concyclic points.
8. In the given figure, ABCD is a cyclic quadrilateral. AF is drawn parallel to CB and DA is produced to point E. If
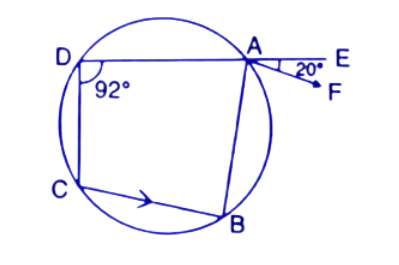
Ans: Given, ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Since, AF||BC
Now,
In a cyclic quadrilateral the exterior angle is equal to the interior opposite angle.
Thus the value of
9. If I is the incentre of triangle ABC and AI when produced meets the circumcircle of triangle ABC in point D. If
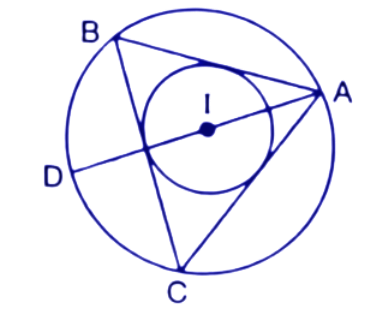
Ans:
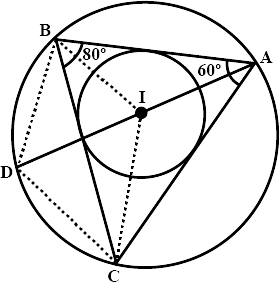
Join the points BD, CD, IB and IC.
(i).
Ans: IA is angle bisector. So,
In a circle, Angles subtended by the same chord at the circumference are always equal.
Thus the value of
(ii).
Ans: IB is an angle bisector. So,
Thus the value of
(iii).
Ans: By using angle sum property in
IC is an angle bisector of
Now, By using angle sum property in
Thus the value of
10. In the given figure, AB=AD=DC=PB and

(i).
Ans: Join the points AC and BD.
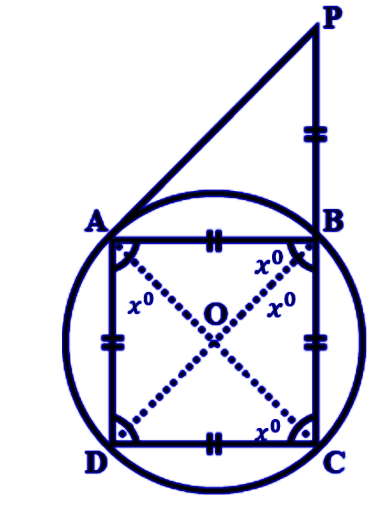
In a circle, Angles subtended by the same chord at the circumference are always equal.
Therefore,
Thus the value of
(ii).
Hence or otherwise, prove that AP is parallel to DB.
Ans: Since, AB=PB
In
We know that the exterior angle is equal to the sum of opposite angles.
Thus the value of
Since,
These are corresponding angles and we know that if two corresponding angles are equal the sides are also equal.
Therefore AP||DB.
11. In the given figure: ABC, AEQ and CEP are straight lines. Show that
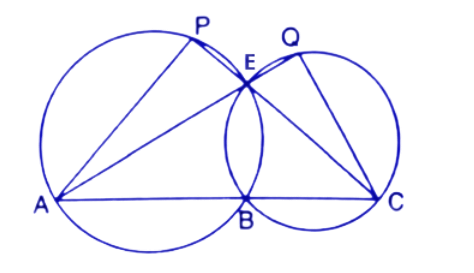
Ans: Join the line EB.
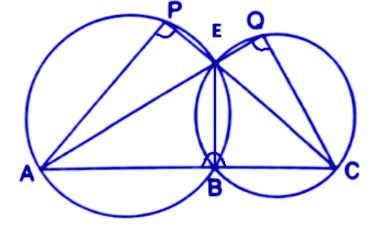
In a cyclic quadrilateral the sum of opposite angles is
ABEP is a cyclic quadrilateral. Hence,
BEQC is a cyclic quadrilateral. Hence,
On adding eq. (1) and (2)
Thus,
12. In the given figure, AB is the diameter of the circle with centre O. If
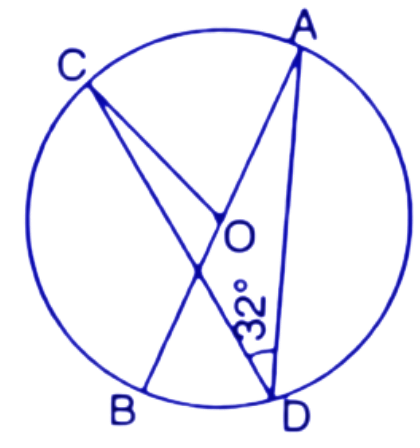
Ans: In a circle, The angle at the centre is doubled at the circumference subtended by the same chord.
Since,
Thus the value of
13. In a cyclic-quadrilateral PQRS, angle
If
Ans:
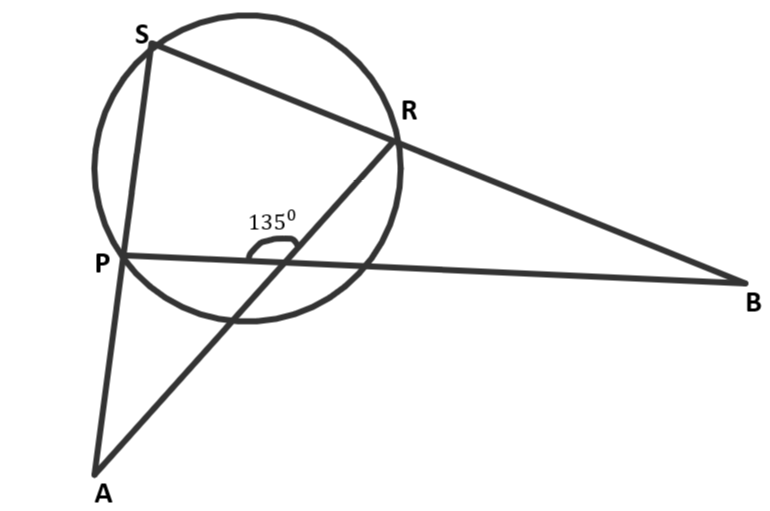
Let
PQRS is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
AQR is a straight line and the sum of angles on a straight line is
By using angle sum property in
Now by using exterior angle property in
From eq. (1) and (2)
Therefore the angle
14. In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.
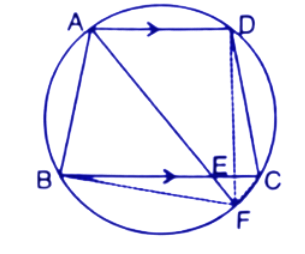
If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
(i). EF=FC
Ans: AF is angle bisector of angle
Hence,
Also,
∵ AD||BC
∴
From eq. (1) and (2)
In
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Also, ADCF is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
By using angle sum property in
since,
and
And,
From eq.(3) and (4)
If in a triangle two angles are equal then the opposite sides of them are also equal.
∴ EF=FC
(ii). BF=DF
Ans: Since AF is angle bisector of
∴
Angle
So, Chord BF= Chord DF
⇒ BF=DF
15. ABCD is a cyclic quadrilateral. Sides AB and DC produced meet at point E; whereas sides BC and AD produced meet at point F.
If
Ans:
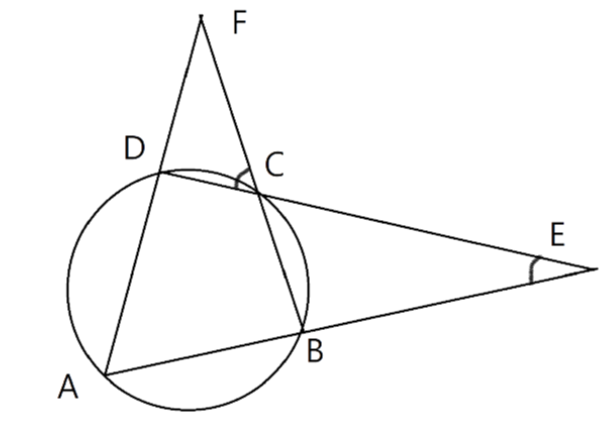
Let
BCF is a straight line.
ABCD is a cyclic quadrilateral and the sum of opposite angles is
In
Since,
So,
In
Ext.
ABCD is a cyclic quadrilateral and the sum of opposite angles is
Therefore the values of angles of a quadrilateral,
16. The following figure shows a circle with PR as its diameter. If PQ=7cm and QR=3RS=6cm, find the perimeter of the cyclic quadrilateral PQRS.

Ans: Since, PQ=7cm and QR=3RS=6cm
So,
Now by using pythagoras theorem,
Similarly,
Therefore the perimeter of cyclic quadrilateral,
17. In the figure, AB is the diameter of a circle with centre O. If chord AC=chord AD, prove that :
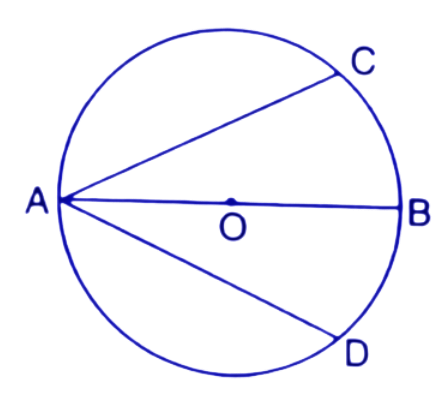
(i). Arc BC=arc DB
(ii). AB is bisector of
Further, if the length of arc AC is twice the length of arc BC, find :
(a).
(b).
Ans: Join points BC and BD

(i). Arc BC=arc DB
Ans: In a circle, Angle subtended by the diameter at the circumference is a right angle.
Therefore,
Now, In
AB=AB (Common side)
AC=AD (Given)
Therefore, BC=BD
(ii). AB is bisector of
Ans: Since,
Therefore,
So AB is a bisector of
Further, if the length of arc AC is twice the length of arc BC, find :
(a).
Ans: If arc AC= 2 Arc BC
Now by using the angle sum property in
(b).
Ans: Since,
Therefore the value of
18. In cyclic quadrilateral ABCD; AD=BC,
(i).
Ans:
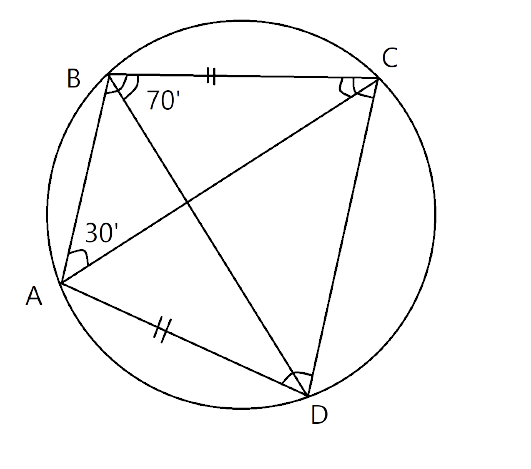
In a circle, the angles subtended by the same chord at the circumference are equal. Angles
Hence,
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
(ii).
Ans: By using angle sum property in
Since, AD=BC
Therefore,
Since,
(iii).
Ans: In a circle, Angles at the circumference subtended by the same chord are always equal.
So,
(iv).
Ans: ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
19. In the given figure,
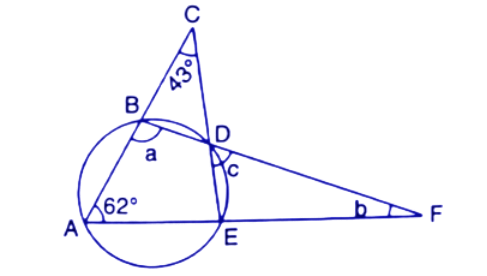
Ans: By using angle sum property in
ABDE is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
From figure,
Thus the value of
By using angle sum property in
By using exterior angle property in
Exterior
Thus the values of
20. In the given figure, AB is parallel to DC,
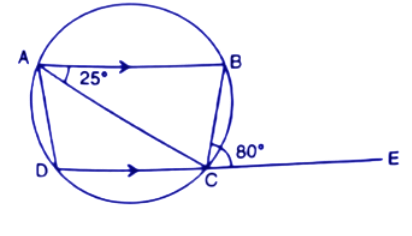
Find :
(i).
Ans: By exterior angle property, the exterior angle is equal to the opposite angle.
Now,
(ii).
Ans: Join the points BD.
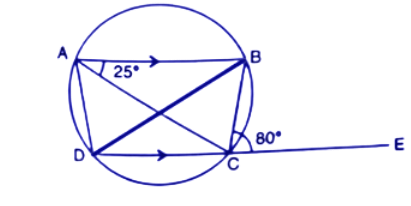
Angles at the circumference subtended by the same chord are always equal.
Here
From (i) part
Therefore,
Thus the value of
(iii).
Ans: Since AB||CD
By using angle sum property in
Thus the value of
21. ABCD is a cyclic quadrilateral of a circle with centre O such that AB is a diameter of this circle and the length of the chord CD is equal to the radius of the circle. If AD and BC produced meet at P, show that
Ans:
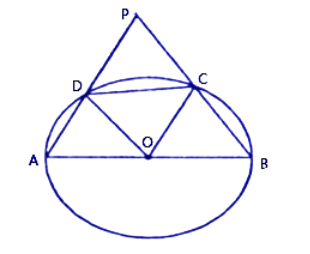
Given, Chord CD= radius OD=radius OC
Therefore,
Let
∵AO=DO and OC=OB (radii of circle)
∴
By using angle sum property in
In
Similarly, In
AOB is a straight line,
In
Thus the value of
22. In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.
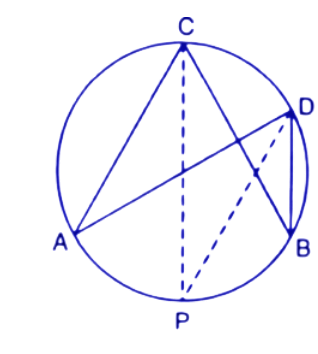
Ans: Since CP is an angle bisector of
Hence,
Angles at the circumference subtended by the same chord are equal.
By eq. (1), (2) and (3)
Therefore, DP is a bisector of angle BDA.
23. In the figure, given below, AD=BC,
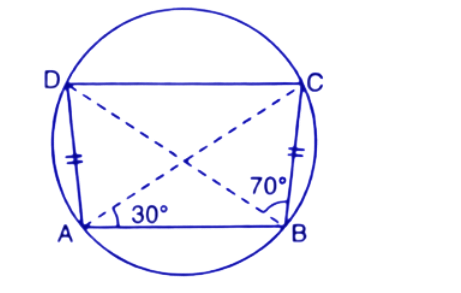
(i).
Ans: Angles at the circumference subtended by the same chord are equal.
Since,
ABCD is a cyclic quadrilateral and in cyclic quadrilateral the sum of opposite angles is
Thus the value of
(ii).
Ans: Since AD=BC and ABCD is an isosceles trapezium. Hence AB||CD.
Since,
Thus the value of
(iii).
Ans: In
Thus the value of
(iv).
Ans: In a circle, Angles at the circumference subtended by the same chord are always equal.
Thus the value of
24. In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and
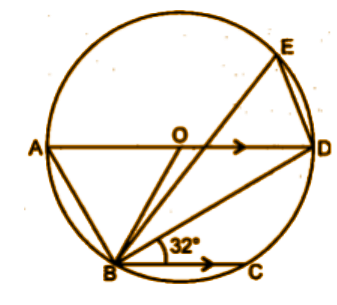
(i).
Ans: Since, AD||BC ⇒OD||BC
Therefore,
Since, OB=OD (radii of circle)
By equation (1) and (2)
(ii).
Ans: In
AD is a diameter that means AOD is a straight line. Hence
(iii).
Ans: OA=OB (radii of circle)
In
Angles at the circumference subtended by the same chord are equal. Here
Thus the value of
25. In the figure given, O is the centre of the circle.
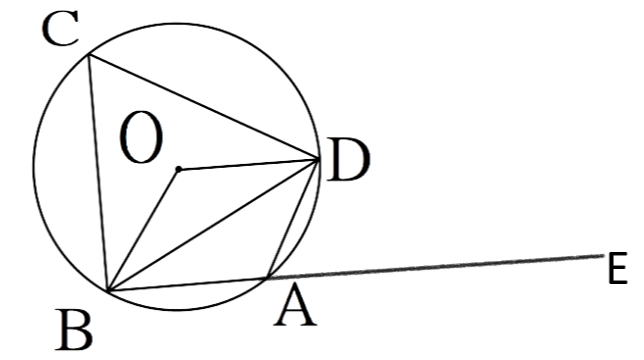
(i).
Ans:
ABCD is a cyclic quadrilateral and in a cyclic quadrilateral the sum of opposite angles is
Thus the value of
(ii).
Ans: In a circle, Angle at centre is doubled the angle at circumference subtended by the same chord.
Hence,
Thus the value of
(iii).
Ans: Since, OB=OD (radii of circle)
In
Thus the value of
ICSE Class 10 Mathematics Chapter 17 Selina Concise Solutions
Preparation Tips for Class 10 Maths
Some of the tips to be followed are as below:
Make a study plan analyzing the syllabus and the topics to be covered for each day.
Practice the theorems and the formulas with proper conceptual understanding.
Revise every day whatever has been studied previously.
Know the weak and the strong points in the chapter
Work more on the weak points.
Follow the steps while solving the problem.
Try not to skip any topics.
Take the mock test and solve the previous year’s question paper.
Practice and try to solve the problems quickly.
Improve on time management.
Enhance the speed of solving the problems.
List the formulas and theorems and revise them every day.
Introduction to the Chapter
This chapter teaches the students how two figures have the same shape but differ in size and yet they are called similar figures. This chapter mainly deals with the similarity of triangles, basics of understanding corresponding sides and corresponding angles of similar triangles, various conditions for similarity of two triangles, the Basic Proportionality theorem, the relation between the areas of two triangles, similarity as a size transformation, and finally the applications to maps and models are the main concepts of the chapter.
ICSE Class 10 Maths Solutions to Chapter 15
Students should learn all the concepts and theorems clearly without any confusion. Each chapter and the topics have to be learned with focus and concentration so that they can be easily remembered.
It is not just learning the concepts but also, to practice to answer relevant questions that are important.
Class 10 is very crucial and students should prepare well to face the challenges and also should be able to solve any problems given in the exam.
Class 10 is not just the completion of secondary school but also, students learn a lot of new concepts. The concepts and the chapters give a strong foundation when they enter classes 11 and 12. It becomes very important that the students focus completely on fundamentals.
The ICSE Solutions will help the students to understand the concepts in a simplified way. The steps provided in solving the problems were very beneficial learning and remembering the concepts.
Chapter 17 teaches the student about the circle and the basic types and parts of a circle, cyclic properties, and some important theorems and results. Students can get clarity of all these topics, concepts, and problem-solving techniques from Vedantu’s ICSE solution.
Maths is a subject that can be grasped only through practice. Students need to have a comprehensive study structure and follow it systematically. To achieve this, one has to plan out what topics to be covered each day.
FAQs on Concise Mathematics Class 10 ICSE Solutions for Chapter 17 - Circle
1. What is a circle according to class 10 ICSE Maths Chapter 17?
2. What does Theorem 13 state in class 10 ICSE chapter 17?
3. What do Theorem 14 and 15 states in class 10 ICSE chapter 17?
4. In chapter 17 of Class 10 ICSE Maths, what does theorem 20 state?
5. What are the benefits of Vedantu’s Class 10 ICSE Maths solutions?





































