
Which of the following is the path difference for destructive interference?
Answer
508.8k+ views
1 likes
Hint: Destructive interference at a point means at that point, the intensity of light wave or simply wave is the minimum. This point is also called minima. Constructive interference at a point means at that point, the intensity of light wave or simply wave is the maximum. This point is also called maxima.
Formula Used:
Complete step-by-step answer:
The amount of intensity present at a point is the function of the phase difference at that point. If the phase difference between two waves is
Hence we can say, for destructive interference,
Now, as we know the relation between phase difference and path difference;
As
Or
Hence, we can say that for destructive interference, the path difference must be an odd multiple of
Diagram showing the condition when two waves undergo destructive interference.
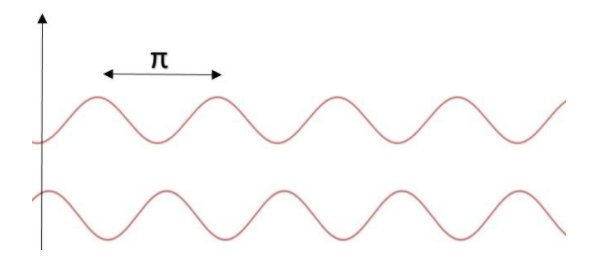
Here we can see that all the points on one wave have a phase difference of
Note: In the above diagram, we can see that the destructive interference occurs if every point on one wave has a phase difference of
Formula Used:
Complete step-by-step answer:
The amount of intensity present at a point is the function of the phase difference at that point. If the phase difference between two waves is
Hence we can say, for destructive interference,
Now, as we know the relation between phase difference and path difference;
As
Or
Hence, we can say that for destructive interference, the path difference must be an odd multiple of
Diagram showing the condition when two waves undergo destructive interference.

Here we can see that all the points on one wave have a phase difference of
Note: In the above diagram, we can see that the destructive interference occurs if every point on one wave has a phase difference of
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




