
Two charges of equal magnitude
A. will execute simple harmonic motion about equilibrium position
B. will be oscillating about equilibrium position but not executes simple harmonic motion
C. will not return back to the equilibrium position
D. will stop at equilibrium position
Answer
432k+ views
Hint:We can find the nature of the motion of the charge particle by using the concept of necessary conditions to execute simple harmonic motion , Coulomb’s law of and the nature of charge particles.
Formula used:
Electrostatic force between two charges
Where,
Complete step by step answer:
Let us consider two charges of equal magnitude
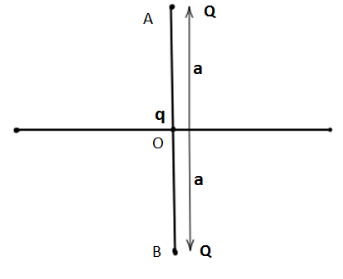
But, when the particle at O is displaced slightly (say
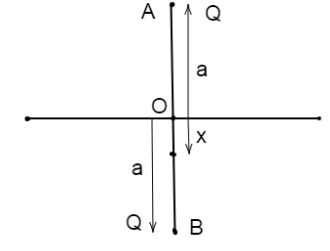
Solving we get,
We get ,
Hence, By the definition of simple harmonic motion, we can say that the particle
Hence, the correct answer is option A.
Note:We must know the concept of simple harmonic motion that restoring force acting on the particle should always be proportional to the displacement of the particle and towards the equilibrium position. Also, make the diagram and place charged particles properly as given in question.
Formula used:
Electrostatic force between two charges
Where,
Complete step by step answer:
Let us consider two charges of equal magnitude

But, when the particle at O is displaced slightly (say

Solving we get,
We get ,
Hence, By the definition of simple harmonic motion, we can say that the particle
Hence, the correct answer is option A.
Note:We must know the concept of simple harmonic motion that restoring force acting on the particle should always be proportional to the displacement of the particle and towards the equilibrium position. Also, make the diagram and place charged particles properly as given in question.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




