
Three charges 2q, −q, −q are located at the vertices of an equilateral triangle. At the centre of the triangle:
A. The field is zero but the potential is non – zero
B. The field is non – zero but the potential is zero
C. Both field and potential are zero
D. Both field and potential are non – zero
Answer
503.1k+ views
Hint: We are given an equilateral triangle and 2 charges placed at its vertices. We need to find the electric field and potential at the centre of the triangle. We know that for an equilateral triangle the distance from all vertices to centre will be the same. By using the respective equations, we can find the field and potential.
Formula used:
Complete answer:
In the question it is said that 3 charges 2q, -q and –q are placed at the three vertices of an equilateral triangle.
We are asked to determine the electric field and the potential at the centre of the triangle.
Consider the figure given below.
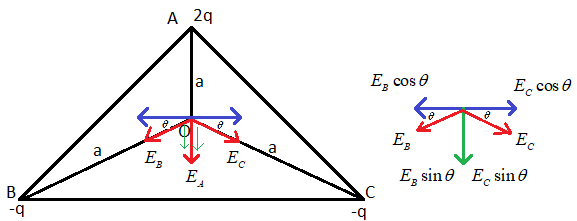
It shows the electric field at the centre of the equilateral triangle.
The charge 2q is placed at ‘A’, -q at ‘B’ and –q at C. ‘O’ is the centre of the triangle and ‘a’ is the distance from the vertices to the centre.
‘
‘
‘
The charge at ‘B’ and ‘C’ is –q.
We know that the electric field is given by the equation,
Therefore the field at ‘O’ due to ‘B’ will be,
And the charge at ‘O’ due to ‘C’ will be,
From this we can see that,
The components of ‘
From the figure we can see that
Therefore the remaining components of are
Therefore the net electric field experienced at the centre of the triangle,
Thus we can see that the electric field at the centre of the triangle is non – zero.
Now let us find the electric potential at the centre of the triangle. For that consider the figure given below.
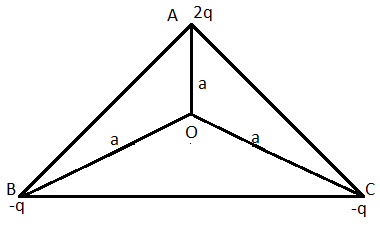
We know that the electric potential is given by the equation,
Here let
Therefore the net electric potential at the centre will be,
Thus we get the electric potential at the centre as zero.
Therefore we have the electric field at the centre of the triangle as non – zero and the electric potential at the centre of the triangle as zero.
So, the correct answer is “Option B”.
Note:
Electric field is a vector quantity, i.e. a quantity with both magnitude and direction. It is simply defined as the force per unit charge at point.
Mathematically we can write,
From coulomb’s law we have the equation for force as,
Therefore we get the electric field as,
Formula used:
Complete answer:
In the question it is said that 3 charges 2q, -q and –q are placed at the three vertices of an equilateral triangle.
We are asked to determine the electric field and the potential at the centre of the triangle.
Consider the figure given below.

It shows the electric field at the centre of the equilateral triangle.
The charge 2q is placed at ‘A’, -q at ‘B’ and –q at C. ‘O’ is the centre of the triangle and ‘a’ is the distance from the vertices to the centre.
‘
‘
‘
The charge at ‘B’ and ‘C’ is –q.
We know that the electric field is given by the equation,
Therefore the field at ‘O’ due to ‘B’ will be,
And the charge at ‘O’ due to ‘C’ will be,
From this we can see that,
The components of ‘
From the figure we can see that
Therefore the remaining components of are
Therefore the net electric field experienced at the centre of the triangle,
Thus we can see that the electric field at the centre of the triangle is non – zero.
Now let us find the electric potential at the centre of the triangle. For that consider the figure given below.

We know that the electric potential is given by the equation,
Here let
Therefore the net electric potential at the centre will be,
Thus we get the electric potential at the centre as zero.
Therefore we have the electric field at the centre of the triangle as non – zero and the electric potential at the centre of the triangle as zero.
So, the correct answer is “Option B”.
Note:
Electric field is a vector quantity, i.e. a quantity with both magnitude and direction. It is simply defined as the force per unit charge at point.
Mathematically we can write,
From coulomb’s law we have the equation for force as,
Therefore we get the electric field as,
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




