
The number of solutions for the equation
(a) 0
(b) 1
(c) 3
(d) 2
Answer
497.4k+ views
Hint: We solve this problem first by taking the trigonometric equations out by using the standard formulas that is
Then we use the composite angle formula of inverse trigonometric equations as
By using the above formulas we find the relation between to functions without trigonometric equations so that we can find the number of solutions using the graphs that is if
Complete step-by-step solution
We are given that the equation that is
We know that the inverse trigonometric relation that is
By using this relation to above equation we get
We know that the formula of the composite angle formula of inverse trigonometric equations as
By using this formula to above equation we get
We know that from the standard table of trigonometric ratios that is
By substituting this value in above equation we get
We know that if
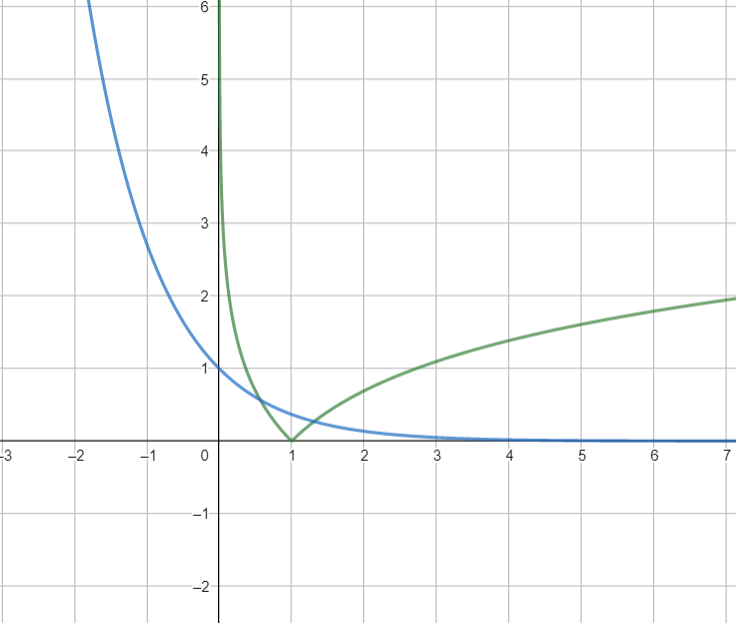
Now, let us draw the graph of
Here we can see that the blue line represents
Here, we can see that both the graphs intersect at 2 points therefore the number of solutions of the given equation is 2.
So, option (d) is the correct answer.
Note: We have a shortcut for solving the problem.
We are given that the equation as
We have a standard result of inverse trigonometric ratios that is if
By using the above result we get
Then we use the composite angle formula of inverse trigonometric equations as
By using the above formulas we find the relation between to functions without trigonometric equations so that we can find the number of solutions using the graphs that is if
Complete step-by-step solution
We are given that the equation that is
We know that the inverse trigonometric relation that is
By using this relation to above equation we get
We know that the formula of the composite angle formula of inverse trigonometric equations as
By using this formula to above equation we get
We know that from the standard table of trigonometric ratios that is
By substituting this value in above equation we get
We know that if
Now, let us draw the graph of
Here we can see that the blue line represents
Here, we can see that both the graphs intersect at 2 points therefore the number of solutions of the given equation is 2.
So, option (d) is the correct answer.

Note: We have a shortcut for solving the problem.
We are given that the equation as
We have a standard result of inverse trigonometric ratios that is if
By using the above result we get
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




