
The distance between the two charges
A. At a distance of
B. At a distance of
C. At a distance of
D. At a distance of
Answer
424.5k+ views
3 likes
Hint: To answer this question we will first define an electric field and give its formula. Later we will draw a diagram exactly as stated in the question. Next we will apply the formula for electric field for both charges and equate them with each other to find the answer.
Formula used:
Where,
Complete step by step answer:
Let us see what electric field intensity is: The electric field is the area around an electric charge where its impact can be felt. The force experienced by a unit positive charge put at a spot is the electric field intensity at that point.Look at the diagram:
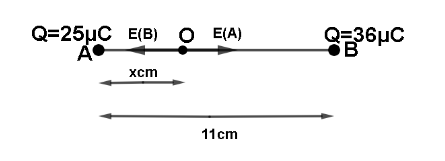
Let the charge at point A be
Let this be equation 1
Now we will find at point B:
Let this be equation 2
Now let us equate equations 1 and 2, we get:
Taking root on both sides
Hence the correct answer is that the intensity will be zero at a distance of
Hence the correct answer is option A.
Note: Students make a mistake while selecting a point where intensity is zero. In this question, to the left side of point A or right side of point B the intensity cannot be zero because at both these points intensities will add up as both are positive charges. Hence it will be zero only in between them.
Formula used:
Where,
Complete step by step answer:
Let us see what electric field intensity is: The electric field is the area around an electric charge where its impact can be felt. The force experienced by a unit positive charge put at a spot is the electric field intensity at that point.Look at the diagram:

Let the charge at point A be
Let this be equation 1
Now we will find at point B:
Let this be equation 2
Now let us equate equations 1 and 2, we get:
Taking root on both sides
Hence the correct answer is that the intensity will be zero at a distance of
Hence the correct answer is option A.
Note: Students make a mistake while selecting a point where intensity is zero. In this question, to the left side of point A or right side of point B the intensity cannot be zero because at both these points intensities will add up as both are positive charges. Hence it will be zero only in between them.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




