
The d electron configuration of
a.)
b.)
c.)
d.)
Answer
504.3k+ views
Hint: The paramagnetic species have unpaired electrons. Higher the number of unpaired electrons, more will be the magnetic moment or paramagnetic character, This can be calculated as -
Where n = number of unpaired electrons
Complete step by step answer :
First, let us understand what is paramagnetic behaviour and what are the conditions that are required to achieve it.
The paramagnetic are those species that have unpaired electrons. The first condition for a species to be paramagnetic is that it must have at least one free electron. When the magnet is placed near a paramagnetic species, the free electrons in the mass get aligned up in the direction of the magnet.
The paramagnetic behaviour in a species leads to the formation of the net magnetic moment. The magnetic moment can be calculated by the formula -
Where n = number of unpaired electrons
The water has charge 0 on it. So, all these metals are in +2 oxidation states.
So, let us see the electrons in 3d orbitals of these transition metals according to their electronic configurations which are given to us and calculate their magnetic moment.
So, the first species is Chromium with +2 charge. It has 4 electrons in 3d orbital. So, the filling will take place as-
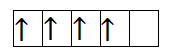
So, from the above filling, it is clear that there are four unpaired electrons. Thus, the magnetic moment is-
µ = 4.47 BM
Now, let us calculate for
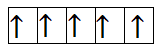
From the above filling, it is clear that there are five unpaired electrons. Thus, the magnetic moment is-
µ = 5.47 BM
Now, let us calculate for
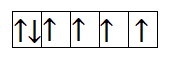
From the above filling, it is clear that there are four unpaired electrons. Thus, the magnetic moment is-
µ = 4.47 BM
Now, let us calculate for
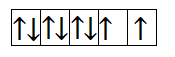
From the above filling, it is clear that there are two unpaired electrons. Thus, the magnetic moment is-
µ = 2.44 BM
So, observing all the values, we can say that
So, the option (d) is the correct answer.
Note: It must be noted that n is the number of unpaired electrons and not the total number of electrons in d orbital. Further, the water is a neutral ligand. It does not have any positive or negative charge. So, the total charge on a complex like here is +2 will be taken as the charge on the metal ion.
Where n = number of unpaired electrons
Complete step by step answer :
First, let us understand what is paramagnetic behaviour and what are the conditions that are required to achieve it.
The paramagnetic are those species that have unpaired electrons. The first condition for a species to be paramagnetic is that it must have at least one free electron. When the magnet is placed near a paramagnetic species, the free electrons in the mass get aligned up in the direction of the magnet.
The paramagnetic behaviour in a species leads to the formation of the net magnetic moment. The magnetic moment can be calculated by the formula -
Where n = number of unpaired electrons
The water has charge 0 on it. So, all these metals are in +2 oxidation states.
So, let us see the electrons in 3d orbitals of these transition metals according to their electronic configurations which are given to us and calculate their magnetic moment.
So, the first species is Chromium with +2 charge. It has 4 electrons in 3d orbital. So, the filling will take place as-

So, from the above filling, it is clear that there are four unpaired electrons. Thus, the magnetic moment is-
µ = 4.47 BM
Now, let us calculate for

From the above filling, it is clear that there are five unpaired electrons. Thus, the magnetic moment is-
µ = 5.47 BM
Now, let us calculate for

From the above filling, it is clear that there are four unpaired electrons. Thus, the magnetic moment is-
µ = 4.47 BM
Now, let us calculate for

From the above filling, it is clear that there are two unpaired electrons. Thus, the magnetic moment is-
µ = 2.44 BM
So, observing all the values, we can say that
So, the option (d) is the correct answer.
Note: It must be noted that n is the number of unpaired electrons and not the total number of electrons in d orbital. Further, the water is a neutral ligand. It does not have any positive or negative charge. So, the total charge on a complex like here is +2 will be taken as the charge on the metal ion.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




