
The area of triangle whose vertices are (1, 2, 3), (2, 5, −1) and (−1, 1, 2) is
150 sq. units
145 sq. units
Answer
534k+ views
1 likes
Hint: All three vertices of the triangle are given. We have to find the vector AB and AC which represents the side vectors. Using the concept of cross product, we have to find cross product of the vector AB and AC. By using the formula of area of triangle, we can simply find the area of triangle ABC. The formula of area of triangle is given below:
Area of triangle
Complete step-by-step solution -
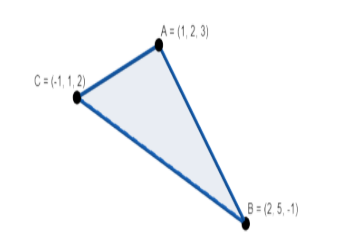
Given: The vertices of the triangle are:
A (1, 2, 3), B (2, 5, -1) and C (-1, 1, 2)
Consider the origin to be at (0, 0). Now,
Similarly, the
Then, the cross product of
The magnitude of a resultant vector
Now, by using the above-mentioned expression, the magnitude of both the vectors,
Now, area of the triangle ABC
Area of the triangle ABC
Hence, the area of the triangle ABC is
Therefore, option (c) is correct.
Note: The key concept involved in solving this problem is the expression of sides in the form of vectors. Students must be careful while calculating the cross product and must be aware of the intricacies of signs involved in cross product. General mistake is done in the calculation of cross product.
Area of triangle
Complete step-by-step solution -

Given: The vertices of the triangle are:
A (1, 2, 3), B (2, 5, -1) and C (-1, 1, 2)
Consider the origin to be at (0, 0). Now,
Similarly, the
Then, the cross product of
The magnitude of a resultant vector
Now, by using the above-mentioned expression, the magnitude of both the vectors,
Now, area of the triangle ABC
Area of the triangle ABC
Hence, the area of the triangle ABC is
Therefore, option (c) is correct.
Note: The key concept involved in solving this problem is the expression of sides in the form of vectors. Students must be careful while calculating the cross product and must be aware of the intricacies of signs involved in cross product. General mistake is done in the calculation of cross product.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




