
Show graphically, the binding energy per nucleon with the mass number and also explain how energy is released in the process of nuclear fission and nuclear fusion.
Answer
433.5k+ views
Hint:Initially, we will discuss the basic idea behind binding energy. Then, gradually we will discuss the stability of an atom with binding energy as a reference. Then we will discuss nuclear fusion and nuclear fission.
Complete answer:
Firstly, let us define binding energy. It can be simply defined as the amount of energy required to break a nucleus into its constituents. In simple words, it can be defined as the breaking energy needed to break a nucleus into individual neutrons and protons.
Let us take some examples; the binding energy for oxygen (
Similarly, the binding energy for Uranium (
Now, if we compare the binding energies of the two atoms, then, can we say that
Thus, we use the concept of binding energy per nucleon. This is a more comparable parameter as we are comparing energy required by each nucleon (neutron or proton) to get separated. For a nucleus, the value can be calculated as
Thus, substituting the values for
And for
Thus, it is apparent that
Now, the graph of binding energy per nucleon with the mass number turns out to be something like
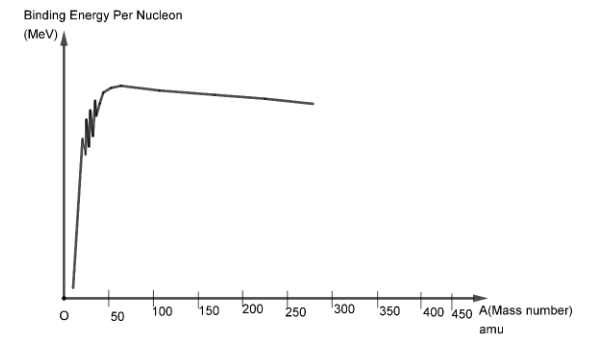
Here, we can see that the binding energy per nucleon initially increases with mass number.Then it reaches a peak value near
Now, let us discuss the energy released in nuclear fission and nuclear fusion. Nuclear fission is the process in which a heavy nucleus is split into two lighter nuclei (daughter nuclei).Whereas, nuclear fusion is the process in which two light nuclei are combined to form a heavier nucleus. In both cases the mass of the products is slightly less than mass of the initial nuclei. This mass difference is released as energy.
For example, when
Similarly, when deuterium (
Note:Students often get confused intuitively with the literary sense of the name binding energy as the energy required to bind the nucleons together. But the actual sense is the amount of energy required to break the binding between the nucleons. Students also commit errors thinking that energy has to be supplied for nuclear fission so no energy is released while a fission process is taking place. But in actuality, the nucleus is bombarded with a neutron for triggering the fission to take place. The neutron released after the fusion has a minimal mass so it is playing a very minor role in affecting the energy released during the fusion process.
Complete answer:
Firstly, let us define binding energy. It can be simply defined as the amount of energy required to break a nucleus into its constituents. In simple words, it can be defined as the breaking energy needed to break a nucleus into individual neutrons and protons.
Let us take some examples; the binding energy for oxygen (
Similarly, the binding energy for Uranium (
Now, if we compare the binding energies of the two atoms, then, can we say that
Thus, we use the concept of binding energy per nucleon. This is a more comparable parameter as we are comparing energy required by each nucleon (neutron or proton) to get separated. For a nucleus, the value can be calculated as
Thus, substituting the values for
And for
Thus, it is apparent that
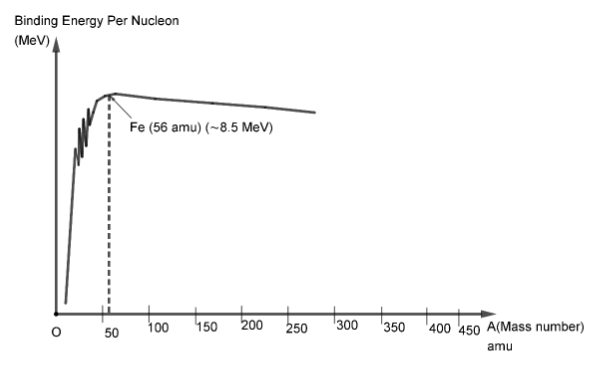
Now, the graph of binding energy per nucleon with the mass number turns out to be something like

Here, we can see that the binding energy per nucleon initially increases with mass number.Then it reaches a peak value near

Now, let us discuss the energy released in nuclear fission and nuclear fusion. Nuclear fission is the process in which a heavy nucleus is split into two lighter nuclei (daughter nuclei).Whereas, nuclear fusion is the process in which two light nuclei are combined to form a heavier nucleus. In both cases the mass of the products is slightly less than mass of the initial nuclei. This mass difference is released as energy.
For example, when
Similarly, when deuterium (
Note:Students often get confused intuitively with the literary sense of the name binding energy as the energy required to bind the nucleons together. But the actual sense is the amount of energy required to break the binding between the nucleons. Students also commit errors thinking that energy has to be supplied for nuclear fission so no energy is released while a fission process is taking place. But in actuality, the nucleus is bombarded with a neutron for triggering the fission to take place. The neutron released after the fusion has a minimal mass so it is playing a very minor role in affecting the energy released during the fusion process.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




