
Positive charge
Answer
494.7k+ views
Hint: We know that the electric force is force experience due multiple charges, and is given by Coulomb's force. It states that the electric force depends on the charge q and inversely proportional to the square of the distance between them. We can use the above to solve the given problem..
Formula:
Complete answer:
We know that the electric force is the force experienced due to a pair charges is given by Coulomb's force. Clearly, the electrical force between the charges, depends on the individual charge q and is inversely proportional to the square of the distance between them.
We know that, the electrical force
Similarly, an electric field E is defined as the electric force F per unit positive charge q, which is infinitesimally small and at rest, and is given as
Here, given that the
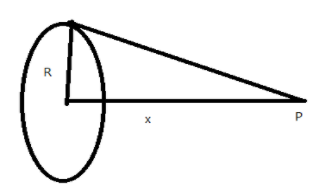
We know that the electric field due to a circular ring, of radius
Then the force at P is given as,
Let the amplitude of the electric field be
Note:
We know that the electric force due to a pair of charges is given by Coulomb's law. This force can be attractive or repelling depending on the nature of the charges. Electric field is in the direction of the force. However, the electric field also depends on the symmetry of the charge carrying conductor.
Formula:
Complete answer:
We know that the electric force is the force experienced due to a pair charges is given by Coulomb's force. Clearly, the electrical force between the charges, depends on the individual charge q and is inversely proportional to the square of the distance between them.
We know that, the electrical force
Similarly, an electric field E is defined as the electric force F per unit positive charge q, which is infinitesimally small and at rest, and is given as
Here, given that the

We know that the electric field due to a circular ring, of radius
Then the force at P is given as,
Let the amplitude of the electric field be
Note:
We know that the electric force due to a pair of charges is given by Coulomb's law. This force can be attractive or repelling depending on the nature of the charges. Electric field is in the direction of the force. However, the electric field also depends on the symmetry of the charge carrying conductor.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




