
Let a total charge 2Q be distributed in a sphere of radius R, with the charge density given by p(r) = kr, where r is the distance from the centre two charges A and B of –Q each are placed on diametrically opposite points, at equal distance a form the center. If A and B do not experience force, then:
Answer
475.2k+ views
3 likes
Hint: In order to find solution of this question force on A due to B must be equal to force on A due to sphere S to do so we have to find electric field on AB in order to do it we have to draw gauss’s surface inside a sphere S with radius r.
Formula used:
Complete answer:
In order to find a solution and equilibrium condition force on A due to B is equal to force on A due to S since A and B are at equal distance and have equal charge Q.
Now force on A due to B
k
Q = charge
a = radius or distance between two charges.
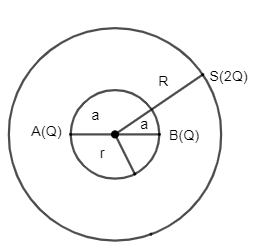
Force due to S and A
First we have to find electric field E we will take gauss’s surface to find electric field on A and B now,
Electric field is constant hence
Now we know that area of the radius r circle is
And it is given in question that
Now
Now we have to find k in order to do it we will use the below equation
Charge on whole sphere
Now substitute the value of k in equation (4)
From figure we can put r = a
Now put all the values in equation (1)
Hence the correct option is (c).
Note:
In this question we have to consider both the charge A and B inside the sphere if we consider them outside the sphere the solution could lead us to the wrong answer or solution.
Formula used:
Complete answer:
In order to find a solution and equilibrium condition force on A due to B is equal to force on A due to S since A and B are at equal distance and have equal charge Q.
Now force on A due to B
k
Q = charge
a = radius or distance between two charges.

Force due to S and A
First we have to find electric field E we will take gauss’s surface to find electric field on A and B now,
Electric field is constant hence
Now we know that area of the radius r circle is
And it is given in question that
Now
Now we have to find k in order to do it we will use the below equation
Charge on whole sphere
Now substitute the value of k in equation (4)
From figure we can put r = a
Now put all the values in equation (1)
Hence the correct option is (c).
Note:
In this question we have to consider both the charge A and B inside the sphere if we consider them outside the sphere the solution could lead us to the wrong answer or solution.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




