
Let A (3, 0, – 1), B (2, 10, 6) and C (1, 2, 1) be the vertices of a triangle and M be the midpoint of AC. If G divides BM in the ratio, 2:1, then
Answer
511.8k+ views
Hint: We are given M is the midpoint of AC. So, we get the line BM is the median as G is the point on BM on dividing it into 2:1. We will get by definition of the centroid that G is the centroid then the coordinate of G is given by
Complete step-by-step answer:
We are given the coordinates of the triangle ABC as A (3, 0, – 1), B (2, 10, 6) and C (1, 2, 1). We have M as the midpoint of AC and G is the point on BM that divides BM in the ratio 2:1. We know that as M is the midpoint, so BM is the median of triangle ABC. Now, the point on the median which divides the median in 2:1 is known as the centroid.
As G lies on the median BM and divides BM in the ratio 2:1, so it means G is the centroid.
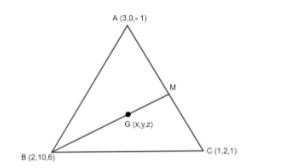
Now we know that coordinate of the centroid G is given as
where
As the vertex, we have A (3, 0, – 1), B (2, 10, 6), C (1, 2, 1). So, we get the coordinate of G as
So, we have the coordinate as G (2, 4, 2).
Now, we have to find the cos of
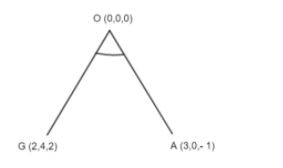
We know that the cos angle between the two vectors is given as
So, for
So, for G (2, 4, 2) and O (0, 0, 0) we have,
For A (3, 0, – 1) and O (0, 0, 0), we have,
Now,
And
Also,
Putting this value in
After simplification we get,
So, the correct answer is “Option C”.
Note: To simplify the square root we need to factorize it.
2 comes out two times as it makes a pain that gives us
Complete step-by-step answer:
We are given the coordinates of the triangle ABC as A (3, 0, – 1), B (2, 10, 6) and C (1, 2, 1). We have M as the midpoint of AC and G is the point on BM that divides BM in the ratio 2:1. We know that as M is the midpoint, so BM is the median of triangle ABC. Now, the point on the median which divides the median in 2:1 is known as the centroid.
As G lies on the median BM and divides BM in the ratio 2:1, so it means G is the centroid.

Now we know that coordinate of the centroid G is given as
where
As the vertex, we have A (3, 0, – 1), B (2, 10, 6), C (1, 2, 1). So, we get the coordinate of G as
So, we have the coordinate as G (2, 4, 2).
Now, we have to find the cos of

We know that the cos angle between the two vectors is given as
So, for
So, for G (2, 4, 2) and O (0, 0, 0) we have,
For A (3, 0, – 1) and O (0, 0, 0), we have,
Now,
And
Also,
Putting this value in
After simplification we get,
So, the correct answer is “Option C”.
Note: To simplify the square root we need to factorize it.
2 comes out two times as it makes a pain that gives us
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




