
Inside a uniform spherical shell
(This question has Multiple correct answers)
A. The gravitational potential is zero.
B. The gravitational field is zero.
C. The gravitational potential is the same everywhere.
D. The gravitational field is the same everywhere.
Answer
523.2k+ views
Hint: A uniform spherical shell has a constant surface mass density. We should take a point inside the spherical shell of radius R centered at O. Then find the potential at that particular point P. We can do this by taking a small cross-section of the spherical shell, finding the corresponding potential at point P due to that cross-section and finally integrate over the whole spherical shell to get the total potential at point P.
Formula Used:
The gravitational potential(V) of a mass M at a distance R away from it, is given by
Complete step-by-step answer:
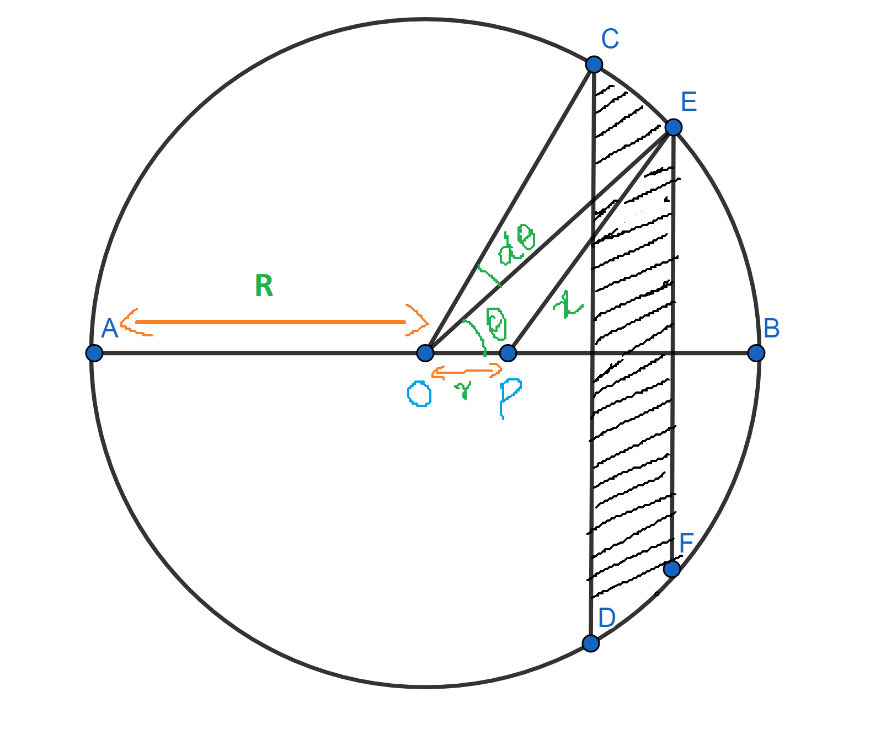
We will consider a spherical shell of radius R, having a constant surface mass density
Draw two planes CD and EF perpendicular to OP and very close to each other. The area in between these two planes will act as a ring of radius EG and width CE.
If
Mass of the ring can be calculated by multiplying the area of the ring with the surface mass density.
Since every point of the ring is at the same distance x from the point P, the small potential
In triangle OEP,
Differentiating the above equation, we get,
Substituting the above relation in equation (1), we get,
To obtain the potential V at P for the whole shell, we will integrate the above expression for dV between the limits
Integrating and applying the limits to the above integral we get,
We know that
This value is similar to the value of the potential at the surface of the shell. So we can conclude that the potential inside the spherical shell is constant.
The force acting on the point P can be found out by differentiating the potential at P by r.
Since the potential is a constant inside the shell, we will get,
So, the gravitational field inside the spherical shell is zero.
So, the answer to the question is both the options (B) and (C).
Note: The gravitational potential of a spherical shell of mass M and radius R at a distance r along the axis is given by,
The gravitational potential of a spherical shell of mass M and radius R on the surface of the shell is given by,
Formula Used:
The gravitational potential(V) of a mass M at a distance R away from it, is given by
Complete step-by-step answer:

We will consider a spherical shell of radius R, having a constant surface mass density
Draw two planes CD and EF perpendicular to OP and very close to each other. The area in between these two planes will act as a ring of radius EG and width CE.
If
Mass of the ring can be calculated by multiplying the area of the ring with the surface mass density.
Since every point of the ring is at the same distance x from the point P, the small potential
In triangle OEP,
Differentiating the above equation, we get,
Substituting the above relation in equation (1), we get,
To obtain the potential V at P for the whole shell, we will integrate the above expression for dV between the limits
Integrating and applying the limits to the above integral we get,
We know that
This value is similar to the value of the potential at the surface of the shell. So we can conclude that the potential inside the spherical shell is constant.
The force acting on the point P can be found out by differentiating the potential at P by r.
Since the potential is a constant inside the shell, we will get,
So, the gravitational field inside the spherical shell is zero.
So, the answer to the question is both the options (B) and (C).
Note: The gravitational potential of a spherical shell of mass M and radius R at a distance r along the axis is given by,
The gravitational potential of a spherical shell of mass M and radius R on the surface of the shell is given by,
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




