
In the circuit shown in figure, find the current through the branch BD.
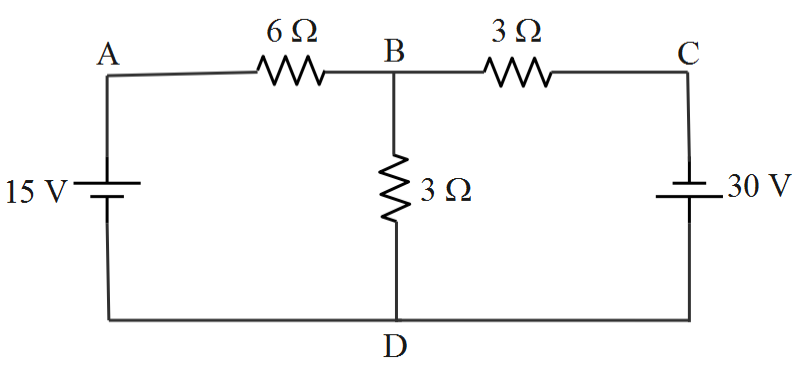
A.
B.
C.
D.

Answer
454.8k+ views
Hint: Use Kirchhoff’s Current Law at node B to obtain an expression for current through BD in terms of the currents due to the two supply voltages. Then, divide the circuit into two independent loops and apply Kirchhoff’s Voltage Law to each to arrive at the magnitude of currents supplied by the supply voltages. Use Ohm’s Law to find the voltage drops across the resistance elements. Then plug the values of these currents into the Current law expression to arrive at an appropriate value for current through the branch BD.
Formula Used:
Kirchhoff’s Current Law (KCL):
Kirchhoff’s Voltage Law (KVL):
Ohm’s law:
Complete answer:
We employ Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL) to determine the current through the branch BD.
Kirchhoff’s Current Law (KCL) suggests that the algebraic sum of currents entering a node in a circuit will be equal to the sum of currents leaving the node.
Kirchhoff’s Voltage Law (KVL) suggests that the sum of all voltages around any closed loop in a circuit must be equal to zero.
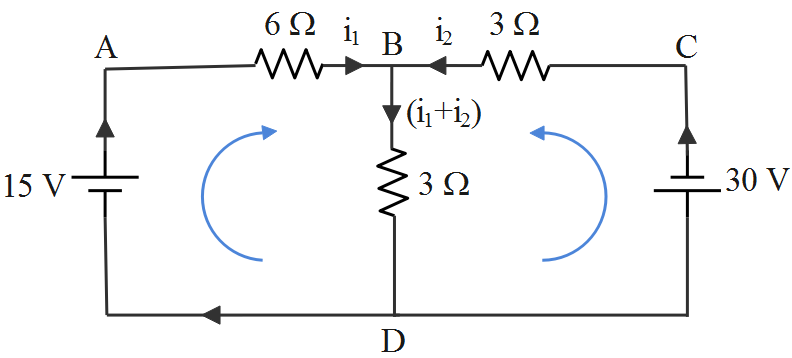
Let the current flowing through the circuit due to the
To find the current through BD, we first apply KCL at node B. We see that
Now, the voltage drops across the resistance elements can be found from Ohm’s law.
Now, we apply KVL to the two independent loops ABDA and BCDB deconstructed from our circuit.
For Loop ABDA:
For Loop BCDB:
Multiplying the above equation by 3 we get:
Solving (1) and (2) simultaneously we get:
Plugging the value of
From the KCL equation, we have:
So, the correct answer is “Option A”.
Note: Though we looked at KCL and KVL from a quantitative perspective, it is important to understand what they mean in a physical sense.
KCL signifies conservation of charge since the law basically suggests that the sum of currents entering a node must be equal to the sum of currents leaving the node, which means that electric charges are neither ambiguously lost nor mysteriously added but remains the same in an isolated system.
KVL signifies conservation of energy since the total energy in a system remains constant, though it may be transferred between components of the system in the form of electric potential and current.
Formula Used:
Kirchhoff’s Current Law (KCL):
Kirchhoff’s Voltage Law (KVL):
Ohm’s law:
Complete answer:
We employ Kirchhoff’s Current Law (KCL) and Kirchhoff’s Voltage Law (KVL) to determine the current through the branch BD.
Kirchhoff’s Current Law (KCL) suggests that the algebraic sum of currents entering a node in a circuit will be equal to the sum of currents leaving the node.
Kirchhoff’s Voltage Law (KVL) suggests that the sum of all voltages around any closed loop in a circuit must be equal to zero.

Let the current flowing through the circuit due to the
To find the current through BD, we first apply KCL at node B. We see that
Now, the voltage drops across the resistance elements can be found from Ohm’s law.
Now, we apply KVL to the two independent loops ABDA and BCDB deconstructed from our circuit.
For Loop ABDA:
For Loop BCDB:
Multiplying the above equation by 3 we get:
Solving (1) and (2) simultaneously we get:
Plugging the value of
From the KCL equation, we have:
So, the correct answer is “Option A”.
Note: Though we looked at KCL and KVL from a quantitative perspective, it is important to understand what they mean in a physical sense.
KCL signifies conservation of charge since the law basically suggests that the sum of currents entering a node must be equal to the sum of currents leaving the node, which means that electric charges are neither ambiguously lost nor mysteriously added but remains the same in an isolated system.
KVL signifies conservation of energy since the total energy in a system remains constant, though it may be transferred between components of the system in the form of electric potential and current.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




