Question

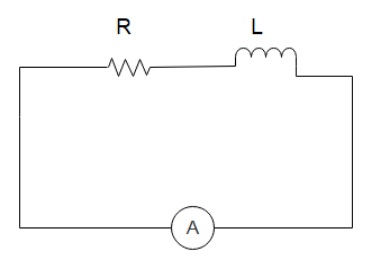
In the circuit shown, a 30 d.c. source gives a current 2 A as recorded in the ammeter A and 30 V a.c. source of frequency 100 Hz gives a current $ 1.2\,A $ . The inductive reactance is:

A) 10 ohm
B) 20 ohm
C) $ 5\sqrt {34} \,{\text{ohm}} $
D) 40 ohm

Answer 1

Hint: In this solution, we will first find the value of resistance of the circuit using ohm’s law. Then we will calculate the inductive reactance using the value of resistance and inductance given to us.

Formula used
In this solution, we will use the following formula
Voltage drop across an inductor: $ V = iR $ where $ i $ is the current and $ R $ is the resistance
Reactance of an a.c. LR circuit: $ I = \sqrt {{R^2} + X_L^2} $ where $ {X_L} $ is the inductive reactance.

Complete step by step answer:
In this circuit given to us, a 30 Volts d.c. the source generates a current of 2 A in the circuit. The current in the circuit when a d.c. voltage is supplied can be determined by ohm’s law as
 $ R = \dfrac{V}{i} $
Substituting the value of $ V = 30\,V $ and $ I = 2A $ , we get
 $ R = 15\,{\text{ohm}} $
When an a.c. the source is connected to the circuit, the reactance of the circuit can be determined by substituting the value of $ V = 30\,V $ and for a current of $ i = 1.2{\text{A}} $ as
 $ I = \dfrac{V}{i} $
Which gives us
 $ I = \dfrac{{30}}{{1.2}} = 25 $
Now that we know the resistance, we can calculate the reactance of the circuit as
 $ I = \sqrt {{R^2} + X_L^2} $
 $ 25 = \sqrt {{{15}^2} + X_L^2} $
Taking the square on both sides, we get
 $ 625 = 225 - X_L^2 $
Which gives us
 $ {X_L} = 20\,\Omega $
Which corresponds to option (B).

Note:
The resistance that we calculated when the DC power supply is attached and is only valid for circuits that have been in that stage for a long time. Only in this situation will the inductor not play any role in the circuit as it will be short-circuited. When an a.c. power supply is attached, the inductor will keep on resisting the change in the circuit and the current we calculated is the average current in the circuit as the current changes with time.