
If f(x) is an odd function, then |f(x)| is
[a] An odd function
[b] An even function
[c] Neither even nor odd
[d] Even and odd
Answer
523.8k+ views
Hint: Use the fact that the absolute value of -x is x. Recall the definitions of odd function and even function and check which of the definitions does the function follow. For an odd function, we know that f(-x) = -f(x) for all values of x in the domain of the function Hence find whether |f(x)| is an odd or even function. Verify with a known odd function, e.g. sinx.
Complete step-by-step solution -
Before solving the question, we need to understand what an odd function is and what an even function is.
Odd function: A function f(x) is said to be an odd function if
Even function: A function f(x) is said to be an even function if
Also, we have
Hence, we have
|-x| = |x|
Now, we have
H(x) = |f(x)|
H(-x) = |f(-x)|
Since f(x) is odd function, we have f(-x) = -f(x)
Hence, we have
H(-x) = |-f(x)|
Since |-x| = x, we have
H(-x) = |f(x)|.
Hence, we have H(-x) = H(x)
Hence, H(x) is even function (From the definition of an even function)
Hence option [b] is correct.
Note: Alternative Solution:
We use graphical transformations to determine the nature of |f(x)| when f(x) is odd.
Transformation Rule: If we know the graph of f(x), then the graph of |f(x)| can be drawn by taking simply the mirror image of the graph of f(x) below x-axis about the y-axis.
Since the graph of an odd function is symmetrical about the origin, a typical form of an odd function is as shown below
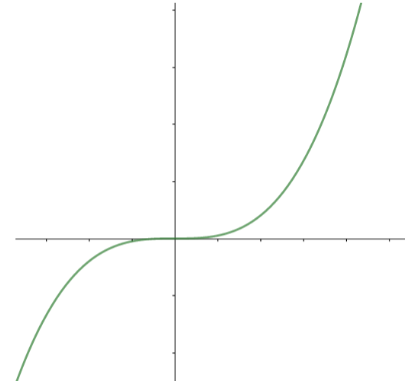
Using the above transformation rule, we draw the graph of |f(x)| as follows
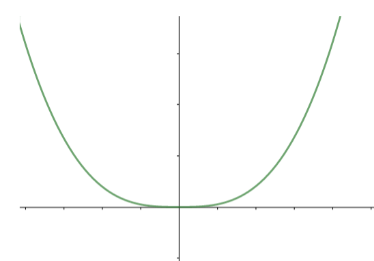
Clearly, the graph is symmetric about the y-axis.
Hence |f(x)| is even.
It, however, should be noted that this not the proof of the question rather a faster way of realisation of the above result.
Complete step-by-step solution -
Before solving the question, we need to understand what an odd function is and what an even function is.
Odd function: A function f(x) is said to be an odd function if
Even function: A function f(x) is said to be an even function if
Also, we have
Hence, we have
|-x| = |x|
Now, we have
H(x) = |f(x)|
H(-x) = |f(-x)|
Since f(x) is odd function, we have f(-x) = -f(x)
Hence, we have
H(-x) = |-f(x)|
Since |-x| = x, we have
H(-x) = |f(x)|.
Hence, we have H(-x) = H(x)
Hence, H(x) is even function (From the definition of an even function)
Hence option [b] is correct.
Note: Alternative Solution:
We use graphical transformations to determine the nature of |f(x)| when f(x) is odd.
Transformation Rule: If we know the graph of f(x), then the graph of |f(x)| can be drawn by taking simply the mirror image of the graph of f(x) below x-axis about the y-axis.
Since the graph of an odd function is symmetrical about the origin, a typical form of an odd function is as shown below

Using the above transformation rule, we draw the graph of |f(x)| as follows

Clearly, the graph is symmetric about the y-axis.
Hence |f(x)| is even.
It, however, should be noted that this not the proof of the question rather a faster way of realisation of the above result.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




