
Four equal point charges each of
Answer
486.3k+ views
2 likes
Hint: We are asked here to find the force on any of the charges on the corners of the square. Here, you will need to use coulomb’s law to find the force between two charges. Use this law to find the force exerted on any one point charge due to the other three charges. Then find the resultant force exerted on a point charge.
Complete step by step answer:
Given, charge on four corners of a square is,
Side of the square,
Here, we will use coulomb’s law, which states force between two charges is given as,
where
Let ABCD be the square with side
We draw the diagram with the given information.
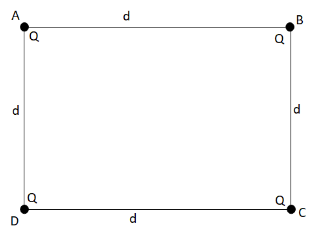
We are asked to calculate the force on any of the charges. The force on any of the charges will be the same.
Let us calculate the force on point A. We will now see the force exerted by the other three points on point A.Force exerted by point B on point A, using coulomb’s law from equation (i) we get,
force exerted by point D on point A, using coulomb’s law from equation (i) we get,
To find the force exerted by point C on point A, we need to find the distance between point A and C that is the length of the diagonal of the square.
We have the formula for length of diagonal of a square as,
Here side of the square is
We redraw the diagram using this length of the diagonal.
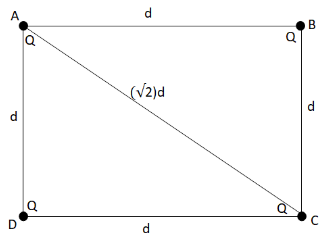
Now, force exerted by point C on point A using coulomb’s law from equation (i), we get
Now, we can find the total force exerted on point A by adding the force exerted by the other three points.
Now, we draw the diagram with the forces
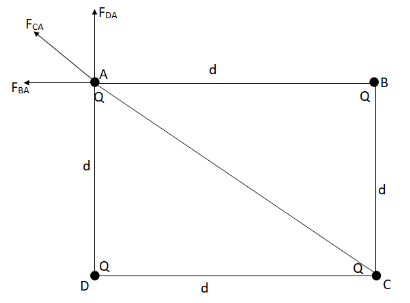
The resultant of forces
Putting the values of
The forces
Putting the values of
Now, putting the values of
Therefore, the force on any charge will be
Note:We have used the coulomb’s law. Coulomb states that force between two charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between the centers of the two charges. If the distance between the charges is increased the force between them reduces and if the distance between the charges is reduced the force between them increases.
Complete step by step answer:
Given, charge on four corners of a square is,
Side of the square,
Here, we will use coulomb’s law, which states force between two charges is given as,
where
Let ABCD be the square with side
We draw the diagram with the given information.

We are asked to calculate the force on any of the charges. The force on any of the charges will be the same.
Let us calculate the force on point A. We will now see the force exerted by the other three points on point A.Force exerted by point B on point A, using coulomb’s law from equation (i) we get,
force exerted by point D on point A, using coulomb’s law from equation (i) we get,
To find the force exerted by point C on point A, we need to find the distance between point A and C that is the length of the diagonal of the square.
We have the formula for length of diagonal of a square as,
Here side of the square is
We redraw the diagram using this length of the diagonal.

Now, force exerted by point C on point A using coulomb’s law from equation (i), we get
Now, we can find the total force exerted on point A by adding the force exerted by the other three points.
Now, we draw the diagram with the forces

The resultant of forces
Putting the values of
The forces
Putting the values of
Now, putting the values of
Therefore, the force on any charge will be
Note:We have used the coulomb’s law. Coulomb states that force between two charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between the centers of the two charges. If the distance between the charges is increased the force between them reduces and if the distance between the charges is reduced the force between them increases.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




