
Find the voltage
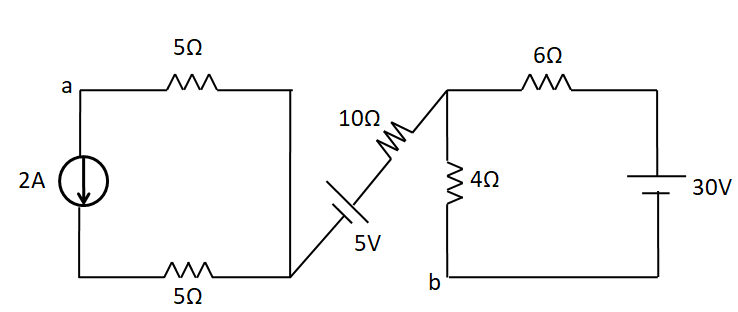
(A)
(B)
(C)
(D)

Answer
490.2k+ views
Hint
To solve this question, start from the initial potential point and reach to the final potential point through any path. While travelling through a path, write all the potential drops and gains which come in between the path.
Complete step by step answer
As in the first mesh, the current source of
We assume a current of
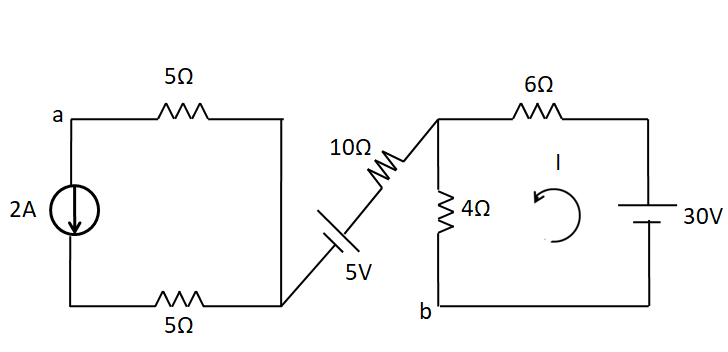
Applying KVL in the second mesh, we get
Dividing both the sides by
The current in the branch containing the resistance
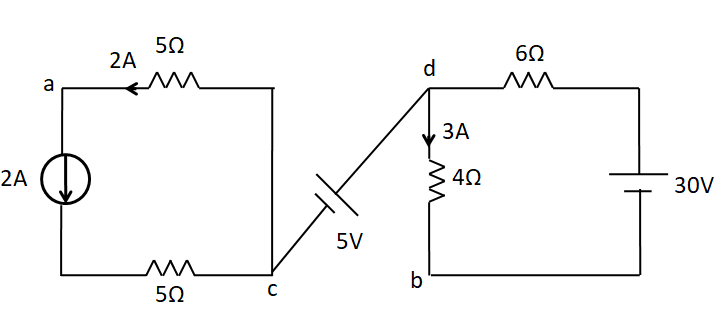
Now, for finding
On rearranging the terms, we get
Or,
Thus, the voltage
Hence, the correct answer is option B.
Note
Do not apply KVL along the path, in between where a current source is there. This is because the potential difference across a current source is unknown to us. Do not assume it to be zero. It can only be found out analytically. So, applying KVL along the path containing a current source will not be possible.
To solve this question, start from the initial potential point and reach to the final potential point through any path. While travelling through a path, write all the potential drops and gains which come in between the path.
Complete step by step answer
As in the first mesh, the current source of
We assume a current of

Applying KVL in the second mesh, we get
Dividing both the sides by
The current in the branch containing the resistance

Now, for finding
On rearranging the terms, we get
Or,
Thus, the voltage
Hence, the correct answer is option B.
Note
Do not apply KVL along the path, in between where a current source is there. This is because the potential difference across a current source is unknown to us. Do not assume it to be zero. It can only be found out analytically. So, applying KVL along the path containing a current source will not be possible.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




