
Find the value of trigonometric expression
Answer
531.9k+ views
Hint:Write the angle inside the trigonometric function given in problem to acute trigonometric relation. i.e. between
Complete step-by-step answer:
The given expression in the problem is
Let us suppose the value of the given expression is ‘M’, So, we can write equation as –
Now, we can observe that the angle involved in the above expression is not lying in
Hence, Let us divide the given expression by
Now, as we know the radian representation of
And with the help of above relation, we can re-write the angle
Hence, we can write the equation (ii) as –
Now, we can re-write the given expression in the problem i.e. equation (i) as –
Now, as we know the quadrant angles are defined as
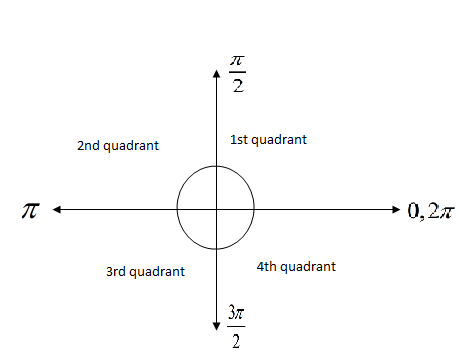
Now, we can apply the trigonometric rules for conversion of trigonometric expressions by changing its angle. We can observe the angle in equation (iv) and hence get that the angle
So, we know that tan function is negative in the 4th quadrant and we know very well that the angle involved in the equation (iv) is
So, we can write trigonometric identity as –
Hence, using the equation (v), we can get the equation (iv) as –
Now, we know the value of tan
M=-1
Hence, the value of the given expression in the problem is -1. So, we get –
So, ‘
Note: Don’t go for calculating the exact value directly with any other identity, it will be a complex approach and may give wrong answers as well. So, always try to solve these kinds of questions by the way given in solution.One can apply the trigonometric identity with the negative expression directly without changing the angles in radian form. It is done in the solution because we are more familiar with the trigonometric relation given in solution in radian form only.So, don’t confuse with that part of the solution, you can evaluate the given expression by using degree form of angle as well.
Complete step-by-step answer:
The given expression in the problem is
Let us suppose the value of the given expression is ‘M’, So, we can write equation as –
Now, we can observe that the angle involved in the above expression is not lying in
Hence, Let us divide the given expression by
Now, as we know the radian representation of
And with the help of above relation, we can re-write the angle
Hence, we can write the equation (ii) as –
Now, we can re-write the given expression in the problem i.e. equation (i) as –
Now, as we know the quadrant angles are defined as

Now, we can apply the trigonometric rules for conversion of trigonometric expressions by changing its angle. We can observe the angle in equation (iv) and hence get that the angle
So, we know that tan function is negative in the 4th quadrant and we know very well that the angle involved in the equation (iv) is
So, we can write trigonometric identity as –
Hence, using the equation (v), we can get the equation (iv) as –
Now, we know the value of tan
M=-1
Hence, the value of the given expression in the problem is -1. So, we get –
So, ‘
Note: Don’t go for calculating the exact value directly with any other identity, it will be a complex approach and may give wrong answers as well. So, always try to solve these kinds of questions by the way given in solution.One can apply the trigonometric identity with the negative expression directly without changing the angles in radian form. It is done in the solution because we are more familiar with the trigonometric relation given in solution in radian form only.So, don’t confuse with that part of the solution, you can evaluate the given expression by using degree form of angle as well.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




