
How do you find the centre, vertices and foci of an ellipse
Answer
482.1k+ views
Hint:
Here we need to compare the general equation of the ellipse which is
Complete step by step solution:
Here we are given the ellipse whose equation is
If we compare both the equations we will get the values of
We can draw the ellipse as:
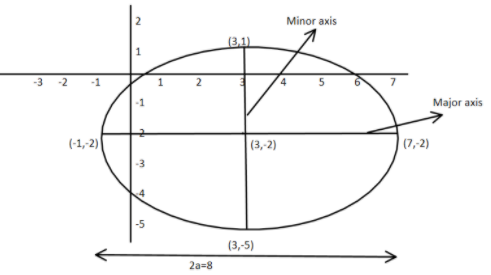
Now we can see that the centre of the ellipse is written as
So on comparing we can write given ellipse as:
So we can say that the centre of the ellipse is
Now we must know that it is the horizontal ellipse as its major axis is greater in length than the minor axis as
Now we can say that there will be
On the major axis the vertices are written as
So we can say:
Vertices on major axis
Vertices on minor axis:
Now to calculate foci we must know that foci for the horizontal ellipse is given by:
Coordinate
So we can substitute the values and get:
Foci
Note:
Here in these types of problems the student must try to draw the figure according to the type of ellipse that is given as this makes the understanding level of the question clearer. One must know all the general equations of the ellipse and also the way to calculate all the terms using the comparison method.
Here we need to compare the general equation of the ellipse which is
Complete step by step solution:
Here we are given the ellipse whose equation is
If we compare both the equations we will get the values of
We can draw the ellipse as:

Now we can see that the centre of the ellipse is written as
So on comparing we can write given ellipse as:
So we can say that the centre of the ellipse is
Now we must know that it is the horizontal ellipse as its major axis is greater in length than the minor axis as
Now we can say that there will be
On the major axis the vertices are written as
So we can say:
Vertices on major axis
Vertices on minor axis:
Now to calculate foci we must know that foci for the horizontal ellipse is given by:
Coordinate
So we can substitute the values and get:
Foci
Note:
Here in these types of problems the student must try to draw the figure according to the type of ellipse that is given as this makes the understanding level of the question clearer. One must know all the general equations of the ellipse and also the way to calculate all the terms using the comparison method.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




