
Find the area bounded by
Answer
504k+ views
1 likes
Hint: In this question, we have to find out the bounded region for the particular given. First we need to find the equation of the tangent with the help of the given equation and then using the formula for a bounded region we will get the area bounded by the given particulars.
Formula used: To find the slope m of a curve at a particular point, we differentiate the equation of the curve. If the given curve is y=f(x) we evaluate
Then the tangent at
Differentiation formula:
The area under a curve between two points can be found by doing a definite integral between the two points. To find the area under the curve y = f(x) between x = a and x = b, integrate y = f(x) between the limits of a and b. i.e.
Complete step-by-step answer:
We have to find out the area bounded by
First we need to find out the tangent line.
Here x=
Thus by substituting the value of x in the given equation we get,
By solving the above equation we get,
Let us now differentiate the given curve with respect to x, we have,
Hence the slope of the tangent line is
By substituting the slope in the equation of tangent we get
The equation of the tangent
On solving the above equation we get,
On further solving we find the equation with respect to y,
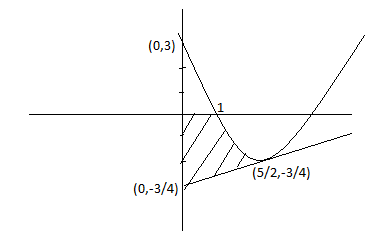
The above graph represents the area to be founded.
Therefore the area bounded by
Let us solve the equation inside the integral we get,
The area bounded by the given curve
Let us now integrate the above equation we get,
Let us substitute the upper and lower limit we get,
The area bounded by the curve
On further solving we get,
The area bounded by the given curve=
Hence, the area bounded by
Additional Information: To determine the equation of a tangent to a curve:
Find the derivative using the rules of differentiation.
Substitute the x-coordinate of the given point into the derivative to calculate the gradient of the tangent.
Substitute the gradient of the tangent and the coordinates of the given point into an appropriate form of the straight line equation that is
Note: We should consider the given point to find the corresponding point because it will lead us to the equation of the tangent line. Suppose we do not find the corresponding point we cannot find the equation of tangent which will not lead to a bound for the given equation.
Formula used: To find the slope m of a curve at a particular point, we differentiate the equation of the curve. If the given curve is y=f(x) we evaluate
Then the tangent at
Differentiation formula:
The area under a curve between two points can be found by doing a definite integral between the two points. To find the area under the curve y = f(x) between x = a and x = b, integrate y = f(x) between the limits of a and b. i.e.
Complete step-by-step answer:
We have to find out the area bounded by
First we need to find out the tangent line.
Here x=
Thus by substituting the value of x in the given equation we get,
By solving the above equation we get,
Let us now differentiate the given curve with respect to x, we have,
Hence the slope of the tangent line is
By substituting the slope in the equation of tangent we get
The equation of the tangent
On solving the above equation we get,
On further solving we find the equation with respect to y,

The above graph represents the area to be founded.
Therefore the area bounded by
Let us solve the equation inside the integral we get,
The area bounded by the given curve
Let us now integrate the above equation we get,
Let us substitute the upper and lower limit we get,
The area bounded by the curve
On further solving we get,
The area bounded by the given curve=
Hence, the area bounded by
Additional Information: To determine the equation of a tangent to a curve:
Find the derivative using the rules of differentiation.
Substitute the x-coordinate of the given point into the derivative to calculate the gradient of the tangent.
Substitute the gradient of the tangent and the coordinates of the given point into an appropriate form of the straight line equation that is
Note: We should consider the given point to find the corresponding point because it will lead us to the equation of the tangent line. Suppose we do not find the corresponding point we cannot find the equation of tangent which will not lead to a bound for the given equation.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




