
Find the area bounded by
A) 2
B) 4
C) 6
D) 8
Answer
487.5k+ views
Hint:
Here, we will use the concept of integration to find the area bounded by the curves. First we will find the point of inflection and then integrate the curves to find the area. Integration is the process of adding small areas to find the whole area.
Formula Used: Area between two curves which intersect each other at
Complete step by step solution:
We are given with a function
Replacing the function
Here,
Differentiating with respect to
Again differentiating with respect to
We know that
Therefore,
So, we have
Also
Using these we can draw a graph as shown below:
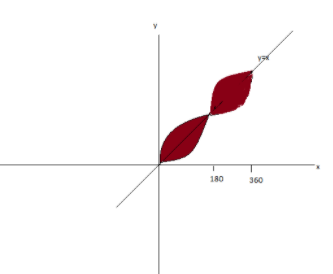
Area between two curves which intersect each other at
Now,
Area bounded by
Inverse function is symmetrical with respect to
Thus by using the property of symmetry, we get
Substituting the function
Subtracting the terms in the integrand, we get
Integrating the function, we get
Substituting the upper limit and lower limit, we get
Adding and multiplying, we get
Adding the terms, we get
Therefore, the area bounded by
Note:
We need to keep in mind that, while doing integration, we should notice from the graph the curve at the bottom should be subtracted from the curve at the top. We should also know that the inflection point is a point where a function changes its sign and its direction. We should remember that the function has to be differentiated twice to find the inflection points. The limits of the integral become when both the curves are symmetrical to each other.
Here, we will use the concept of integration to find the area bounded by the curves. First we will find the point of inflection and then integrate the curves to find the area. Integration is the process of adding small areas to find the whole area.
Formula Used: Area between two curves which intersect each other at
Complete step by step solution:
We are given with a function
Replacing the function
Here,
Differentiating with respect to
Again differentiating with respect to
We know that
Therefore,
So, we have
Also
Using these we can draw a graph as shown below:

Area between two curves which intersect each other at
Now,
Area bounded by
Inverse function is symmetrical with respect to
Thus by using the property of symmetry, we get
Substituting the function
Subtracting the terms in the integrand, we get
Integrating the function, we get
Substituting the upper limit and lower limit, we get
Adding and multiplying, we get
Adding the terms, we get
Therefore, the area bounded by
Note:
We need to keep in mind that, while doing integration, we should notice from the graph the curve at the bottom should be subtracted from the curve at the top. We should also know that the inflection point is a point where a function changes its sign and its direction. We should remember that the function has to be differentiated twice to find the inflection points. The limits of the integral become when both the curves are symmetrical to each other.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE




