Answer
417.6k+ views
Hint: Follow the rule of division method for finding the square root of any number. Put bars from the unit place and use two digits for one bar. Now think of the largest number whose square is even to just less than the first bar digit in the number. Now subtract and bring down the quotient to the divisor, now add a new digit in quotient and divisor (in front) and repeat it to get the quotient. At last when the remainder becomes 0, quotient is the square root of it.
Complete step-by-step solution -
Division method for finding square root can be given as
(a) First place a bar over every pair of digits starting from unit digit, if the number of digits are odd then the left most single digit will also have a bar.
(b) Think of the largest number whose square is even to first less than the first bar digit. Take this number as divisor and also as quotient.
(c) Next subtract the product of the divisor and the quotient from the first bar digit and bring down the next pair of digits which have a bar to the right side of the remainder, that becomes a new dividend.
(d) Now, the new divisor is obtained by adding the first divisor and the quotient and add a digit to the right side of it that we have to choose according to the new dividend which is chosen in such a way that product of new divisor and this digit is less than or equal to the new dividend.
(e) Repeat steps (b, c, d) till the bar digit has been taken up. Now quotient is the required square root of the given number.
Now, we need to find the square root of 7.1289 by the above rules of division method. So, we can put bars to the given number from the last digit in pairs. So, we get number 7.1283 as $\overline{7}.\overline{12}\overline{89}$ (after putting bars as per the rule).
Now, we have a single digit number ‘7’ and hence we need to find any number such that the square of that number is just less than 7. So, we know
${{2}^{2}}<7<{{3}^{2}}$
Hence, divide the number $\overline{7}.\overline{12}\overline{89}$ by 2 and put quotient ‘2’ as well in the following way:
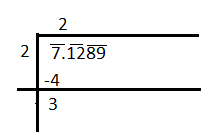
Now, add the quotient ‘2’ to the number 2 as per the steps. So, we get
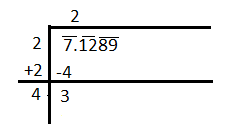
Now, put the decimal after the number 2 in the quotient and put ‘12’ adjacent to 3. So, we get
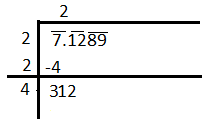
Now, as per the rules, we need to put a number in the divisor part (i.e. adjacent to 4) and the same number in the quotient as well such that multiplication of the number in the divisor and the digit in the quotient is just less than or equal to the number 312. So, we can observe that
$\begin{align}
& 46\times 6=276 \\
& 47\times 7=329 \\
\end{align}$
So, we need to put 6 to the adjacent ‘4’. So, we get
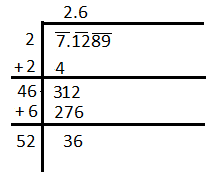
Now, in the similar way, put 89 (whole number) adjacent to 36 and divide 3689 with putting a digit adjacent to 52 and same digit to the quotient as well. So, we get

Hence, the square root of $\overline{7}.\overline{12}\overline{89}$ or 7.1289 is the quotient in the division method i.e. 2.67. Hence, 2.67 is the answer.
Note: Don’t put bars from the starting of a number in the division method. It is a general mistake done by students with this method. Always put the same digit in divisor and quotient. They should be the same for getting the square root. Bars should be only on maximum two digits and minimum on one digit.
One can verify the square root by calculating it by prime factorization method.
Complete step-by-step solution -
Division method for finding square root can be given as
(a) First place a bar over every pair of digits starting from unit digit, if the number of digits are odd then the left most single digit will also have a bar.
(b) Think of the largest number whose square is even to first less than the first bar digit. Take this number as divisor and also as quotient.
(c) Next subtract the product of the divisor and the quotient from the first bar digit and bring down the next pair of digits which have a bar to the right side of the remainder, that becomes a new dividend.
(d) Now, the new divisor is obtained by adding the first divisor and the quotient and add a digit to the right side of it that we have to choose according to the new dividend which is chosen in such a way that product of new divisor and this digit is less than or equal to the new dividend.
(e) Repeat steps (b, c, d) till the bar digit has been taken up. Now quotient is the required square root of the given number.
Now, we need to find the square root of 7.1289 by the above rules of division method. So, we can put bars to the given number from the last digit in pairs. So, we get number 7.1283 as $\overline{7}.\overline{12}\overline{89}$ (after putting bars as per the rule).
Now, we have a single digit number ‘7’ and hence we need to find any number such that the square of that number is just less than 7. So, we know
${{2}^{2}}<7<{{3}^{2}}$
Hence, divide the number $\overline{7}.\overline{12}\overline{89}$ by 2 and put quotient ‘2’ as well in the following way:
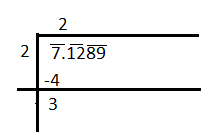
Now, add the quotient ‘2’ to the number 2 as per the steps. So, we get
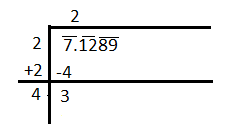
Now, put the decimal after the number 2 in the quotient and put ‘12’ adjacent to 3. So, we get
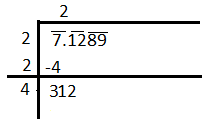
Now, as per the rules, we need to put a number in the divisor part (i.e. adjacent to 4) and the same number in the quotient as well such that multiplication of the number in the divisor and the digit in the quotient is just less than or equal to the number 312. So, we can observe that
$\begin{align}
& 46\times 6=276 \\
& 47\times 7=329 \\
\end{align}$
So, we need to put 6 to the adjacent ‘4’. So, we get
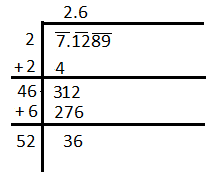
Now, in the similar way, put 89 (whole number) adjacent to 36 and divide 3689 with putting a digit adjacent to 52 and same digit to the quotient as well. So, we get

Hence, the square root of $\overline{7}.\overline{12}\overline{89}$ or 7.1289 is the quotient in the division method i.e. 2.67. Hence, 2.67 is the answer.
Note: Don’t put bars from the starting of a number in the division method. It is a general mistake done by students with this method. Always put the same digit in divisor and quotient. They should be the same for getting the square root. Bars should be only on maximum two digits and minimum on one digit.
One can verify the square root by calculating it by prime factorization method.
Recently Updated Pages
The branch of science which deals with nature and natural class 10 physics CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Define absolute refractive index of a medium

Find out what do the algal bloom and redtides sign class 10 biology CBSE

Prove that the function fleft x right xn is continuous class 12 maths CBSE

Find the values of other five trigonometric functions class 10 maths CBSE

Trending doubts
Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Write an application to the principal requesting five class 10 english CBSE

Difference Between Plant Cell and Animal Cell

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What organs are located on the left side of your body class 11 biology CBSE

Discuss what these phrases mean to you A a yellow wood class 9 english CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE



