
How do you find an equation of a parabola given endpoints of the latus rectum are
Answer
473.7k+ views
Hint: We will start by plotting an appropriate figure parabola of the given endpoints. We have the endpoints of the latus rectum, using these points we can calculate the focus of the parabola using the midpoint formula. Using the point of focus, we can calculate the vertex of the parabola, but since we do not know as in which direction is the parabola open, so we have two equations of parabola for parabola open upwards and downwards as well.
Complete step by step solution:
According to the given question, we have been given the endpoints of the latus rectum and we have to find an equation of the parabola having the latus rectum with the given endpoints.
Firstly, we will plot the given point and draw the possible parabola’s which can be drawn, we have,
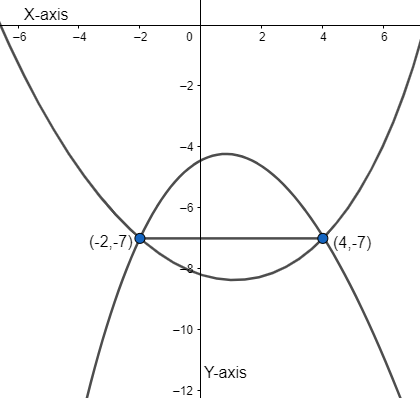
The above plot has two parabolas since we are not given in which the parabola opens. So, using the endpoints given we can get two equations of parabola.
We know that the equation of parabola along the y-axis is
But these equations were valid as the vertex of the parabola was at the origin, but now we cannot use the above equation as the vertex of the parabolas of this question would be a point other than the origin, so we will use the formula:
And
where
Latus rectum refers to the line (parallel to the x-axis in the current case) which passes through a focus and its length is 4 times the length from the vertex to the midpoint of the latus rectum.
So as to find the equation of the parabola we have to find the vertex of the parabolas.
We will find the focus of the parabola using the midpoint formula, we have,
Given endpoints of the latus rectum are:
We get the focus as,
Focus
Focus
Now, we have to find the length of the latus rectum using the endpoints of the latus rectum are:
Distance =
(As stated above, we know that the length of latus rectum is 4a. Therefore, we have,)
and
The value of ‘h’ in the vertex of a parabola has the same value as that of x-coordinate of the focus
That is,
We know that,
The vertex is
and
The vertex is
Therefore, substituting the values of all the variables we obtained we have,
the equation of the parabola opening upwards and having vertex
And
the equation of the parabola opening downwards and having vertex
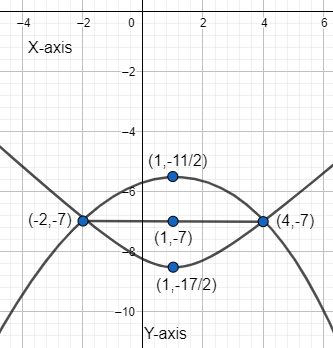
So, we have the graph as,
Note:
While solving for the focus of the parabola, the focus should not be intermixed with the vertex of the parabola. Both are two different terms. And the equation for parabola opening upwards and downwards differ only by the presence of a negative sign rest everything is the same. Similarly, the equation to find the value of y component of the vertex varies in sign with parabola facing upwards and downwards.
Complete step by step solution:
According to the given question, we have been given the endpoints of the latus rectum and we have to find an equation of the parabola having the latus rectum with the given endpoints.
Firstly, we will plot the given point and draw the possible parabola’s which can be drawn, we have,

The above plot has two parabolas since we are not given in which the parabola opens. So, using the endpoints given we can get two equations of parabola.
We know that the equation of parabola along the y-axis is
But these equations were valid as the vertex of the parabola was at the origin, but now we cannot use the above equation as the vertex of the parabolas of this question would be a point other than the origin, so we will use the formula:
And
where
Latus rectum refers to the line (parallel to the x-axis in the current case) which passes through a focus and its length is 4 times the length from the vertex to the midpoint of the latus rectum.
So as to find the equation of the parabola we have to find the vertex of the parabolas.
We will find the focus of the parabola using the midpoint formula, we have,
Given endpoints of the latus rectum are:
We get the focus as,
Focus
Focus
Now, we have to find the length of the latus rectum using the endpoints of the latus rectum are:
Distance =
(As stated above, we know that the length of latus rectum is 4a. Therefore, we have,)
and
The value of ‘h’ in the vertex of a parabola has the same value as that of x-coordinate of the focus
That is,
We know that,
The vertex is
and
The vertex is
Therefore, substituting the values of all the variables we obtained we have,
the equation of the parabola opening upwards and having vertex
And
the equation of the parabola opening downwards and having vertex
So, we have the graph as,

Note:
While solving for the focus of the parabola, the focus should not be intermixed with the vertex of the parabola. Both are two different terms. And the equation for parabola opening upwards and downwards differ only by the presence of a negative sign rest everything is the same. Similarly, the equation to find the value of y component of the vertex varies in sign with parabola facing upwards and downwards.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




