
Derive the expression for the angular magnification of a simple microscope.
Answer
491.4k+ views
Hint : When the object is at a distance closer than the distance of the least distinct vision, we can use a microscope to view the object clearly. The angular magnification of a microscope is defined as the ratio of the angle subtended by the image after refraction from the microscope to the angle subtended by the object at an unaided eye/without the apparatus.
Complete step by step answer
The angular magnification is defined as follows:
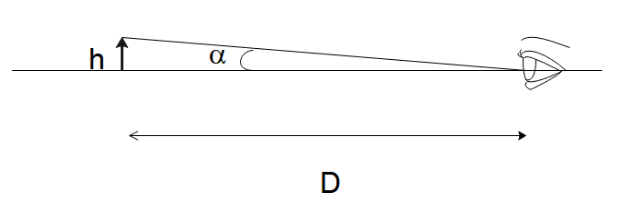
When looking at a small object, as shown above, the angle subtended by the object at the unaided eye is very small and can be approximately written as
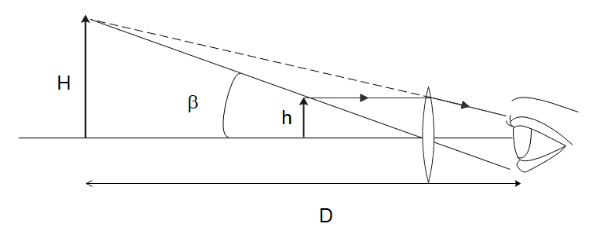
When a lens is placed in between the object and the eye as is done in a simple microscope shown above, the angle subtended by the object will be
Since the image will be formed at the least distance of distinct vision
Now from the lens-maker formula, we can write
We can rearrange the above equation to write,
Since the ratio of the image to the object position is also equal to magnification and we want the image to form at the least distance of distinct vision
This is the magnification power for a simple microscope to form an image at the least distance of distinct vision of the observer.
Note
If the object is placed at infinity, the angle
So,
Complete step by step answer
The angular magnification is defined as follows:

When looking at a small object, as shown above, the angle subtended by the object at the unaided eye is very small and can be approximately written as

When a lens is placed in between the object and the eye as is done in a simple microscope shown above, the angle subtended by the object will be
Since the image will be formed at the least distance of distinct vision
Now from the lens-maker formula, we can write
We can rearrange the above equation to write,
Since the ratio of the image to the object position is also equal to magnification and we want the image to form at the least distance of distinct vision
This is the magnification power for a simple microscope to form an image at the least distance of distinct vision of the observer.
Note
If the object is placed at infinity, the angle
So,
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




