
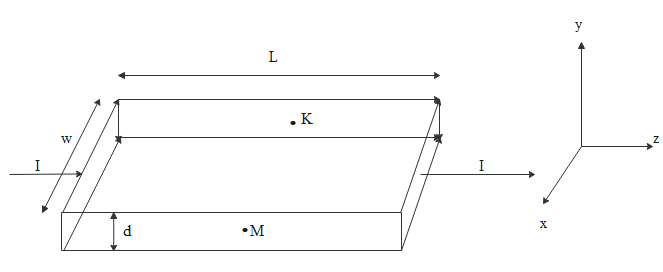
Consider two different metallic strips (1 and 2) of the same material. Their lengths are the same, widths are
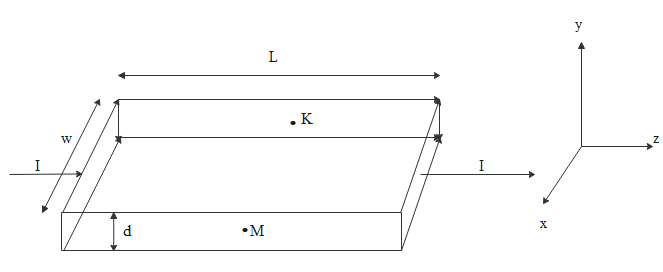
A. If
B. If
C. If
D. If

Answer
493.2k+ views
Hint: If there is a charge, an electric field and magnetic field is acting on that charge then force will be produced due to both electric and magnetic fields. The current passing through the cross section can be expressed in terms of area of cross section. By using the above information we will solve the question.
Formula used:
Complete step by step answer:
It is given that there is a magnetic field B and due to the potential difference generated, an electric field also will be produced. So if we consider the charge ‘q’ it will be acted upon the magnetic force and electric force. we will equate the both forces. We do this because the charge at the slab will be static. Static in the sense the forces should be balanced. Now the current is passing through the cross section. Hence we will apply the formula which will relate current with the drift velocity(v) and area of cross section(A) and charge density(n) and electron charge(e).
Due to the electric field the force acting on the charge ‘q’ will be
Due to the magnetic field the force acting on the charge ‘q’ will be
We have
So the electric force will be
We have
If
So if
Since the potential difference is not dependant on width at all, if the thicknesses are equal then
Hence If
So option A and option D will be correct.
Note: In this question, in case of current flowing we should consider the cross section area perpendicular to the flow of the current. That charges on the slab can’t move anywhere as it is not forming the closed circuit for the charges to flow. Hence we equated the forces so that charges will be at the rest.
Formula used:
Complete step by step answer:
It is given that there is a magnetic field B and due to the potential difference generated, an electric field also will be produced. So if we consider the charge ‘q’ it will be acted upon the magnetic force and electric force. we will equate the both forces. We do this because the charge at the slab will be static. Static in the sense the forces should be balanced. Now the current is passing through the cross section. Hence we will apply the formula which will relate current with the drift velocity(v) and area of cross section(A) and charge density(n) and electron charge(e).

Due to the electric field the force acting on the charge ‘q’ will be
Due to the magnetic field the force acting on the charge ‘q’ will be
We have
So the electric force will be
We have
If
So if
Since the potential difference is not dependant on width at all, if the thicknesses are equal then
Hence If
So option A and option D will be correct.
Note: In this question, in case of current flowing we should consider the cross section area perpendicular to the flow of the current. That charges on the slab can’t move anywhere as it is not forming the closed circuit for the charges to flow. Hence we equated the forces so that charges will be at the rest.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Why is insulin not administered orally to a diabetic class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




